Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(7,\\ a,A=x^2-4x+3+11=\left(x-2\right)^2+10\ge10\\ \text{Dấu }"="\Leftrightarrow x=2\\ b,B=-\left(4x^2-4x+1\right)+6=-\left(2x-1\right)^2+6\le6\\ \text{Dấu }"="\Leftrightarrow x=\dfrac{1}{2}\\ c,x-y=2\Leftrightarrow x=y+2\\ \Leftrightarrow B=y^2-3x^2=y^2-3\left(y+2\right)^2\\ \Leftrightarrow B=y^2-3y^2-12y-12=-4y^2-12y-12\\ \Leftrightarrow B=-\left(4y^2+12y+9\right)-3=-\left(2y+3\right)^2-3\le-3\\ \text{Dấu }"="\Leftrightarrow y=-\dfrac{3}{2}\Leftrightarrow x=\dfrac{1}{2}\)
\(8,\\ \Leftrightarrow x^3-3x^2+5x+a=\left(x-2\right)\cdot a\left(x\right)\)
Thay \(x=2\Leftrightarrow8-12+10+a=0\Leftrightarrow a=-6\)

Bài 7:
a.
$A=(x-1)(x-3)+11=x^2-4x+3+11=x^2-4x+14$
$=(x^2-4x+4)+10=(x-2)^2+10\geq 10$
Vậy gtnn của $A$ là $10$ khi $x=2$
b.
$B=5-4x^2+4x=6-(4x^2-4x+1)=6-(2x-1)^2\leq 6$
Vậy gtln của $B$ là $6$ khi $2x-1=0\Leftrightarrow x=\frac{1}{2}$
c.
$x-y=2\Rightarrow x=y+2$. Khi đó:
$B=y^2-3x^2=y^2-3(y+2)^2=y^2-(3y^2+12y+12)=-2y^2-12y-12$
$=6-2(y^2+6y+9)=6-2(y+3)^2\leq 6$
Vậy $B_{\max}=6$
Bài 8:
Đặt $f(x)=x^3-3x^2+5x+a$
Theo định lý Bê-du, để $f(x)\vdots x-2$ thì $f(2)=0$
$\Leftrightarrow 6+a=0$
$\Leftrightarrow a=-6$

\(a+b\ge a^2+b^2\ge\dfrac{1}{2}\left(a+b\right)^2\Rightarrow a+b\le2\)
\(\Rightarrow2\ge a+b\ge2\sqrt{ab}\Rightarrow ab\le1\)
Xét \(Q=\dfrac{a}{a+1}+\dfrac{b}{b+1}=\dfrac{a\left(b+1\right)+b\left(a+1\right)}{\left(a+1\right)\left(b+1\right)}=\dfrac{a+b+2ab}{\left(a+1\right)\left(b+1\right)}\)
\(Q=\dfrac{a+b+ab+ab}{\left(a+1\right)\left(b+1\right)}\le\dfrac{a+b+ab+1}{\left(a+1\right)\left(b+1\right)}=\dfrac{\left(a+1\right)\left(b+1\right)}{\left(a+1\right)\left(b+1\right)}=1\)
\(\Rightarrow P\le2020+1^{2021}=2021\)
Dấu "=" xảy ra khi \(a=b=1\)

\(B=-\left(4x^2-4x+1\right)+6=-\left(2x-1\right)^2+6\le6\)
Dấu \("="\Leftrightarrow x=\dfrac{1}{2}\)
cho hai số a, b thoả mãn a^2+b^2=1. tìm giá trị lớn nhất và giá trị nhỏ nhất của biểu thức A=a^6+b^6

Ta có
A = a6 + b6 = (a2 + b2)(a4 - a2 b2 + b4)
= a4 - a2 b2 + b4 = (a2 + b2)2 - 3a2b2 = 1 - 3a2 b2 (1)
Ta lại có
1 = a2 + b2 \(\ge\)2ab
\(\Rightarrow ab\le\frac{1}{2}\)(2)
Từ (1) và (2) =>A \(\ge1-\frac{3}{4}=\frac{1}{4}\)
Đạt được khi a2 = b2 = 0,5
Giá trị lớn nhất không có
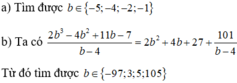
a x b nha