
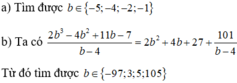
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

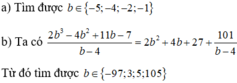

A = \(\frac{x^3-x^2+2}{x-1}=\frac{x^2\left(x-1\right)}{x-1}+\frac{2}{x-1}\)= \(x^2+\frac{2}{x-1}\)
Vì x2 ϵ Z nên để A có giá trị là một số nguyên thì:
2 ⋮ (x - 1) ⇒ (x - 1) ϵ Ư(2)
⇒ Ư(2) = {1; -1; 2; -2}
Ta có bảng sau:
| x - 1 | 1 | -1 | 2 | -2 |
| x | 2 | 0 | 3 | -1 |
Vậy x ϵ {2; 0; 3; -1} thì A có giá trị là một số nguyên.

Câu 1:
a) \(A=\left[\dfrac{2}{3x}-\dfrac{2}{x+1}.\left(\dfrac{x+1}{3x}-x-1\right)\right]:\dfrac{x-1}{x}\)
\(=\left[\dfrac{2}{3x}-\dfrac{2}{3x}+\dfrac{2x}{x+1}+\dfrac{2}{x+1}\right]\dfrac{x}{x-1}\)
\(=\left[\dfrac{2x}{x+1}+\dfrac{2}{x+1}\right]\dfrac{x}{x-1}\)
\(=\dfrac{2x+2}{x+1}.\dfrac{x}{x-1}\)
\(=\dfrac{2\left(x+1\right)}{x+1}.\dfrac{x}{x-1}\)
\(=2.\dfrac{x}{x-1}\)
\(=\dfrac{2x}{x-1}\)
Câu 1:
ĐKXĐ: \(x\notin\left\{0;-1;1\right\}\)
a) Ta có: \(A=\left(\dfrac{2}{3x}-\dfrac{2}{x+1}\cdot\left(\dfrac{x+1}{3x}-x-1\right)\right):\dfrac{x-1}{x}\)
\(=\left(\dfrac{2}{3x}-\dfrac{2}{x+1}\cdot\left(\dfrac{x+1}{3x}-\dfrac{3x\left(x+1\right)}{3x}\right)\right):\dfrac{x-1}{x}\)
\(=\left(\dfrac{2}{3x}-\dfrac{2}{x+1}\cdot\dfrac{x+1-3x^2-3x}{3x}\right):\dfrac{x-1}{x}\)
\(=\left(\dfrac{2}{3x}-\dfrac{2}{x+1}\cdot\dfrac{-3x^2-2x+1}{3x}\right):\dfrac{x-1}{x}\)
\(=\left(\dfrac{2\left(x+1\right)}{3x\left(x+1\right)}-\dfrac{2\cdot\left(-3x^2-2x+1\right)}{3x\left(x+1\right)}\right):\dfrac{x-1}{x}\)
\(=\dfrac{2x+2+6x^2+4x-2}{3x\left(x+1\right)}:\dfrac{x-1}{x}\)
\(=\dfrac{6x^2+6x}{3x\left(x+1\right)}:\dfrac{x-1}{x}\)
\(=\dfrac{6x\left(x+1\right)}{3x\left(x+1\right)}:\dfrac{x-1}{x}\)
\(=2\cdot\dfrac{x}{x-1}=\dfrac{2x}{x-1}\)
b) Để A nguyên thì \(2x⋮x-1\)
\(\Leftrightarrow2x-2+2⋮x-1\)
mà \(2x-2⋮x-1\)
nên \(2⋮x-1\)
\(\Leftrightarrow x-1\inƯ\left(2\right)\)
\(\Leftrightarrow x-1\in\left\{1;-1;2;-2\right\}\)
\(\Leftrightarrow x\in\left\{2;0;3;-1\right\}\)
Kết hợp ĐKXĐ, ta được: \(x\in\left\{2;3\right\}\)
Vậy: Để A nguyên thì \(x\in\left\{2;3\right\}\)

1. Đặt \(t=x^2,t\ge0\)
\(3x^4+4x^2-2\ge3.0+4.0-2=-2\)
=> MIN = -2 khi x = 0
2. \(\left(x^2+2\right)\left(x+1\right)=0\)\(\Leftrightarrow\left[\begin{array}{nghiempt}x^2+2=0\\x+1=0\end{array}\right.\)
Vì \(x^2+2\ge2>0\) => Vô nghiệm
Vậy x+1 = 0 => x = -1
3. Kết quả là 10
4. Ko rõ đề

a: \(\left(a+2\right)^2-\left(a-2\right)^2\)
\(=a^2+4a+4-a^2+4a-4=8a⋮4\)
b: \(\Leftrightarrow n^3-n^2+3n^2-3n+2⋮n-1\)
\(\Leftrightarrow n-1\in\left\{1;-1;2;-2\right\}\)
hay \(n\in\left\{2;0;3;-1\right\}\)

a, \(\dfrac{6}{2x+1}\Rightarrow2x+1\inƯ\left(6\right)=\left\{\pm1;\pm2;\pm3;\pm6\right\}\)
| 2x + 1 | 1 | -1 | 2 | -2 | 3 | -3 | 6 | -6 |
| 2x | 0 | -2 | 1 | -3 | 2 | -4 | 5 | -7 |
| x | 0 | -1 | 1/2 ( loại ) | -3/2 ( loại ) | 1 | -2 | 5/2 ( loại ) | -7/2 ( loại ) |
c, \(\dfrac{x-3}{x-1}=\dfrac{x-1-2}{x-1}=1-\dfrac{2}{x-1}\Rightarrow x-1\inƯ\left(2\right)=\left\{\pm1;\pm2\right\}\)
| x - 1 | 1 | -1 | 2 | -2 |
| x | 2 | 0 | 3 | -1 |
tương tự ....

a) Ta có M = ( b + 2 ) ( 3 b − 10 ) + 5 b + 2 = 3 b − 10 + 5 b + 2 . Ta có, với b nguyên thì M nhận giá trị nguyên khi và chỉ khi b + 2 nhận giá trị ước của 5. Đáp số: b ∈ − 7 ; − 3 ; − 1 ; 3
b) Tương tự, ta có b ∈ − 3 ; 0 ; 1 ; 2 ; 4 ; 5 ; 6 ; 9