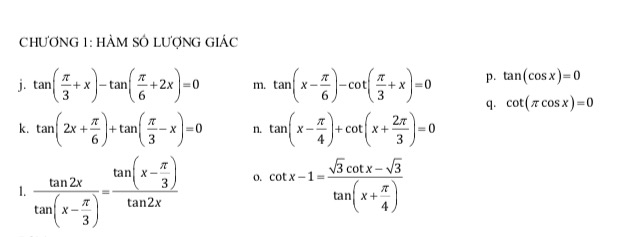DN
0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NL
2
NV
Nguyễn Việt Lâm
Giáo viên
1 tháng 11 2021
\(y=4\left(1-sin^2x\right)+2sinx+2=-4sin^2x+2sinx+6\)
Đặt \(sinx=t\in\left[-1;1\right]\Rightarrow y=f\left(t\right)=-4t^2+2t+6\)
\(-\dfrac{b}{2a}=\dfrac{1}{4}\in\left[-1;1\right]\)
\(f\left(-1\right)=0\) ; \(f\left(\dfrac{1}{4}\right)=\dfrac{25}{4}\); \(f\left(1\right)=4\)
\(\Rightarrow y_{max}=\dfrac{25}{4}\) khi \(sinx=\dfrac{1}{4}\)
\(y_{min}=0\) khi \(sinx=-1\)
1 tháng 11 2021
Ta có: \(y=4cos^2x+2sinx+2=4-4sin^2x+2sinx+2=-4sin^2x+2sinx+6=-\left(4sin^2x-2sinx+\dfrac{1}{16}-\dfrac{1}{16}-6\right)=-\left(2sin^2x-\dfrac{1}{4}\right)^2+\dfrac{97}{16}\)
Ta có: \(-\left(2sin^2x-\dfrac{1}{4}\right)^2\le0\Rightarrow y\le\dfrac{97}{16}\)
Vậy \(y_{max}=\dfrac{97}{16}\)
RT
1
2 tháng 7 2021
Pt \(\Leftrightarrow\left(2sinx-1\right)\left(2sin2x-1\right)=3-4\left(1-sin^2x\right)\)
\(\Leftrightarrow2sin2x\left(2sinx-1\right)-2sinx+1=-1+4sin^2x\)
\(\Leftrightarrow2sin2x\left(2sinx-1\right)-\left(4sin^2x+2sinx-2\right)=0\)
\(\Leftrightarrow2sin2x\left(2sinx-1\right)-2\left(2sinx-1\right)\left(sinx+1\right)=0\)
\(\Leftrightarrow2\left(2sinx-1\right)\left(sin2x-sinx-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=\dfrac{1}{2}\left(1\right)\\sin2x=sinx+1\left(2\right)\end{matrix}\right.\)
Từ (1) \(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{\pi}{6}+k2\pi\\x=\dfrac{5\pi}{6}+k2\pi\end{matrix}\right.\),\(k\in Z\)
Từ (2)\(\Leftrightarrow2sinx.cosx-sinx-1=0\)
(Cái này tạm thời nghĩ ko ra,tối làm :)
2 tháng 7 2021
\(sin2x=sinx+1\)
\(\Rightarrow\left\{{}\begin{matrix}sin2x\ge0\\sin^22x=\left(sinx+1\right)^2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}sin2x\ge0\\4sin^2x.cos^2x=\left(sinx+1\right)^2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}sin2x\ge0\\4sin^2x\left(1-sin^2x\right)=\left(sinx+1\right)^2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}sin2x\ge0\\\left(sinx+1\right)\left(4sin^2x-4sin^3x-sinx-1\right)=0\end{matrix}\right.\)
Bấm máy thấy pt \(-4sin^3x+4sin^2x-sinx-1=0\) có một nghiệm \(sinx< 0\) không thỏa mãn \(sin2x\ge0\)
(Hoặc thử sd phương pháp cardano xem, chắc sẽ tìm được cụ thể nghiệm)
\(\Rightarrow sinx=-1\Leftrightarrow x=-\dfrac{\pi}{2}+k2\pi\) (\(k\in Z\))
Vậy...
NA
Giải pt:
\(\left(2sinx-1\right)^2-\left(2sinx-1\right)\left(sinx-\frac{3}{2}\right)=0\)
Giúp với ạ !
1
NV
Nguyễn Việt Lâm
Giáo viên
20 tháng 9 2020
\(\Leftrightarrow\left(2sinx-1\right)\left(2sinx-1-sinx+\frac{3}{2}\right)=0\)
\(\Leftrightarrow\left(2sinx-1\right)\left(sinx+\frac{1}{2}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sinx=\frac{1}{2}\\sinx=-\frac{1}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\frac{\pi}{6}+k2\pi\\x=\frac{5\pi}{6}+k2\pi\\x=-\frac{\pi}{6}+k2\pi\\x=\frac{7\pi}{6}+k2\pi\end{matrix}\right.\)
17 tháng 8 2023
1: cot x=-6 nên cosx/sinx=-6
=>cosx=-6*sinx
\(F=\dfrac{sinx-3\cdot cosx}{cosx+2\cdot sinx}=\dfrac{sinx+18\cdot sinx}{-6\cdot sinx+2\cdot sinx}=\dfrac{20}{-4}=-5\)
2: cotx=1
=>cosx/sinx=1
=>cosx=sinx
\(I=\dfrac{sin^3x-4\cdot sin^3x}{sinx+3sinx}=\dfrac{5\cdot sin^3x}{4\cdot sinx}=\dfrac{5}{4}\cdot sin^2x\)
\(1+cot^2x=\dfrac{1}{sin^2x}\)
=>\(\dfrac{1}{sin^2x}=1+1=2\)
=>sin^2=1/2
=>\(I=\dfrac{5}{4}\cdot\dfrac{1}{2}=\dfrac{5}{8}\)
3: cotx=3
=>cosx/sinx=3
=>cosx=3*sinx
1+cot^2x=1/sin^2x
=>\(\dfrac{1}{sin^2x}=1+9=10\)
=>\(sin^2x=\dfrac{1}{10}\)
\(I=\dfrac{2\cdot sin^3x+cos^3x}{4\cdot sinx-6\cdot cosx}\)
\(=\dfrac{2\cdot sin^3x+\left(3\cdot sinx\right)^3}{4\cdot sinx-6\cdot\left(3\cdot sinx\right)}=\dfrac{2\cdot sin^3x+27\cdot sin^3x}{4\cdot sinx-18\cdot sinx}\)
\(=\dfrac{29}{-14}\cdot sin^2x=\dfrac{-29}{14}\cdot\dfrac{1}{10}=-\dfrac{29}{140}\)
TB
1
HP
23 tháng 9 2021
j, ĐK: \(x\ne\dfrac{\pi}{6}+\dfrac{k\pi}{2}\)
\(tan\left(\dfrac{\pi}{3}+x\right)-tan\left(\dfrac{\pi}{6}+2x\right)=0\)
\(\Leftrightarrow tan\left(\dfrac{\pi}{3}+x\right)=tan\left(\dfrac{\pi}{6}+2x\right)\)
\(\Leftrightarrow\dfrac{\pi}{3}+x=\dfrac{\pi}{6}+2x+k\pi\)
\(\Leftrightarrow x=\dfrac{\pi}{6}+k\pi\left(l\right)\)
\(\Rightarrow\) vô nghiệm.
TV
0
AH
Akai Haruma
Giáo viên
22 tháng 12 2020
Lời giải:
\(y=-4\cos ^2x+2\sin x+3=-4(1-\sin ^2x)+2\sin x+3=4\sin ^2x+2\sin x-1\)
Đặt \(\sin x=t(t\in [-1;1])\) thì:
\(y=4t^2+2t-1\)
\(y'=8t+2=0\Leftrightarrow t=-\frac{1}{4}\)
Lập BBT. Với các giá trị \(y(\frac{-1}{4})=\frac{-5}{4}; y(-1)=1; y(1)=5\) ta thấy:
\(y_{\max}=5\Leftrightarrow t=1\Leftrightarrow x=2k\pi +\frac{\pi}{2}\)
\(y_{\min}=\frac{-5}{4}\Leftrightarrow t=\frac{-1}{4}\Leftrightarrow x=2k\pi -2\tan ^{-1}(4\pm \sqrt{15})\)