Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(x>1\)
\(f'\left(x\right)=\left(2x+2\right)\sqrt{x-1}+\frac{x^2+2x}{2\sqrt{x-1}}=\frac{5x^2+2x-4}{2\sqrt{x-1}}\)
\(f'\left(x\right)\ge0\Leftrightarrow\frac{5x^2+2x-4}{\sqrt{x-1}}\ge0\Leftrightarrow5x^2+2x-4\ge0\)
\(\Rightarrow x>1\)

1.
\(y'=12x+\dfrac{4}{x^2}\)
2.
\(y'=\dfrac{3}{\left(-x+1\right)^2}\)
3.
\(y'=\dfrac{2x-3}{2\sqrt{x^2-3x+4}}\)
4.
\(y=\dfrac{x^3+3x^2-x-3}{x-4}\)
\(y'=\dfrac{\left(3x^2+6x-1\right)\left(x-4\right)-\left(x^3+3x^2-x-3\right)}{\left(x-4\right)^2}=\dfrac{2x^3-9x^2-24x+7}{\left(x-4\right)^2}\)
5.
\(y'=-\dfrac{4x-3}{\left(2x^2-3x+5\right)^2}\)
6.
\(y'=\sqrt{x^2-1}+\dfrac{x\left(x+1\right)}{\sqrt{x^2-1}}\)

\(\lim\limits_{x\rightarrow1^-}f\left(x\right)=\lim\limits_{x\rightarrow1^-}\frac{\sqrt[3]{5x+3}-2+2-\sqrt{2x+2}}{x-1}=\lim\limits_{x\rightarrow1^-}\frac{\frac{5\left(x-1\right)}{\sqrt[3]{\left(5x+3\right)^2}+2\sqrt[3]{5x+3}+4}-\frac{2\left(x-1\right)}{2+\sqrt{2x+2}}}{x-1}\)
\(=\lim\limits_{x\rightarrow1^-}\left(\frac{5}{\sqrt[3]{\left(5x+3\right)^2}+2\sqrt[3]{5x+3}+4}-\frac{2}{2+\sqrt{2x+2}}\right)=-\frac{1}{12}\)
\(\lim\limits_{x\rightarrow1^+}=\lim\limits_{x\rightarrow1^+}m.sin\left(\frac{\pi x}{2}+2019\right)=\)
Đến đây lại thêm vấn đề nữa, \(sin\left(\frac{\pi x}{2}+2019\right)\) hay \(sin\left(\frac{\pi x}{2}+2019\pi\right)\) bạn?
Bạn ghi đề sai thì phải, nhìn hàm khi \(x< 1\) thì \(\lim\limits_{x\rightarrow1^-}f\left(x\right)\) không tồn tại (ko phải dạng vô định \(\frac{0}{0}\), khi thay x=1 vào tử số ra khác 0)

Chọn B.
Ta có: y = (2x – 1)(3x + 2) = (2x2 – x)(3x + 2)
y’ = [(2x2 – x)(3x + 2)]’ = (2x2 – x)’(3x + 2) + (3x + 2)’.(2x2 – x)
= (4x – 1)(3x + 2) + 3(2x2 – x) = 18x2 + 2x – 2.

\(f^2\left(1+2x\right)=x-f^3\left(1-2x\right)\)
Thay \(x=0\) vào ta được:
\(f^2\left(1\right)=-f^3\left(1\right)\Leftrightarrow f^2\left(1\right)\left[1+f\left(1\right)\right]=0\Rightarrow\left[{}\begin{matrix}f\left(1\right)=0\\f\left(1\right)=-1\end{matrix}\right.\)
Đạo hàm 2 vế:
\(\Rightarrow4f\left(1+2x\right).f'\left(1+2x\right)=1+6f^2\left(1-2x\right).f'\left(1-2x\right)\)
Thay \(x=0\) vào ta được:
\(4f\left(1\right).f'\left(1\right)=1+6f^2\left(1\right).f'\left(1\right)\)
- Nếu \(f\left(1\right)=0\Rightarrow0=1\) (loại)
- Nếu \(f\left(1\right)=-1\Rightarrow-4f'\left(1\right)=1+6f'\left(1\right)\Rightarrow f'\left(1\right)=-\frac{1}{10}\)

Câu 1:
Đặt \(f\left(x\right)=x^3+mx^2+\left(m-3\right)x-1\)
Ta có \(f\left(0\right)=-1\) ; \(f\left(-1\right)=1\)
\(\Rightarrow f\left(0\right).f\left(-1\right)< 0\Rightarrow f\left(x\right)\) có ít nhất 1 nghiệm thuộc \(\left(-1;0\right)\)
Mặt khác \(\left\{{}\begin{matrix}f\left(0\right)=-1< 0\\\lim\limits_{x\rightarrow+\infty}=+\infty\end{matrix}\right.\) \(\Rightarrow f\left(x\right)\) có ít nhất 1 nghiệm thuộc \(\left(0;+\infty\right)\)
\(\left\{{}\begin{matrix}f\left(-1\right)=1>0\\\lim\limits_{x\rightarrow-\infty}=-\infty\end{matrix}\right.\) \(\Rightarrow f\left(x\right)\) có ít nhất 1 nghiệm thuộc \(\left(-\infty;-1\right)\)
Vậy pt đã cho có 3 nghiệm phân biệt với mọi m
Câu 2:
\(f'\left(x\right)=x^2+2\left(m-1\right)x+m+1\)
Để \(f'\left(x\right)\ge0\) \(\forall x\) \(\Leftrightarrow\Delta'=\left(m-1\right)^2-\left(m+1\right)\le0\)
\(\Leftrightarrow m^2-3m\le0\Leftrightarrow0\le m\le3\)
Câu 3:
Nhận thấy \(x=0\) không phải nghiệm
\(\Leftrightarrow2x^3+3x^2-2=-mx\)
\(\Leftrightarrow\frac{2x^3+3x^2-2}{x}=-m\)
Đặt \(f\left(x\right)=\frac{2x^3+3x^2-2}{x}\Rightarrow f'\left(x\right)=\frac{\left(6x^2+6x\right)x-\left(2x^3+3x^2-2\right)}{x^2}=\frac{4x^3+3x^2+2}{x^2}\)
\(f'\left(x\right)=\frac{4x^2\left(x+1\right)+2-x^2}{x^2}\Rightarrow f'\left(x\right)>0\) \(\forall x\in\left(-1;1\right)\)
\(\Rightarrow f\left(x\right)\) đồng biến trên \(\left(-1;1\right)\)
\(\lim\limits_{x\rightarrow0^-}f\left(x\right)=+\infty\) ; \(\lim\limits_{x\rightarrow0^+}f\left(x\right)=-\infty\)
\(\Rightarrow y=-m\) luôn cắt đồ thị \(y=f\left(x\right)\) hay phương trình đã cho luôn có ít nhất 1 nghiệm trong khoảng \(\left(-1;1\right)\) với mọi m
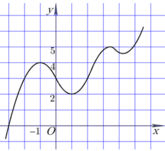
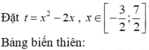
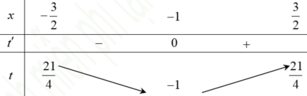




Ta có:f'(x)=4x-1
=>f'(x)\(\sqrt{x^2+1}=2x^2+2x+1\)
<=>(4x-1)\(\sqrt{x^2+1}=2x^2+2x+1\)
Nhận xét: vế phải > 0 nên đk để phương trình có nghiệm:x>\(\dfrac{1}{4}\)
Từ điều kiện trên phương trình
<=>(16x2-8x+1)(x2+1)=4x4+8x3+8x2+4x+1
<=>16x4+16x2-8x3-8x+x2+1=4x4+8x3+8x2+4x+1
<=>12x4-16x3+9x2-12x=0
<=>x(12x3-16x2+9x-12)=0
<=>x(3x-4)(4x2+3)=0
<=>x=0 hoặc x=\(\dfrac{4}{3}\)(do 4x2+3>0)
Vậy...
x=0 loại