Tìm GTLN của :-|2,68-2x|-5,9
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
12 tháng 12
-|2,68 - 2\(x\)| - 5,9
Vì |2,68 - 2\(x\)| ≥ 0 ⇒ -|2,68 - 2\(x\)| ≤ 0 ⇒ - |2,68 - 2\(x\)| - 5,9 ≤ -5,9
Dấu bằng xảy ra khi:
2,68 - 2\(x\) = 0 ⇒ 2\(x\) = 2,68 ⇒ \(x\) = 2,68 : 2 ⇒ \(x=1,34\)
Vậy giá trị lớn nhất của biểu thức:
- |2,68 - 2\(x\)| - 5,9 là -5,9 xảy ra khi \(x=1,34\)
TN
1
11 tháng 10 2019
Ta có: \(\left|2,68-2x\right|\ge0\)
\(\Rightarrow-\left|2,68-2x\right|\le0\)
\(\Rightarrow-\left|2,68-2x\right|-5,9\le0-5,9\)
\(\Rightarrow B\le-5,9\)
GTLN của B là -5,9
Dấu "=" xảy ra khi: \(2,68-2x=-5,9\)
\(\Rightarrow2x=2,68-\left(-5,9\right)\)
\(\Rightarrow2x=8,58\)
\(\Rightarrow x=4,29\)
25 tháng 10 2020
Tìm GTLN?
Ta có:
\(A=-\left|2,68-2x\right|-5,9\)
Mà \(-\left|2,68-2x\right|\le0\left(\forall x\right)\)
\(\Rightarrow A=-\left|2,68-2x\right|-5,9\le-5,9\left(\forall x\right)\)
Dấu "=" xảy ra khi: \(-\left|2,68-2x\right|=0\)
\(\Rightarrow x=1,34\)
Vậy Max(A) = -5,9 khi x = 1,34
13 tháng 11 2021
\(A=\left(x^2-2x+1\right)+4=\left(x-1\right)^2+4\ge4\\ A_{min}=4\Leftrightarrow x=1\\ B=2\left(x^2-3x\right)=2\left(x^2-2\cdot\dfrac{3}{2}x+\dfrac{9}{4}\right)-\dfrac{9}{2}\\ B=2\left(x-\dfrac{3}{2}\right)^2-\dfrac{9}{2}\ge-\dfrac{9}{2}\\ B_{min}=-\dfrac{9}{2}\Leftrightarrow x=\dfrac{3}{2}\\ C=-\left(x^2-4x+4\right)+7=-\left(x-2\right)^2+7\le7\\ C_{max}=7\Leftrightarrow x=2\)
13 tháng 11 2021
a,\(A=x^2-2x+5=\left(x^2-2x+1\right)+4=\left(x-1\right)^2+4\ge4\)
Dấu "=" \(\Leftrightarrow x=-1\)
b,\(B=2\left(x^2-3x\right)=2\left(x^2-3x+\dfrac{9}{4}\right)-\dfrac{9}{2}=2\left(x-\dfrac{3}{2}\right)^2-\dfrac{9}{2}\ge-\dfrac{9}{2}\)
Dấu "=" \(\Leftrightarrow x=\dfrac{3}{2}\)
c,\(=C=-\left(x^2-4x-3\right)=-\left[\left(x^2-4x+4\right)-7\right]=-\left(x-2\right)^2+7\le7\)
Dấu "=" \(\Leftrightarrow x=2\)
AH
Akai Haruma
Giáo viên
3 tháng 2
Câu 1:
$y=-2x^2+4x+3=5-2(x^2-2x+1)=5-2(x-1)^2$
Vì $(x-1)^2\geq 0$ với mọi $x\in\mathbb{R}$ nên $y=5-2(x-1)^2\leq 5$
Vậy $y_{\max}=5$ khi $x=1$
Hàm số không có min.
AH
Akai Haruma
Giáo viên
3 tháng 2
Câu 2:
Hàm số $y$ có $a=-3<0; b=2, c=1$ nên đths có trục đối xứng $x=\frac{-b}{2a}=\frac{1}{3}$
Lập BTT ta thấy hàm số đồng biến trên $(-\infty; \frac{1}{3})$ và nghịch biến trên $(\frac{1}{3}; +\infty)$
Với $x\in (1;3)$ thì hàm luôn nghịch biến
$\Rightarrow f(3)< y< f(1)$ với mọi $x\in (1;3)$
$\Rightarrow$ hàm không có min, max.
PP
1
HP
2 tháng 8 2021
Đặt \(sin^24x=t\left(t\in\left[0;1\right]\right)\)
\(y=1-8sin^22x.cos^22x+2sin^42x\)
\(=1-2sin^24x+2sin^42x\)
\(\Rightarrow y=f\left(t\right)=1-2t+2t^2\)
\(y_{min}=min\left\{f\left(0\right);f\left(1\right);f\left(\dfrac{1}{2}\right)\right\}=\dfrac{1}{2}\)
\(y_{max}=max\left\{f\left(0\right);f\left(1\right);f\left(\dfrac{1}{2}\right)\right\}=1\)
YN
23 tháng 2 2020
B =2012-| 3x + 3 | - ||x+3| + 2x|
Ta có \(\hept{\begin{cases}\left|3x+3\right|\ge0\\\left|\left|x+3\right|+2x\right|\ge0\end{cases}\forall x}\)
\(\Leftrightarrow\left|3x+3\right|+\left|\left|x+3\right|+2x\right|\ge0\forall x\)
\(\Leftrightarrow-\left|3x+3\right|-\left|\left|x+3\right|+2x\right|\le0\forall x\)
\(\Leftrightarrow2012-\left|3x+3\right|-\left|\left|x+3\right|+2x\right|\le2012\forall x\)
\(\Leftrightarrow B\le2012\forall x\).
Dấu "=" xảy ra <=> \(\hept{\begin{cases}\left|3x+3\right|=0\\\left|\left|x+3\right|+2x\right|=0\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}3x+3=0\\\left|x+3\right|+2x=0\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}3x=-3\\\left|x+3\right|=-2x\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x=-1\\\left|-1+3\right|=-2.\left(-1\right)\end{cases}}\)
\(\Leftrightarrow\hept{\begin{cases}x=-1\\2=2\end{cases}}\)
<=> x = 1
Vậy Max B = 2012 <=> x = 1
y ở đâu v bạn ~~?????
@@ Học tốt
Chiyuki Fujito
F
23 tháng 2 2020
Bài giải
Ta có : \(B=2012-\left|3x+3\right|-||x+3|+2x|=2012-\text{( }\left|3x+3\right|+||x+3|+2x|\text{ ) }\)
B đạt GTLN khi \(\text{( }\left|3x+3\right|+||x+3|+2x|\text{ ) }\)đạt GTNN
Đặt \(C=\text{( }\left|3x+3\right|+||x+3|+2x|\text{ ) }\ge|3x+3+\text{ | }x+3\text{ |}+2x|\text{ }=\left|5x+3\text{ + | }x+3\text{ | }\right|\)
Dấu " = " xảy ra khi \(\hept{\begin{cases}x\ge-1\text{ hoặc }x\le-1\\x=-1\end{cases}}\)
Vậy Min C = 0 khi x = - 1
Vậy Max B = 2012 khi x = - 1
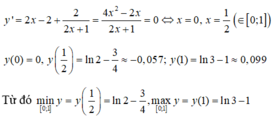
-|2,68 - 2\(x\)| - 5,9
Vì |2,68 - 2\(x\)| ≥ 0 ⇒ -|2,68 - 2\(x\)| ≤ 0 ⇒ - |2,68 - 2\(x\)| - 5,9 ≤ -5,9
Dấu bằng xảy ra khi:
2,68 - 2\(x\) = 0 ⇒ 2\(x\) = 2,68 ⇒ \(x\) = 2,68 : 2 ⇒ \(x=1,34\)
Vậy giá trị lớn nhất của biểu thức:
- |2,68 - 2\(x\)| - 5,9 là -5,9 xảy ra khi \(x=1,34\)