NS
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
15 tháng 11 2021
\(a,=x^2+x+4x+4=\left(x+1\right)\left(x+4\right)\\ b,=x^2+2x-3x-6=\left(x-3\right)\left(x+2\right)\\ c,=x^2-2x-3x+6=\left(x-2\right)\left(x-3\right)\\ d,=3\left(x^2-2x+5x-10\right)=3\left(x-2\right)\left(x+5\right)\\ e,=-3x^2+6x-x+2=\left(x-2\right)\left(1-3x\right)\\ f,=x^2-x-6x+6=\left(x-1\right)\left(x-6\right)\\ h,=4\left(x^2-3x-6x+18\right)=4\left(x-3\right)\left(x-6\right)\\ i,=3\left(3x^2-3x-8x+5\right)=3\left(x-1\right)\left(3x-8\right)\\ k,=-\left(2x^2+x+4x+2\right)=-\left(2x+1\right)\left(x+2\right)\\ l,=x^2-2xy-5xy+10y^2=\left(x-2y\right)\left(x-5y\right)\\ m,=x^2-xy-2xy+2y^2=\left(x-y\right)\left(x-2y\right)\\ n,=x^2+xy-3xy-3y^2=\left(x+y\right)\left(x-3y\right)\)
NT
0
TQ
2
2 tháng 10 2023
\(\left(x-2y\right)\left(x^2+2xy+4y^2\right)-\left(x-y\right)\left(x^2+8y^2\right)\)
\(=x^3-8y^3-\left(x^3-x^2y+8xy^2-8y^3\right)\)
\(=x^3-8y^3-x^3+x^2y-8xy^2+8y^3\)
\(=x^2y-8xy^2\)
MA
0
A
10 tháng 4 2019
https://tailieu.vn/doc/cac-dang-bai-tap-dai-so-lop-9-va-cac-luu-y-khi-giai-phan-1-789511.html
https://tailieu.vn/doc/cac-dang-bai-tap-dai-so-lop-9-va-cac-luu-y-khi-giai-phan-2-789512.html
https://tailieu.vn/doc/tuyen-tap-bai-tap-toan-hinh-hoc-lop-9-310559.html
https://tailieu.vn/doc/80-bai-tap-hinh-hoc-lop-9-co-dap-an-872980.html
16 tháng 2 2023
\(\dfrac{x}{a}=\dfrac{m-\dfrac{x}{2}}{m}\)
\(\Rightarrow xm=a\left(m-\dfrac{x}{2}\right)\)
\(\Rightarrow xm=am-\dfrac{ax}{2}\)
\(\Rightarrow2xm=2am-ax\)
\(\Rightarrow2xm+ax=2am\)
\(\Rightarrow x\left(2m+a\right)=2am\)
\(\Rightarrow x=\dfrac{2am}{a+2m}\)
mn ơi ai thấy bài này giúp em được ko ạ,e đang cần gấp lắm
30 tháng 10 2021
a, Vì D,M là trung điểm AB,AC nên DM là đtb tg ABC
Do đó \(DM=\dfrac{1}{2}BC=\dfrac{7}{2}\left(cm\right)\) và DM//BC
21 tháng 8 2021
a) \(\left(x+2\right)^3-x^2.\left(x+6\right)\)
\(=x^3+6x^2+12x+8-x^3-6x^2\)
\(=12x+8\)
b) \(\left(x-2\right)\left(x+2\right)-\left(x+1\right)^3-2x.\left(x-1\right)^2\)
\(=x^2-4-x^3-3x^2-3x-1-2x^3+4x^2-2x\)
\(=-3x^3+2x^2-5x-5\)
CG
4
3 tháng 5 2017
(Toán học) Trị số khi thay vào ẩn số của một phương trình thì làm cho phương trình được thỏa mãn. Tập nghiệm của phương trình x + 2 = 4 là 2. Trị số khi thay vào biến x thì làm cho đa thức bằng 0.
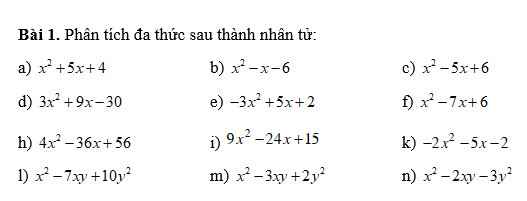
pt⇔y2(x2−7)=(x+y)2(1)
Phương trình đã cho có nghiệm x=y=0x=y=0
Xét x,y\ne0x,y≠0, từ (1)(1) suy ra x^2-7x2−7 là một số chính phương
Đặt x^2-7=a^2x2−7=a2 ta có:
\left(x-a\right)\left(x+a\right)=7(x−a)(x+a)=7 từ đây tìm được x
Vậy (x,y)=(0,0);(4,-1);(4,2);(-4,1);(-4;-2)(x,y)=(0,0);(4,−1);(4,2);(−4,1);(−4;−2)