
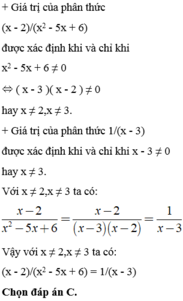
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

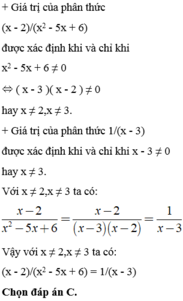

Bài 2:
(1 + x)3 + (1 - x)3 - 6x(x + 1) = 6
<=> x3 + 3x2 + 3x + 1 - x3 + 3x2 - 3x + 1 - 6x2 - 6x = 6
<=> -6x + 2 = 6
<=> -6x = 6 - 2
<=> -6x = 4
<=> x = -4/6 = -2/3
Bài 3:
a) (7x - 2x)(2x - 1)(x + 3) = 0
<=> 10x3 + 25x2 - 15x = 0
<=> 5x(2x - 1)(x + 3) = 0
<=> 5x = 0 hoặc 2x - 1 = 0 hoặc x + 3 = 0
<=> x = 0 hoặc x = 1/2 hoặc x = -3
b) (4x - 1)(x - 3) - (x - 3)(5x + 2) = 0
<=> 4x2 - 13x + 3 - 5x2 + 13x + 6 = 0
<=> -x2 + 9 = 0
<=> -x2 = -9
<=> x2 = 9
<=> x = +-3
c) (x + 4)(5x + 9) - x2 + 16 = 0
<=> 5x2 + 9x + 20x + 36 - x2 + 16 = 0
<=> 4x2 + 29x + 52 = 0
<=> 4x2 + 13x + 16x + 52 = 0
<=> 4x(x + 4) + 13(x + 4) = 0
<=> (4x + 13)(x + 4) = 0
<=> 4x + 13 = 0 hoặc x + 4 = 0
<=> x = -13/4 hoặc x = -4

bài1 A=\(\left(\frac{3-x}{x+3}\cdot\frac{x^2+6x+9}{x^2-9}+\frac{x}{x+3}\right):\frac{3x^2}{x+3}\)
=\(\left(-\frac{x-3\cdot\left(x+3\right)^2}{\left(x+3\right)^2\cdot\left(x-3\right)}+\frac{x}{x+3}\right):\frac{3x^2}{x+3}\)
=\(-\frac{x}{x+3}\cdot\frac{x+3}{3x^2}=\frac{-1}{3x}\)
b) thế \(x=-\frac{1}{2}\)vào biểu thức A
\(-\frac{1}{3\cdot\left(-\frac{1}{2}\right)}=\frac{2}{3}\)
c) A=\(-\frac{1}{3x}< 0\)
VÌ (-1) <0 nên 3x>0
x >0

1)
a) Biểu thức \(\dfrac{x-2}{x^2+8x}\) vô nghĩa khi \(x^2+8x=0\)
\(\Leftrightarrow x\left(x+8\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x+8=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-8\end{matrix}\right.\)
Vậy: Khi \(x\in\left\{0;-8\right\}\) thì biểu thức \(\dfrac{x-2}{x^2+8x}\) vô nghĩa
b) Biểu thức \(\dfrac{25x^2-1}{16x^2-25}\) vô nghĩa khi \(16x^2-25=0\)
\(\Leftrightarrow\left(4x-5\right)\left(4x+5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}4x-5=0\\4x+5=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}4x=5\\4x=-5\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{5}{4}\\x=-\dfrac{5}{4}\end{matrix}\right.\)
Vậy: Khi \(x\in\left\{\dfrac{5}{4};-\dfrac{5}{4}\right\}\) thì biểu thức \(\dfrac{25x^2-1}{16x^2-25}\) vô nghĩa
c) Biểu thức \(\dfrac{x^2+1}{2x^2-28x+98}\) vô nghĩa khi \(2x^2-28x+98=0\)
\(\Leftrightarrow2\left(x^2-14x+49\right)=0\)
\(\Leftrightarrow\left(x-7\right)^2=0\)
\(\Leftrightarrow x-7=0\)
hay x=7
Vậy: Khi x=7 thì biểu thức \(\dfrac{x^2+1}{2x^2-28x+98}\) vô nghĩa
d) Để biểu thức \(\dfrac{2x+3}{9-\left(x+3\right)^2}\) vô nghĩa thì \(9-\left(x+3\right)^2=0\)
\(\Leftrightarrow\left(3-x-3\right)\left(3+x+3\right)=0\)
\(\Leftrightarrow-x\left(x+6\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}-x=0\\x+6=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-6\end{matrix}\right.\)
Vậy: Khi \(x\in\left\{0;-6\right\}\) thì biểu thức \(\dfrac{2x+3}{9-\left(x+3\right)^2}\) vô nghĩa
2)
a) ĐKXĐ: \(x\notin\left\{0;-8\right\}\)
b) ĐKXĐ: \(x\notin\left\{\dfrac{5}{4};-\dfrac{5}{4}\right\}\)
c) ĐKXĐ: \(x\ne7\)
d) ĐKXĐ: \(x\notin\left\{0;-6\right\}\)
3)
a) Để phân thức \(\dfrac{x-2}{x^2+8x}=0\) thì x-2=0
hay x=2(nhận)
Vậy: Khi x=2 thì phân thức \(\dfrac{x-2}{x^2+8x}=0\)
b) Để phân thức \(\dfrac{25x^2-1}{16x^2-25}=0\) thì \(25x^2-1=0\)
\(\Leftrightarrow\left(5x-1\right)\left(5x+1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}5x-1=0\\5x+1=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}5x=1\\5x=-1\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{5}\left(nhận\right)\\x=-\dfrac{1}{5}\left(nhận\right)\end{matrix}\right.\)
Vậy: Khi \(x\in\left\{\dfrac{1}{5};-\dfrac{1}{5}\right\}\) thì phân thức \(\dfrac{25x^2-1}{16x^2-25}=0\)
c) Để phân thức \(\dfrac{x^2+1}{2x^2-28x+98}=0\) thì \(x^2+1=0\)
mà \(x^2+1>0\forall x\) thỏa mãn ĐKXĐ
nên \(x\in\varnothing\)
Vậy: Không có giá trị nào của x để \(\dfrac{x^2+1}{2x^2-28x+98}=0\)
d) Để phân thức \(\dfrac{2x+3}{9-\left(x+3\right)^2}=0\) thì 2x+3=0
\(\Leftrightarrow2x=-3\)
hay \(x=-\dfrac{3}{2}\)(nhận)
Vậy: Khi \(x=-\dfrac{3}{2}\) thì phân thức \(\dfrac{2x+3}{9-\left(x+3\right)^2}=0\)
mình chỉ làm 1 câu thôi nhé các câu khác làm tương tự
1. biểu thức vô nghĩa <=> mẫu thức = 0
\(x^2+8x=0< =>\left[{}\begin{matrix}x=0\\x=-8\end{matrix}\right.\)
vậy ...
2. tập xác định là tập hợp các giá trị làm phân thức có nghĩa (trong căn thì ≥ 0 ; dưới mẫu thì ≠ 0)
\(x^2+8x\ne0< =>\left[{}\begin{matrix}x\ne0\\x\ne-8\end{matrix}\right.\)
vậy ...
3. để phân thức = 0 => tử bằng không và mẫu khác không
\(\left\{{}\begin{matrix}x-2=0\\x^2+8x\ne0\end{matrix}\right.< =>\left\{{}\begin{matrix}x=2\left(tm\right)\\\left[{}\begin{matrix}x\ne0\\x\ne-8\end{matrix}\right.\end{matrix}\right.\)

a, \(E=\left(\frac{x^2+4}{x^2-4}+\frac{6}{6-3x}+\frac{1}{x+2}\right):\left(x-2+\frac{10-x^2}{x+2}\right)\)ĐK : \(x\ne\pm2\)
\(=\left(\frac{x^2+4}{x^2-4}+\frac{2}{2-x}+\frac{1}{x+2}\right):\left(\frac{x^2-4+10-x^2}{x+2}\right)\)
\(=\left(\frac{x^2+4-2\left(x+2\right)+x-2}{\left(x-2\right)\left(x+2\right)}\right):\left(\frac{6}{x+2}\right)\)
\(=\frac{x^2+4-2x-4+x-2}{\left(x-2\right)\left(x+2\right)}.\frac{x+2}{6}=\frac{x^2-x-2}{6\left(x-2\right)}=\frac{x+1}{6}\)
b, Ta có : \(\left|2x-3\right|=1\Leftrightarrow\orbr{\begin{cases}2x-3=1\\2x-3=-1\end{cases}\Leftrightarrow\orbr{\begin{cases}x=2\left(ktmđk\right)\\x=1\end{cases}}}\)
Thay x = 1 vào biểu thức E ta được : \(\frac{1+1}{6}=\frac{2}{6}=\frac{1}{3}\)
Vậy với x = 1 thì E = 1/3
c, Ta có : \(E< 0\)hay \(\frac{x+1}{6}< 0\Rightarrow x+1>0\)( do 6 > 0 )
\(\Leftrightarrow x>-1\)
Với với x > -1 thì E < 0
d, Ta có E = 3 - x hay \(\frac{x+1}{6}=3-x\Rightarrow x+1=18-6x\Leftrightarrow7x=17\Leftrightarrow x=\frac{17}{7}\)

a) \(\frac{2x^2-4x+8}{x^3+8}\Rightarrow\) ĐKXĐ: \(x^3+8\ne0 \Leftrightarrow x^3\ne-8 \Leftrightarrow x\ne-2 \)
b) \(\frac{2x^2-4x+8}{x^3+8}=\frac{2\left(x^2-2x+4\right)}{\left(x+2\right)\left(x^2-2x+4\right)}=\frac{2}{x+2}\)
c) \(\frac{2}{x+2}\Rightarrow f\left(2\right)=\frac{2}{2+2}=\frac{1}{2}\)
d) \(\frac{2}{x+2}=2\)
\(\Leftrightarrow x+2=1\)
\(\Leftrightarrow x=-1\)
uum, mik nghĩ phần C chỗ x+2=1 thì phải gt tại sao x+2=1 thì đúng hơn