PM
2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
3 tháng 12 2021
c: \(-x^2+2x-2=-\left(x-1\right)^2-1\le-1\forall x\)
\(\Leftrightarrow V\ge-1\forall x\)
Dấu '=' xảy ra khi x=1
PT
1
CM
24 tháng 10 2017
B = 9 x - 3 x 2 = 3 3 x - x 2 = 3 9 / 4 - 9 / 4 + 2 . 3 / 2 x - x 2
= 3 9 / 4 - 9 / 4 - 3 / 2 x + x 2
= 3 9 / 4 - 3 / 2 x - x 2 = 27 / 4 - 3 / 2 - x 2
Vì 3 / 2 - x 2 ≥ 0 với mọi x
⇒ B = 27/4 − 3 / 2 - x 2 ≤ 27/4 do đó giá trị lớn nhất của B bằng 27/4 tại x = 3/2
PM
2
KY
16 tháng 9 2019
Ta có: \(x^2\ge0\)
\(\Leftrightarrow2x^2\ge0\)
\(\Leftrightarrow-2x^2\le0\)
\(\Leftrightarrow4-2x^2\le4\)
Vậy \(B_{max}=4\Leftrightarrow x=0\)
13 tháng 11 2021
\(A=\left(x^2-2x+1\right)+4=\left(x-1\right)^2+4\ge4\\ A_{min}=4\Leftrightarrow x=1\\ B=2\left(x^2-3x\right)=2\left(x^2-2\cdot\dfrac{3}{2}x+\dfrac{9}{4}\right)-\dfrac{9}{2}\\ B=2\left(x-\dfrac{3}{2}\right)^2-\dfrac{9}{2}\ge-\dfrac{9}{2}\\ B_{min}=-\dfrac{9}{2}\Leftrightarrow x=\dfrac{3}{2}\\ C=-\left(x^2-4x+4\right)+7=-\left(x-2\right)^2+7\le7\\ C_{max}=7\Leftrightarrow x=2\)
13 tháng 11 2021
a,\(A=x^2-2x+5=\left(x^2-2x+1\right)+4=\left(x-1\right)^2+4\ge4\)
Dấu "=" \(\Leftrightarrow x=-1\)
b,\(B=2\left(x^2-3x\right)=2\left(x^2-3x+\dfrac{9}{4}\right)-\dfrac{9}{2}=2\left(x-\dfrac{3}{2}\right)^2-\dfrac{9}{2}\ge-\dfrac{9}{2}\)
Dấu "=" \(\Leftrightarrow x=\dfrac{3}{2}\)
c,\(=C=-\left(x^2-4x-3\right)=-\left[\left(x^2-4x+4\right)-7\right]=-\left(x-2\right)^2+7\le7\)
Dấu "=" \(\Leftrightarrow x=2\)
PT
1
NT
1
8 tháng 1 2021
Ta có: \(A=-2x^2-5x+3\)
\(=-2\left(x^2+\dfrac{5}{2}x-\dfrac{3}{2}\right)\)
\(=-2\left(x^2+2\cdot x\cdot\dfrac{5}{4}+\dfrac{25}{16}-\dfrac{49}{16}\right)\)
\(=-2\left(x+\dfrac{5}{4}\right)^2+\dfrac{49}{8}\)
Ta có: \(\left(x+\dfrac{5}{4}\right)^2\ge0\forall x\)
\(\Rightarrow-2\left(x+\dfrac{5}{4}\right)^2\le0\forall x\)
\(\Rightarrow-2\left(x+\dfrac{5}{4}\right)^2+\dfrac{49}{8}\le\dfrac{49}{8}\forall x\)
Dấu '=' xảy ra khi \(x+\dfrac{5}{4}=0\)
hay \(x=-\dfrac{5}{4}\)
Vậy: Giá trị lớn nhất của biểu thức \(A=-2x^2-5x+3\) là \(\dfrac{49}{8}\) khi \(x=-\dfrac{5}{4}\)
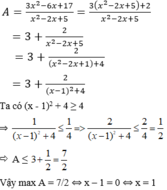
\(D=-3x^2+2x-5\)
\(=-\left(3x^2-2x+5\right)\)
\(=-\left[\left(\sqrt{3}x\right)^2-2.\sqrt{3}x.\frac{2}{\sqrt{3}}+\frac{4}{3}+\frac{11}{3}\right]\)
\(=-\left[\left(\sqrt{3}x-\frac{2}{\sqrt{3}}\right)^2+\frac{11}{3}\right]\)
\(=-\left(\sqrt{3}x-\frac{2}{\sqrt{3}}\right)^2-\frac{11}{3}\le\frac{-11}{3}\)
Vậy \(D_{max}=\frac{-11}{3}\Leftrightarrow\sqrt{3}x-\frac{2}{\sqrt{3}}=0\Leftrightarrow x=\frac{2}{3}\)
bài này làm đúng nhưng mà sai xíu là \(\frac{2}{\sqrt{3}}\)thành \(\frac{1}{\sqrt{3}}\)và \(-\frac{11}{3}\)thành \(-\frac{14}{3}\)