K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
AD
23 tháng 7 2023
\(a,Q=\left(-2x^3y+7x^2y+3xy\right)+P=\left(-2x^3y+7x^2y+3xy\right)+\left(3x^2y-2xy^2-4xy+2\right)\\ =-2x^3y+7x^2y+3xy+3x^2y-3xy^2-4xy+2\\ =-2x^3y^2+10x^2y-3xy^2-xy+2\)
\(b,M=\left(3x^2y^2-5x^2y+8xy\right)-P\\ =\left(3x^2y^2-5x^2y+8xy\right)-\left(3x^2y-2xy^2-4xy+2\right)\\ =3x^2y^2-5x^2y+8xy-3x^2y^2+2xy^2+4xy-2\\ =-3x^2y+12xy-2\)
NV
Nguyễn Việt Lâm
Giáo viên
30 tháng 12 2020
\(p=\left(x-1\right)\left(x+7\right)\left(x+2\right)\left(x+4\right)+2075\)
\(=\left(x^2+6x-7\right)\left(x^2+6x+8\right)+2075\)
\(=\left(x^2+6x+2-9\right)\left(x^2+6x+2+6\right)+2075\)
\(=\left(x^2+6x+2\right)^2-3\left(x^2+6x+2\right)+2021\)
\(\Rightarrow p\) chia q dư \(2021\)
9 tháng 8 2023
a: P(x)-Q(x)+H(x)
=x^3-2x^2+3x+1-x^3-x+1+2x^2-1
=2x+1
b: P(x)-Q(x)+H(x)=0
=>2x+1=0
=>x=-1/2
HP
14 tháng 8 2016
\(P=\left(x-1\right)\left(x+2\right)\left(x+4\right)\left(x+7\right)+2069\)
\(=\left(x-1\right)\left(x+7\right)\left(x+2\right)\left(x+4\right)+2069\)
\(=\left(x^2+6x-7\right)\left(x^2+6x+8\right)+2069\)
\(=\left(x^2+6x+2-9\right)\left(x^2+6x+2+6\right)+2069\)
Mà \(x^2+6x+2=Q\)
\(=>P=\left(Q-9\right)\left(Q+6\right)+2069=Q^2-3Q-54+2069\)
\(=Q^2-3Q+2015=Q\left(Q-3\right)+2015\)
Dễ thấy \(Q\left(Q-3\right)=BS\left(Q\right)\)
\(=>P\)chia Q có số dư là 2015
Vậy................
SG
1
19 tháng 10 2019
c) Cách 1:
Để \(P\left(x\right)⋮Q\left(x\right)\)
\(\Leftrightarrow\left(a+3\right)x+b=0\)
\(\Leftrightarrow\hept{\begin{cases}a+3=0\\b=0\end{cases}\Leftrightarrow}\hept{\begin{cases}a=-3\\b=0\end{cases}}\)
Vậy a=-3 và b=0 để \(P\left(x\right)⋮Q\left(x\right)\)
19 tháng 10 2019
a)
Để \(2n^2-n+2⋮2n+1\)
\(\Leftrightarrow3⋮2n+1\)
\(\Leftrightarrow2n+1\inƯ\left(3\right)=\left\{\pm1;\pm3\right\}\)
\(\Leftrightarrow n\in\left\{0;1;-2;-1\right\}\)
Vậy \(n\in\left\{0;1;-2;-1\right\}\)để \(2n^2-n+2⋮2n+1\)
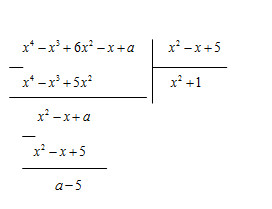
Q(x).( x - 2 ) + 28 = ( x2 + x + 1 )( x + 2 )
⇔ Q(x).( x - 2 ) = x3 + 3x2 + 3x + 2 - 28
⇔ Q(x).( x - 2 ) = x3 + 3x2 + 3x - 26
⇔ Q(x).( x - 2 ) = x3 - 2x2 + 5x2 - 10x + 13x - 26
⇔ Q(x).( x - 2 ) = x2( x - 2 ) + 5x( x - 2 ) + 13( x - 2 )
⇔ Q(x).( x - 2 ) = ( x - 2 )( x2 + 5x + 13 )
⇔ Q(x) = x2 + 5x + 13