Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

b: Để A là số nguyên thì \(2x+2⋮x+3\)
\(\Leftrightarrow x+3\in\left\{1;-1;2;-2;4;-4\right\}\)
hay \(x\in\left\{-4;-1;-5;1;-7\right\}\)

\(A=\frac{x^3-x^2+2}{x-1}=x^2+\frac{2}{x-1}\inℤ\Leftrightarrow\frac{2}{x-1}\inℤ\)
mà \(x\inℤ\)nên \(x-1\inƯ\left(2\right)=\left\{-2,-1,1,2\right\}\)
\(\Leftrightarrow x\in\left\{-1,0,2,3\right\}\).

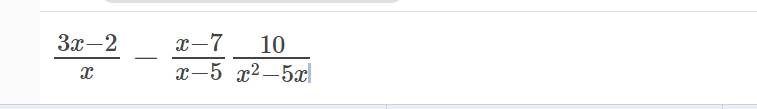
a: Sửa đề: \(A=\dfrac{3x-2}{x}-\dfrac{x-7}{x-5}-\dfrac{10}{x^2-5x}\)
\(=\dfrac{3x-2}{x}-\dfrac{x-7}{x-5}-\dfrac{10}{x\left(x-5\right)}\)
\(=\dfrac{\left(3x-2\right)\left(x-5\right)-x\left(x-7\right)-10}{x\left(x-5\right)}\)
\(=\dfrac{3x^2-15x-2x+10-x^2+7x-10}{x\left(x-5\right)}\)
\(=\dfrac{2x^2-10x}{x\left(x-5\right)}=\dfrac{2\left(x^2-5x\right)}{x\left(x-5\right)}=2\)
b: \(B=A\cdot\dfrac{x+1}{x-1}=\dfrac{2x+2}{x-1}\)(ĐKXĐ: x<>1)
Để B là số nguyên thì \(2x+2⋮x-1\)
=>\(2x-2+4⋮x-1\)
=>\(4⋮x-1\)
=>\(x-1\in\left\{1;-1;2;-2;4;-4\right\}\)
=>\(x\in\left\{2;0;3;-1;5;-3\right\}\)
Kết hợp ĐKXĐ của cả A và B, ta được: \(x\in\left\{2;3;-1;-3\right\}\)

a: \(A=\dfrac{x^2-8x+16-x^2+16}{\left(x-4\right)\left(x+4\right)}\cdot\dfrac{x}{2\left(x-1\right)}\)
\(=\dfrac{-8\left(x-4\right)}{\left(x-4\right)\left(x+4\right)}\cdot\dfrac{x}{2\left(x-1\right)}\)
\(=\dfrac{-4x}{\left(x+4\right)\left(x-1\right)}\)

\(A=\left(\dfrac{x}{x^2-4}+\dfrac{2}{2-x}+\dfrac{1}{x+2}\right):\left(x-2+\dfrac{10-x^2}{x+2}\right)\)
\(\Rightarrow A=\left(\dfrac{x-2\left(x+2\right)+1\left(x-2\right)}{\left(x-2\right)\left(x+2\right)}\right):\left(\dfrac{\left(x-2\right)\left(x+2\right)+10-x^2}{x+2}\right)\)
\(\Rightarrow A=\left(\dfrac{-6}{x^2-4}\right):\left(\dfrac{6}{x+2}\right)\)
\(\Rightarrow A=-\dfrac{6}{x^2-4}.\dfrac{x+2}{6}=-\dfrac{6\left(x+2\right)}{\left(x-2\right)\left(x+2\right)6}=-\dfrac{1}{x-2}\)
để A<0 thì :
\(\left\{{}\begin{matrix}x-2\ne0\\x-2\notin Z-\end{matrix}\right.\)\(\Leftrightarrow x\in\left\{3;4;5;6;7;8;9;....n\right\}\)
( Z- là tập hợp số nguyên âm )
Để A có giá trị nguyên thì :
\(\left\{{}\begin{matrix}x-2=1\\x-2=-1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=3\\x=1\end{matrix}\right.\)
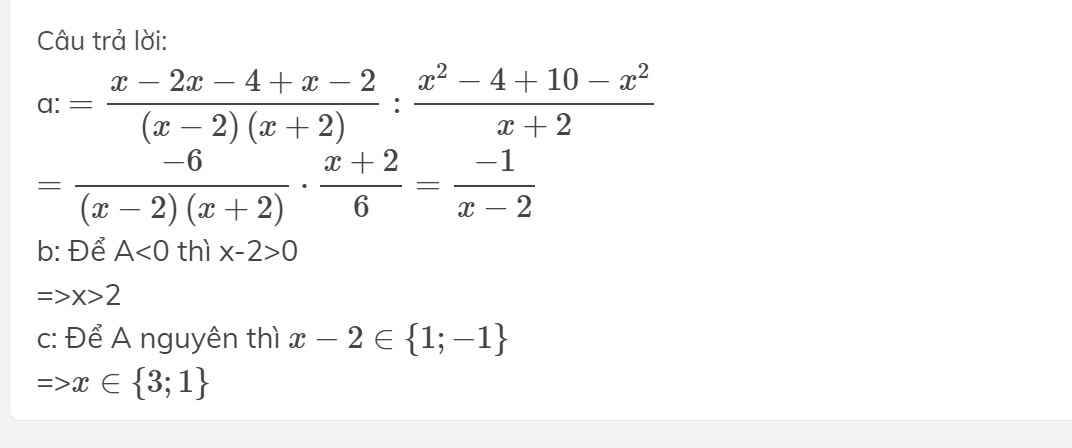
để P có giá trị nguyên <=> \(2⋮\left(x-1\right)\)
\(\Rightarrow\) x-1 là ước của 2
=> \(\left[{}\begin{matrix}x-1=1\\x-1=-1\\x-1=2\\x-1=-2\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=2\\x=0\\x=3\\x=-1\left(loai\right)\end{matrix}\right.\)