
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(11,=\dfrac{\sqrt{10}}{5}=\dfrac{1}{5}\sqrt{10}\left(A\right)\\ 12,=\dfrac{2\left(\sqrt{5}+3\right)}{-4}=-\dfrac{\sqrt{5}+3}{2}\left(B\right)\\ 13,=\dfrac{\sqrt{a}}{3\sqrt{3}}=\dfrac{\sqrt{3a}}{9}\left(A\right)\\ 14,B\)

đề đây nha mn giúp mik vs ạ
cho tam giác ABC nhọn (AB<AC) nội tiếp đường tròn (O;K) có BD là đường kính và đường cao AH của tam giác ABC cắt (O;K) tại E




\(\text{Δ}=\left(-2m\right)^2-4\left(m^2-m-2\right)\)
\(=4m^2-4m^2+4m+8\)
=4m+8
Để phương trình có hai nghiệm thì 4m+8>=0
hay m>=-2
Theo đề, ta có: \(\left(x_1+x_2\right)^2-2x_1x_2=4\)
\(\Leftrightarrow\left(-2m\right)^2-2\left(m^2-m-2\right)=4\)
\(\Leftrightarrow4m^2-2m^2+2m=0\)
=>2m(m+1)=0
=>m=0 hoặc m=-1

Câu 5:
$\frac{20}{\sqrt{5}}=\frac{20\sqrt{5}}{5}=4\sqrt{5}$
Câu 6:
\(\frac{3}{\sqrt{5}+\sqrt{2}}+\frac{3}{\sqrt{5}-\sqrt{2}}=3.\frac{\sqrt{5}-\sqrt{2}+\sqrt{5}+\sqrt{2}}{(\sqrt{5}+\sqrt{2})(\sqrt{5}-\sqrt{2})}=3.\frac{2\sqrt{5}}{5-2}=2\sqrt{5}\)
Câu 7:
1. ĐKXĐ: $x\neq 1; x\geq 0$
\(A=\left[\frac{\sqrt{x}(\sqrt{x}+1)}{\sqrt{x}+1}+1\right]:\left[\frac{\sqrt{x}(\sqrt{x}-1)}{\sqrt{x}-1}-1\right]=(\sqrt{x}+1):(\sqrt{x}-1)\)
\(=\frac{\sqrt{x}+1}{\sqrt{x}-1}\)
2.
\(A< 1\Leftrightarrow \frac{\sqrt{x}+1}{\sqrt{x}-1}-1<0\Leftrightarrow \frac{2}{\sqrt{x}-1}<0\)
\(\Leftrightarrow \sqrt{x}-1<0\Leftrightarrow x< 1\)
Kết hợp ĐKXĐ suy ra $0\leq x< 1$



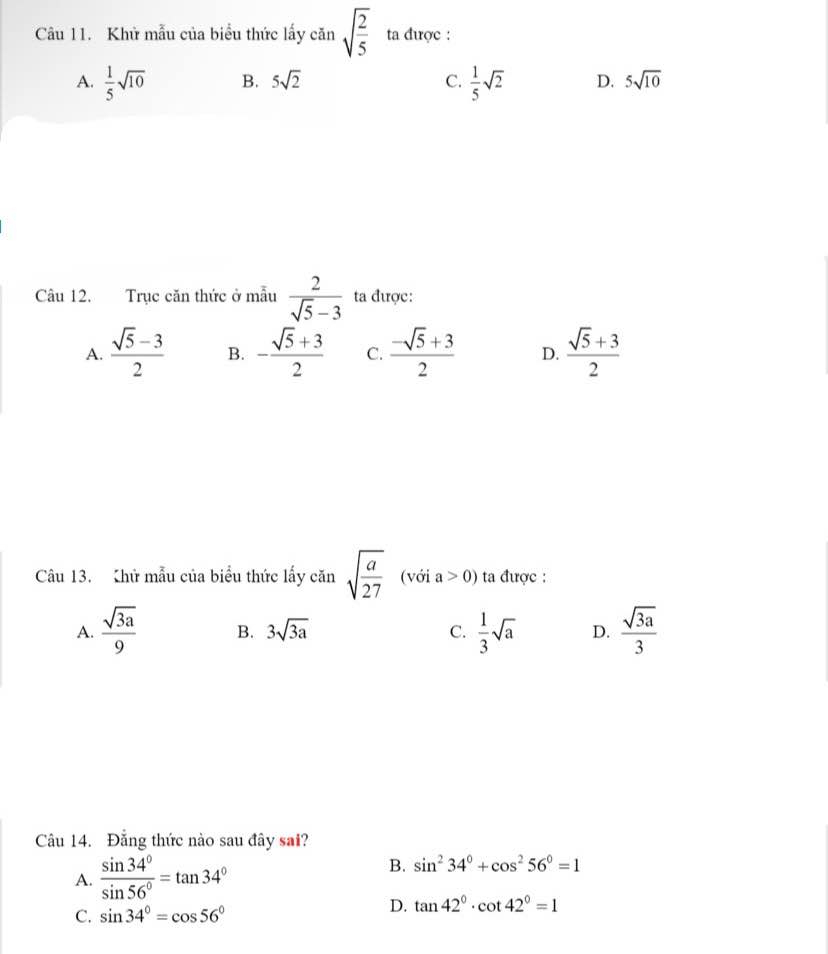
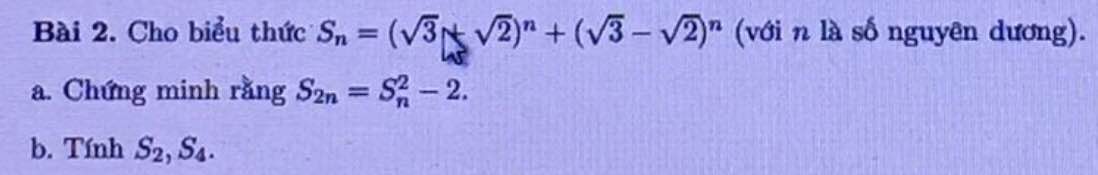 mọi người giải giúp em bài này với ạ 🥺
mọi người giải giúp em bài này với ạ 🥺



 Ai giúp mình giải câu 3 với,mình cảm ơn nhìuuuu ạ 🥺
Ai giúp mình giải câu 3 với,mình cảm ơn nhìuuuu ạ 🥺
2. C
3A
4A
5C
\(2,ĐK:\left\{{}\begin{matrix}\dfrac{2}{a+5}\ge0\\a+5\ne0\end{matrix}\right.\Leftrightarrow a+5>0\Leftrightarrow a>-5\left(C\right)\\ 3,M=2\sqrt{3}=\sqrt{12}< \sqrt{15}=N\left(C\right)\\ 4,=\left|3-\sqrt{3}\right|=3-\sqrt{3}\left(A\right)\\ 5,=\dfrac{3\sqrt{5}-3\sqrt{3}+3\sqrt{5}+3\sqrt{3}}{\left(\sqrt{5}+\sqrt{3}\right)\left(\sqrt{5}-\sqrt{3}\right)}=\dfrac{6\sqrt{5}}{2}=3\sqrt{5}\left(C\right)\)