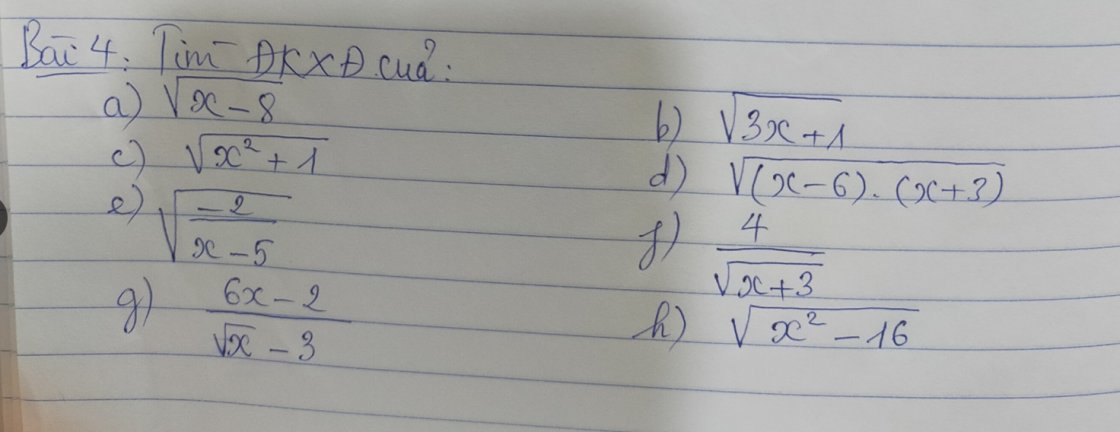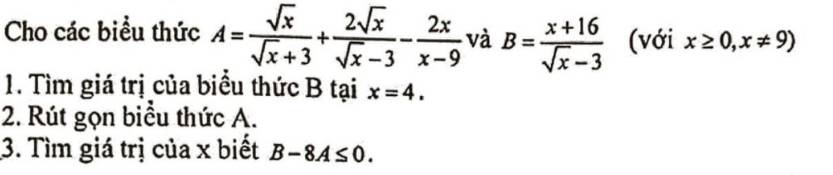Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Bài 2:
a: Xét tứ giác ABOC có
\(\widehat{ABO}+\widehat{ACO}=180^0\)
Do đó: ABOC là tứ giác nội tiếp

\(2,\Leftrightarrow\left\{{}\begin{matrix}20x+25y-10xy=0\\20x-30y+xy=0\end{matrix}\right.\Leftrightarrow55y-11xy=0\\ \Leftrightarrow11y\left(5-x\right)=0\Leftrightarrow\left[{}\begin{matrix}y=0\\x=5\end{matrix}\right.\)
Với \(y=0\Leftrightarrow4x+0=0\Leftrightarrow x=0\)
Với \(x=5\Leftrightarrow20+5y=10y\Leftrightarrow y=4\)
Vậy \(\left(x;y\right)=\left\{\left(0;0\right);\left(5;4\right)\right\}\)

a) \(\sqrt{x-8}\) xác định khi
\(x-8\ge0\Leftrightarrow x\ge8\)
b) \(\sqrt{3x+1}\) xác định khi
\(3x+1\ge0\Leftrightarrow3x\ge-1\Leftrightarrow x\le-\dfrac{1}{3}\)
c) \(\sqrt{x^2+1}\)
Ta có: \(x^2\ge0\Rightarrow x^2+1\ge0\)
Vậy biểu thức được xác định với mọi x
d) \(\sqrt{\left(x-6\right)\left(x+3\right)}\)
Xác định khi
\(\left\{{}\begin{matrix}\left\{{}\begin{matrix}x-6\ge0\\x+3\ge0\end{matrix}\right.\\\left\{{}\begin{matrix}x-6< 0\\x+3\ge0\end{matrix}\right.\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left\{{}\begin{matrix}x\ge6\\x\ge-3\end{matrix}\right.\\\left\{{}\begin{matrix}x< 6\\x< -3\end{matrix}\right.\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge6\\x< -3\end{matrix}\right.\)
e) \(\sqrt{\dfrac{-2}{x-5}}\) xác định khi
\(\left\{{}\begin{matrix}\dfrac{-2}{x-5}\ge0\\x-5\ne0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\le5\\x\ne5\end{matrix}\right.\)
\(\Leftrightarrow x< 5\)
f) \(\dfrac{4}{\sqrt{x+3}}\) xác định khi
\(x+3>0\)
\(\Leftrightarrow x>-3\)
g) \(\dfrac{6x-2}{\sqrt{x}-3}\)
Xác định khi:
\(\left\{{}\begin{matrix}\sqrt{x}\ge0\\\sqrt{x}-3\ne0\end{matrix}\right.\)
\(\left\{{}\begin{matrix}x\ge0\\x\ne9\end{matrix}\right.\)
h) \(\sqrt{x^2-16}=\sqrt{\left(x+4\right)\left(x-4\right)}\)
Xác định khi
\(\left\{{}\begin{matrix}\left\{{}\begin{matrix}x+4< 0\\x-4< 0\end{matrix}\right.\\\left\{{}\begin{matrix}x+4\ge0\\x-4\ge0\end{matrix}\right.\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left\{{}\begin{matrix}x< -4\\x< 4\end{matrix}\right.\\\left\{{}\begin{matrix}x\ge-4\\x\ge4\end{matrix}\right.\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x< -4\\x\ge4\end{matrix}\right.\)

Câu 6
Thay x=-1 và y=2 vào (d), ta được:
-m+1+2m-3=2
\(\Leftrightarrow m=4\)
Câu 5:
Gọi đths cần tìm là \(y=ax+b\left(a\ne0\right)\)
Vì đt vuông góc với \(y=2x+7\) nên \(2a=-1\Leftrightarrow a=-\dfrac{1}{2}\)
Do đó hệ số góc của đt là \(a=-\dfrac{1}{2}\)

Rút gọn được \(A=\dfrac{\sqrt{x}}{\sqrt{x}-3}\)
\(B-8A\le0\Leftrightarrow\dfrac{x+16}{\sqrt{x}-3}-\dfrac{8\sqrt{x}}{\sqrt{x}-3}\le0\)
\(\Leftrightarrow\dfrac{x-8\sqrt{x}+16}{\sqrt{x}-3}\le0\)
\(\Leftrightarrow\dfrac{\left(\sqrt{x}-4\right)^2}{\sqrt{x}-3}\le0\)
\(\Rightarrow\left[{}\begin{matrix}\sqrt{x}-4=0\\\sqrt{x}-3< 0\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=16\\x< 9\end{matrix}\right.\)
Kết hợp ĐKXD ta được: \(\left[{}\begin{matrix}x=16\\0\le x< 9\end{matrix}\right.\)

b: =(m-1)^2-4(-m^2-2)
=m^2+2m+1+4m^2+8
=5m^2+2m+9
=5(m^2+2/5m+9/5)
=5(m^2+2*m*1/5+1/25+44/25)
=5(m+1/5)^2+44/5>=44/5>0 với mọi m
=>PT luôn có hai nghiệm pb

3.2:
Theo vi ét: \(\left\{{}\begin{matrix}x_1+x_2=2m+2\\x_1x_2=m^2+m\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left(x_1+x_2\right)^2=\left(2m+2\right)^2=4m^2+8m+4\\4x_1x_2=4m^2+4m\end{matrix}\right.\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-4x_1x_2=4m+4=2\left(2m+2\right)=2\left(x_1+x_2\right)\)
\(\Rightarrow\left(x_1+x_2\right)^2-4x_1x_2-2\left(x_1+x_2\right)=4m^2+8m+4-4m^2-4m-4m-4=0\)
Vậy hệ thức liên hệ giữa \(x_1\) và \(x_2\) mà không phụ thuộc vào tham số m là \(\left(x_1+x_2\right)^2-4x_1x_2-2\left(x_1+x_2\right)\)
2: x1+x2=2m+2; x1x2=m^2+m
(x1+x2)^2-4x1x2
=4m^2+8m+4-4m^2-4m=4m+4
=>(x1+x2)^2-4x1x2-2(x1+x2)=4m+4-4m-4=0 ko phụ thuộc m

 không cần làm câu c đâu ạ, giúp e với nhaaa mn
không cần làm câu c đâu ạ, giúp e với nhaaa mn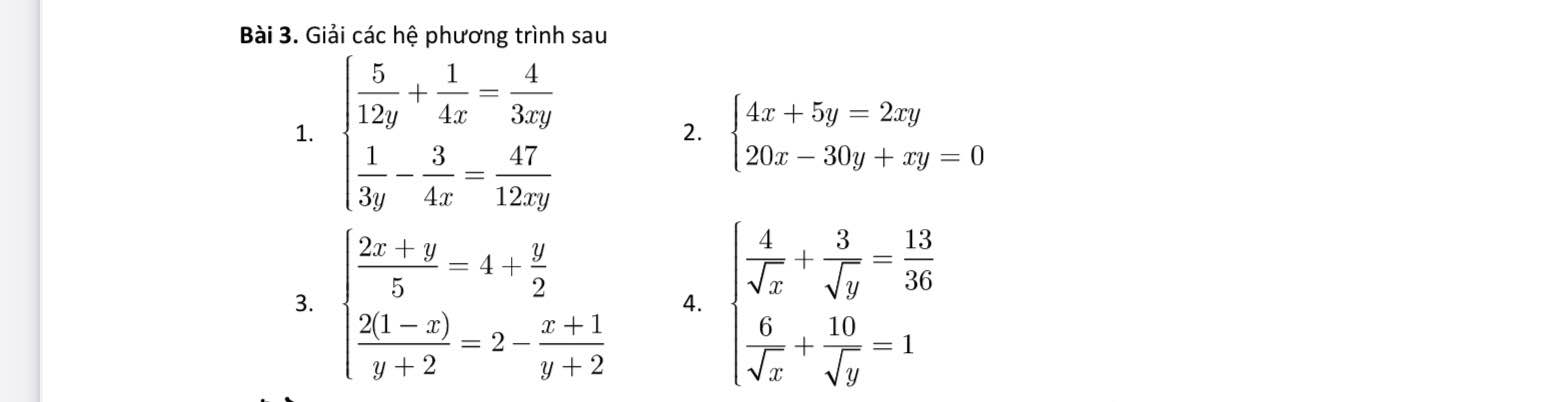 không cần làm hết đâu ạ chỉ cần 2,3 câu là đc ạ
không cần làm hết đâu ạ chỉ cần 2,3 câu là đc ạ