Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: Thay x=-1 vào (6), ta được:
1+2m+m+6=0
=>3m+7=0
=>m=-7/3
x1+x2=-2m/1=-2*7/3=-14/3
=>x2=-14/3-x1=-14/3+1=-11/3
b: \(\text{Δ}=0^2-2\left(2m+m+6\right)=-2\left(3m+6\right)\)
Để phương trình có nghiệm kép thì 3m+6=0
=>m=-2
Khi m=-2 thì (6) sẽ là x^2+2*(-2)-2+6=0
=>x^2-4x+4=0
=>x=2
ụa bạn ơi, trên câu a á m= -7/3 vậy sao xuống dưới thành 7/3 rồi

1: \(\text{Δ}=\left(-1\right)^2-4\cdot1\cdot\left(-m+1\right)\)
=1+4m-4
=4m-3
Để phương trình có nghiệm kép thì 4m-3=0
hay m=3/4
Thay m=3/4 vào pt, ta được: \(x^2-x+\dfrac{1}{4}=0\)
hay x=1/2
2: Để phương trình có hai nghiệm thì 4m-3>=0
hay m>=3/4
Theo đề, ta có hệ phương trình:
\(\left\{{}\begin{matrix}2x_1+x_2=5\\x_1+x_2=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x_1=4\\x_2=-3\end{matrix}\right.\)
Theo đề, ta có: \(x_1x_2=-m+1\)
=>1-m=-12
hay m=13

\(a,\)Để pt \(x^2+\left(2m+1\right)x+m\left(m-1\right)=0\) có nghiệm kép thì \(\Delta=0\)
\(\Leftrightarrow\left(2m+1\right)^2-4\left(m^2-m\right)=0\)
\(\Leftrightarrow4m^2+4m+1-4m^2+4m=0\)
\(\Leftrightarrow8m+1=0\)
\(\Leftrightarrow m=-\dfrac{1}{8}\)
Thay \(m=-\dfrac{1}{8}\) vào pt
\(\Rightarrow x^2+\left[2.\left(-\dfrac{1}{8}\right)+1\right]x-\dfrac{1}{8}\left(-\dfrac{1}{8}-1\right)=0\)
\(\Rightarrow x^2+\dfrac{3}{4}x+\dfrac{9}{64}=0\)
\(\Rightarrow x=-\dfrac{3}{8}\)
\(b,\) Thay \(m=1\) vào pt :
\(\Rightarrow x^2+\left(2.1+1\right)x+1\left(1-1\right)=0\)
\(\Rightarrow x^2+3x=0\)
\(\Rightarrow\left[{}\begin{matrix}x=0\\x=-3\end{matrix}\right.\)

A, ta có: \(\Delta’\)=m2-1
Vậy trình có 2 nghiệm phân biệt <=> m2-1>0 => m>1
B,Phương trình có nghiệm kép khi: m2-1=0 => m=+- 1
Nghiem kép đó là: 0
\(x^2+2\left(m+1\right)x+2m+2=0\)
\(\Delta'=\left(m+1\right)^2-\left(2m+2\right)=m^2-1\)
a, Để phương trình có hai nghiệm phân biệt thì:
\(\Delta'>0\)
\(\Leftrightarrow m^2>1\)
\(\Leftrightarrow m^2-1>0\)
\(\Leftrightarrow m< -1;m>1\)
b, Phương trinh có nghiệm kép khi:
\(\Delta'\ge0\)
\(\Leftrightarrow m^2-1\ge0\)
\(\Leftrightarrow m\le-1;m\ge1\)
Theo Viet ta có:
\(x_1+x_2=-2\left(m+1\right)\)
\(x_1x_2=2\left(m+1\right)\)
\(x_1^2+x_2^2=8\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=8\)
\(\Leftrightarrow4m^2+4m-8=0\)
\(\Leftrightarrow\orbr{\begin{cases}m=1\\m=-2\end{cases}}\)
So với điều kiện phương trình có nghiệm m=1 ; m =-2

b) Thay x=2 vào pt, ta được:
\(4\left(m^2-1\right)-4m+m^2+m+4=0\)
\(\Leftrightarrow4m^2-4-4m+m^2+m+4=0\)
\(\Leftrightarrow5m^2-3m=0\)
\(\Leftrightarrow m\left(5m-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m=0\\m=\dfrac{3}{5}\end{matrix}\right.\)
Áp dụng hệ thức Vi-et, ta được:
\(x_1+x_2=\dfrac{2m}{m^2-1}\)
\(\Leftrightarrow\left[{}\begin{matrix}x_2+2=0\\x_2+2=\dfrac{6}{5}:\left(\dfrac{36}{25}-1\right)=\dfrac{30}{11}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x_2=-2\\x_2=\dfrac{8}{11}\end{matrix}\right.\)

a. Bạn tự giải
b. Pt có nghiệm kép khi:
\(\Delta'=\left(m+1\right)^2-4m=0\Leftrightarrow m^2-2m+1=0\Leftrightarrow m=1\)
Khi đó: \(x_{1,2}=m+1=2\)
c. Do pt có nghiệm bằng 4:
\(\Rightarrow4^2-2\left(m+1\right).4+4m=0\)
\(\Leftrightarrow8-4m=0\Rightarrow m=2\)
\(x_1x_2=4m\Rightarrow x_2=\dfrac{4m}{x_1}=\dfrac{4.2}{4}=2\)
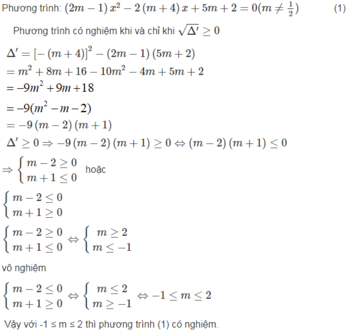
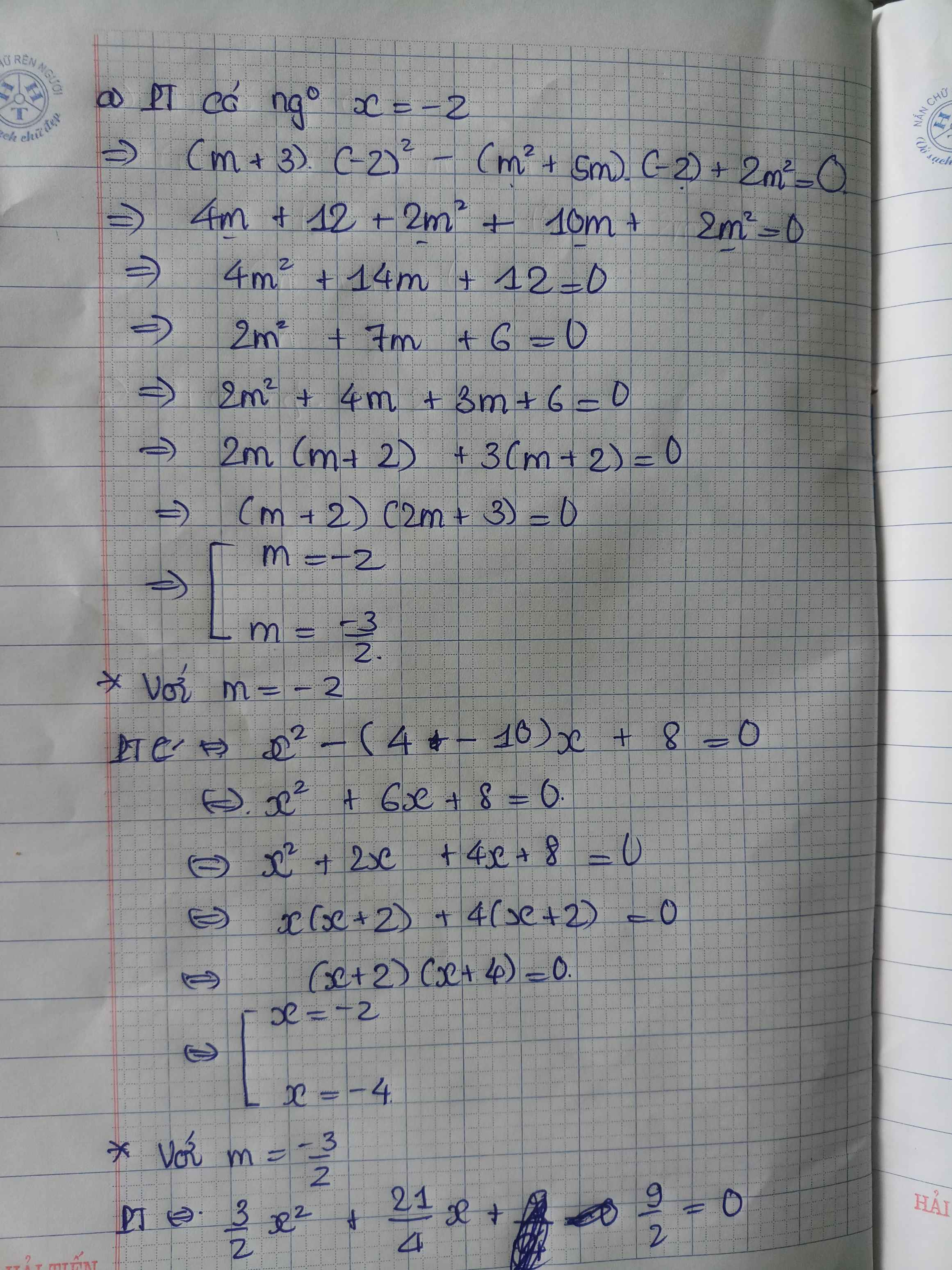

Bạn giải denta và chú ý điều kiện của a nhá