Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Ta có:
\(\overrightarrow {GA} + \overrightarrow {GB} + \overrightarrow {GC} + \overrightarrow {GD} = \overrightarrow 0 \Leftrightarrow \left( {\overrightarrow {GI} + \overrightarrow {IA} } \right) + \left( {\overrightarrow {GI} + \overrightarrow {IB} } \right) + \left( {\overrightarrow {GJ} + \overrightarrow {JC} } \right) + \left( {\overrightarrow {GJ} + \overrightarrow {JD} } \right) = \overrightarrow 0 \)
\( \Leftrightarrow 2\overrightarrow {GI} + \left( {\overrightarrow {IA} + \overrightarrow {IB} } \right) + 2\overrightarrow {GJ} + \left( {\overrightarrow {JC} + \overrightarrow {JD} } \right) = \overrightarrow 0 \)
\( \Leftrightarrow 2\overrightarrow {GI} + 2\overrightarrow {GJ} = \overrightarrow 0 \Leftrightarrow 2\left( {\overrightarrow {GI} + \overrightarrow {GJ} } \right) = \overrightarrow 0 \)
\( \Leftrightarrow \overrightarrow {GI} + \overrightarrow {GJ} = \overrightarrow 0 \Rightarrow \)G là trung điểm của đoạn thẳng IJ
Vậy I, G, J thẳng hàng

a: \(\overrightarrow{AE}=\dfrac{2}{3}\overrightarrow{EC}\)
=>E nằm giữa A và C và AE=2/3EC
Ta có: AE+EC=AC(E nằm giữa A và C)
=>\(AC=\dfrac{2}{3}EC+EC=\dfrac{5}{3}EC\)
=>\(\dfrac{AE}{AC}=\dfrac{\dfrac{2}{3}EC}{\dfrac{5}{3}EC}=\dfrac{2}{3}:\dfrac{5}{3}=\dfrac{2}{5}\)
=>\(AE=\dfrac{2}{5}AC\)
=>\(\overrightarrow{AE}=\dfrac{2}{5}\cdot\overrightarrow{AC}\)
\(\overrightarrow{BE}=\overrightarrow{BA}+\overrightarrow{AE}\)
\(=-\overrightarrow{AB}+\dfrac{2}{5}\cdot\overrightarrow{AC}\)
b: \(\left|\overrightarrow{IA}+\overrightarrow{IG}\right|=\left|\overrightarrow{IA}-\overrightarrow{IG}\right|\)
=>\(\left[{}\begin{matrix}\overrightarrow{IA}+\overrightarrow{IG}=\overrightarrow{IA}-\overrightarrow{IG}\\\overrightarrow{IA}+\overrightarrow{IG}=\overrightarrow{IG}-\overrightarrow{IA}\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}2\cdot\overrightarrow{IG}=\overrightarrow{0}\\2\cdot\overrightarrow{IA}=\overrightarrow{0}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}I\equiv G\\I\equiv A\end{matrix}\right.\)

Ta có:
\(\overrightarrow{MN}=\overrightarrow{MA}+\overrightarrow{MB}+4\overrightarrow{MC}\)
\(=6\overrightarrow{MI}+\overrightarrow{IA}+\overrightarrow{IB}+4\overrightarrow{IC}\)
\(=6\overrightarrow{MI}+4\overrightarrow{IG}+4\overrightarrow{IC}\)
\(=6\overrightarrow{MI}\)
\(\Rightarrow M,I,N\) thẳng hàng

Lời giải:
a.
$\overrightarrow{AC}=\overrightarrow{AB}+\overrightarrow{AD}$ (tính chất hình bình hành)
b.
$\overrightarrow{AM}=\frac{2}{3}\overrightarrow{AC}=\frac{2}{3}(\overrightarrow{AB}+\overrightarrow{AD})$
c.
$\overrightarrow{AN}=\overrightarrow{AC}+\overrightarrow{CN}=\overrightarrow{AC}+\frac{1}{2}\overrightarrow{BA}$
$=\overrightarrow{AB}+\overrightarrow{AD}-\frac{1}{2}\overrightarrow{AB}$
$=\frac{1}{2}\overrightarrow{AB}+\overrightarrow{AD}$
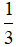

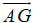
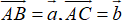
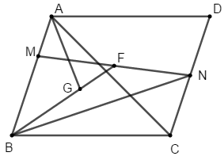

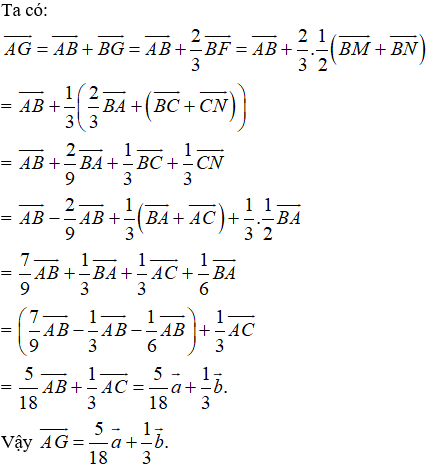
a, Ta có:AM+AN=OM-OA+ON-OA=OM+ON+AC=OC+AC=3/2OC
GA+3GB+GC+OD=2GB+OD=OB+OD=0
C,