
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a: Thay x=81 vào C, ta được:
\(C=\dfrac{81-1}{4\cdot9}=\dfrac{80}{36}=\dfrac{20}{9}\)

3:
b: x1^2+x2^2=12
=>(x1+x2)^2-2x1x2=12
=>(2m+2)^2-4m=12
=>4m^2+4m+4=12
=>m^2+m+1=3
=>(m+2)(m-1)=0
=>m=1;m=-2
2:
b: =>|x1|-|x2|=m+3-|-1|=m+2
=>x1^2+x2^2-2|x1x2|=m+2
=>(x1+x2)^2-2x1x2-2|x1x2|=m+2
=>(2m)^2-2(-1)-2|-1|=m+2
=>4m^2-m-2=0
=>m=(1+căn 33)/8; m=(1-căn 33)/8

\(1,\\ a,x^4-8x^2-9=0\\ \Leftrightarrow x^4+x^2-9x^2-9=0\\ \Leftrightarrow\left(x^2+1\right)\left(x^2-9\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x^2+1=0\\x-3=0\\x+3=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x\in\varnothing\left(x^2+1\ge1>0\right)\\x=3\\x=-3\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=3\\x=-3\end{matrix}\right.\\ b,\left\{{}\begin{matrix}2\left(x-1\right)-3\left(x-3y\right)=5\\3\left(x-1\right)+5\left(x-3y\right)=-2\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}6\left(x-1\right)-9\left(x-3y\right)=15\\6\left(x-1\right)+10\left(x-3y\right)=-4\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}19\left(x-3y\right)=-19\\3\left(x-1\right)+5\left(x-3y\right)=-2\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x-3y=-1\\3\left(x-1\right)-5=-2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x-3y=-1\\x-1=-\dfrac{7}{3}\end{matrix}\right.\\ \Leftrightarrow\left\{{}\begin{matrix}x=-\dfrac{4}{3}\\-\dfrac{4}{3}-3y=-1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-\dfrac{4}{3}\\y=-\dfrac{1}{9}\end{matrix}\right.\)
5b.
Theo Bunhiacopxki:
\(\left(\sqrt{x\left(2x+y\right)}+\sqrt{y\left(2y+x\right)}\right)^2\le\left(x+y\right)\left(\left(2x+y\right)+\left(2y+x\right)\right)=3\left(x+y\right)^2\)
\(\Rightarrow\sqrt{x\left(2x+y\right)}+\sqrt{y\left(2y+x\right)}\le\sqrt{3}\left(x+y\right)\)
\(\Rightarrow\dfrac{x+y}{\sqrt{x\left(2x+y\right)}+\sqrt{y\left(2y+x\right)}}\ge\dfrac{x+y}{\sqrt{3}\left(x+y\right)}=\dfrac{1}{\sqrt{3}}\)
Dấu "=" xảy ra khi x=y




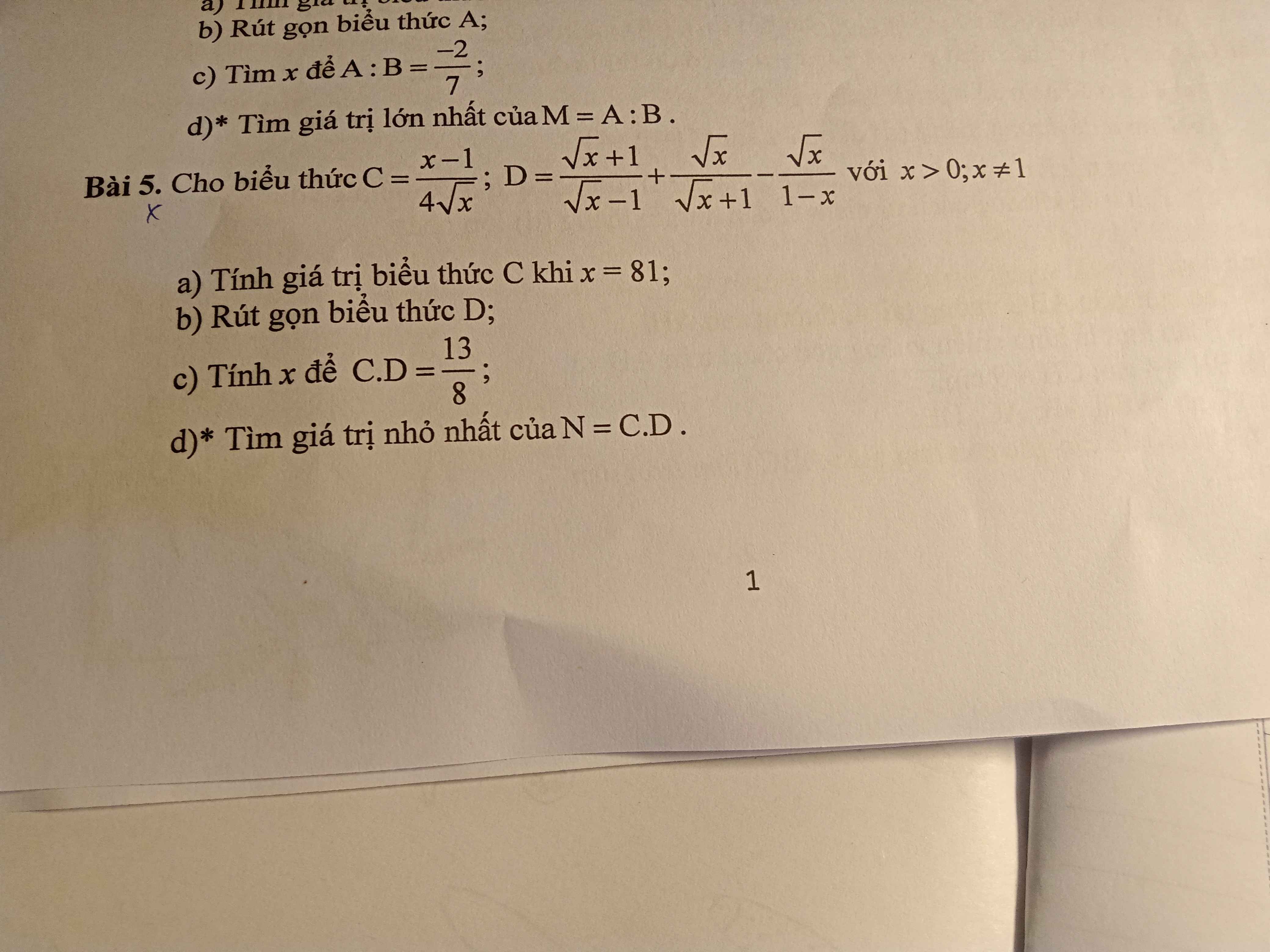

 Các cao nhân giúp em bài màu hồng hồng với ạ, em bị ngáo tạm thời. Mong giúp em chứ em làm nãy h kh ra ạ
Các cao nhân giúp em bài màu hồng hồng với ạ, em bị ngáo tạm thời. Mong giúp em chứ em làm nãy h kh ra ạ






