giúp một tay (x+2015)7=(x+2015)5
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
ND
2
7 tháng 9 2021
\(\dfrac{x+5}{2015}+\dfrac{x+6}{2014}+\dfrac{x+7}{2013}+\dfrac{x+8}{2012}=-4\\ \Leftrightarrow\left(\dfrac{x+5}{2015}+1\right)+\left(\dfrac{x+6}{2014}+1\right)+\left(\dfrac{x+7}{2013}+1\right)+\left(\dfrac{x+8}{2012}+1\right)=0\\ \Leftrightarrow\dfrac{x+2020}{2015}+\dfrac{x+2020}{2014}+\dfrac{x+2020}{2013}+\dfrac{x+2020}{2012}=0\\ \Leftrightarrow\left(x+2020\right)\left(\dfrac{1}{2015}+\dfrac{1}{2014}+\dfrac{1}{2013}+\dfrac{1}{2012}\right)=0\\ \Leftrightarrow x+2020=0\\ \Leftrightarrow x=-2020\)
Vậy x = -2020 là nghiệm của pt.
7 tháng 9 2021
\(\dfrac{x+5}{2015}+\dfrac{x+6}{2014}+\dfrac{x+7}{2013}+\dfrac{x+8}{2012}=-4\)
\(\Leftrightarrow x+2020=0\)
hay x=-2020
20 tháng 8 2015
x8 - 2015.x7 + 2015.x6 - 2015.x5 + .... - 2015.x + 2015
= x^8 - (x+1)x^7 + (x+1)x^6 -(x+1)x^5 +(x+1)x^4+...-(x+1) + 2015
= x^8 -x^8 - x^7 + x^7 + x^6 -x^6 -x^5 + x^5 + x^4 + ...-x - 1+ 2015
=2014
NM
3
10 tháng 7 2017
a) \(\frac{x+2015}{5}+\frac{x+2015}{6}=\frac{x+2015}{7}+\frac{x+2015}{8}\)
\(\frac{x+2015}{5}+\frac{x+2015}{6}-\frac{x+2015}{7}-\frac{x+2015}{8}=0\)
\(\left(x+2015\right).\left(\frac{1}{5}+\frac{1}{6}-\frac{1}{7}-\frac{1}{8}\right)=0\)
vì \(\frac{1}{5}+\frac{1}{6}-\frac{1}{7}-\frac{1}{8}\ne0\)
\(\Rightarrow\)x + 2015 = 0
\(\Rightarrow\)x = -2015
b) Tương tự
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
12 tháng 5 2023
a, \(13\) \(\times\) 15 - 150 + 97 \(\times\) 15
= 15 \(\times\) ( 13 - 10 + 97)
= 15 \(\times\) ( 3 + 97)
= 15 \(\times\) 100
= 1500
b, \(\dfrac{2016}{2015}\) \(\times\) \(\dfrac{4}{7}\) - \(\dfrac{4}{7}\) \(\times\) \(\dfrac{1}{2015}\) + \(\dfrac{3}{7}\)
= \(\dfrac{4}{7}\) \(\times\) ( \(\dfrac{2016}{2015}\) - \(\dfrac{1}{2015}\)) + \(\dfrac{3}{7}\)
= \(\dfrac{4}{7}\) \(\times\) \(\dfrac{2015}{2015}\) + \(\dfrac{3}{7}\)
= \(\dfrac{4}{7}\) + \(\dfrac{3}{7}\)
= \(\dfrac{7}{7}\)
= 1
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
12 tháng 5 2023
a, 13 \(\times\) 15 - 150 + 97 \(\times\)15
13 \(\times\) 15 - 15 \(\times\) 10 + 97 \(\times\) 15
= 15 \(\times\) ( 13 - 10 + 97)
= 15 \(\times\) ( 3 + 97)
= 15 \(\times\) 100
=1500
b, \(\dfrac{2016}{2015}\) \(\times\) \(\dfrac{4}{7}\) - \(\dfrac{4}{7}\) \(\times\) \(\dfrac{1}{2015}\) + \(\dfrac{3}{7}\)
= \(\dfrac{4}{7}\) \(\times\) ( \(\dfrac{2016}{2015}\) - \(\dfrac{1}{2015}\)) + \(\dfrac{3}{7}\)
= \(\dfrac{4}{7}\) \(\times\) \(\dfrac{2015}{2015}\) + \(\dfrac{3}{7}\)
= \(\dfrac{4}{7}\) + \(\dfrac{3}{7}\)
= \(\dfrac{7}{7}\)
= 1
PT
1
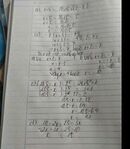
\(\left(x+2015\right)^7=\left(x+2015\right)^5\)
\(\Rightarrow\left(x+2015\right)\in\left\{0;1;-1\right\}\)
\(\Rightarrow x\in\left\{-2015;-2014;-2016\right\}\)
Vậy \(x\in\left\{-2015;-2014;-2016\right\}\)
\(\left(x+2015\right)^7=\left(x+2015\right)^5\)
\(\Leftrightarrow\left(x+2015\right)^7-\left(x+2015\right)^5=0\)
\(\Leftrightarrow\left(x+2015\right)^5\left[\left(x+2015\right)^2-1\right]=0\)
\(\Leftrightarrow\left(x+2015\right)^5\left(x+2014\right)\left(x+2016\right)=0\)
\(\Leftrightarrow x=-2016;x=-2015;x=-2014\)