A= X2-6x+11
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Trả lời:
a, \(x^2-6x+11=x^2-6x+9+2=\left(x-3\right)^2+2\ge2\forall x\)
Dấu "=" xảy ra khi x - 3 = 0 <=> x = 3
Vậy GTNN của biểu thức bằng 2 khi x = 3
b, \(-x^2+6x-11=-\left(x^2-6x+11\right)=-\left(x^2-6x+9+2\right)=-\left[\left(x-3\right)^2+2\right]\)
\(=-\left(x-3\right)^2-2\le-2\forall x\)
Dấu "=" xảy ra khi x - 3 = 0 <=> x = 3
Vậy GTLN của biểu thức bằng - 2 khi x = 3
c, \(x^2+2x+2=x^2+2x+1+1=\left(x+1\right)^2+1\ge1>0\forall x\inℤ\) (đpcm)
Dấu "=" xảy ra khi x + 1 = 0 <=> x = - 1

√(x2-6x+11) + √(x2-6x+13) + √(x2-4x+5) = 3+√2 (1)
Có: \(\sqrt{x^2-6x+11}=\sqrt{\left(x-3\right)^2+2}\ge\sqrt{2}\)
(Dấu = xảy ra khi x = 3)
\(\sqrt{x^2-6x+13}=\sqrt{\left(x-3\right)^2+4}\ge\sqrt{4}=2\)
(Dấu = xảy ra khi x = 3)
\(\sqrt{x^2-4x+5}=\sqrt{\left(x-2\right)^2+1}\ge1\)
(Dấu = xảy ra khi x = 2)
Nhận xét PT (1):
\(VT\ge3+\sqrt{2}\)
\(VP=3+\sqrt{2}\)
Nên: √(x2-6x+11) + √(x2-6x+13) + √(x2-4x+5) = 3+√2 khi: x = 3 và x = 2
=> PT vô nghiệm

a:
ĐKXĐ: \(x^2+3x>=0\)
=>x(x+3)>=0
=>\(\left[{}\begin{matrix}x>=0\\x< =-3\end{matrix}\right.\)
\(\sqrt{16}-\sqrt{x^2+3x}=0\)
=>\(\sqrt{x^2+3x}=\sqrt{16}\)
=>x^2+3x=16
=>x^2+3x-16=0
\(\text{Δ}=3^2-4\cdot1\cdot\left(-16\right)=9+64=73>0\)
Do đó: Phương trình có 2 nghiệm phân biệt là:
\(\left\{{}\begin{matrix}x_1=\dfrac{-3-\sqrt{73}}{2}\\x_2=\dfrac{-3+\sqrt{73}}{2}\end{matrix}\right.\)
b:
ĐKXĐ: \(x\in R\)
\(3x-1-\sqrt{4x^2-12x+9}=0\)
=>\(\sqrt{\left(2x-3\right)^2}=3x-1\)
=>\(\left\{{}\begin{matrix}3x-1>=0\\\left(3x-1\right)^2=\left(2x-3\right)^2\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x>=\dfrac{1}{3}\\\left(3x-1-2x+3\right)\left(3x-1+2x-3\right)=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x>=\dfrac{1}{3}\\\left(x+2\right)\left(5x-4\right)=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-2\left(loại\right)\\x=\dfrac{4}{5}\left(nhận\right)\end{matrix}\right.\)
c:
ĐKXĐ: \(\left\{{}\begin{matrix}x^2-6x+8>=0\\2x^2-10x+11>=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\left[{}\begin{matrix}x>=4\\x< =2\end{matrix}\right.\\\left[{}\begin{matrix}x< =\dfrac{5-\sqrt{3}}{2}\\x>=\dfrac{5+\sqrt{3}}{2}\end{matrix}\right.\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}x< =\dfrac{5-\sqrt{3}}{2}\\x>=4\end{matrix}\right.\)
\(\sqrt{2x^2-10x+11}=\sqrt{x^2-6x+8}\)
\(\Leftrightarrow2x^2-10x+11=x^2-6x+8\)
=>\(x^2-4x+3=0\)
=>(x-1)(x-3)=0
=>x=3(loại) hoặc x=1(nhận)

\(A=\left(x^2-6x+9\right)+2=\left(x-3\right)^2+2\ge2\\ A_{min}=2\Leftrightarrow x=3\\ B=2\left(x^2-10x+25\right)+51=2\left(x-5\right)^2+51\ge51\\ B_{min}=51\Leftrightarrow x=5\\ C=\left[\left(x^2-4xy+4y^2\right)+10\left(x-2y\right)+25\right]+\left(y^2-2y+1\right)+2\\ C=\left(x-2y+5\right)^2+\left(y-1\right)^2+2\ge2\\ C_{min}=2\Leftrightarrow\left\{{}\begin{matrix}x-2y+5=0\\y-1=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=2y-5=2-5=-3\\y=1\end{matrix}\right.\)
a) \(A=\left(x^2-6x+9\right)+2=\left(x-3\right)^2+2\ge2\)
\(minA=2\Leftrightarrow x=3\)
b) \(B=2\left(x^2-10x+25\right)+51=2\left(x-5\right)^2+51\ge51\)
\(minB=51\Leftrightarrow x=5\)
c) \(C=\left[x^2-2x\left(2y-5\right)+\left(2y-5\right)^2\right]+\left(y^2-2y+1\right)+2=\left(x-2y+5\right)^2+\left(y-1\right)^2+2\ge2\)
\(minC=2\Leftrightarrow\left\{{}\begin{matrix}x=-3\\y=1\end{matrix}\right.\)

Đề là gì bạn? Lần sau bạn đăng câu hỏi nhớ ghi kèm cái đề nhé!

Điều kiện: 1 ≤ x ≤ 3
bpt 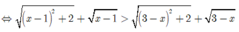
Xét
f ( t ) = t 2 + 2 + t , t ≥ 0 f ' ( t ) = t 2 t 2 + 2 + 1 2 t , ∀ t > 0
Do đó hàm số đồng biến trên [ 0 ; + ∞ ) .
Từ (1) suy ra f(x-1) >f(3-x) hay x-1> 3-x
Suy ra : x> 2
So với điều kiện, bpt có tập nghiệm là S= (2; 3]
Do đó; a=2; b=3 và b-a=1
Chọn A.

Đáp án A
Điều kiện: 1 ≤ x ≤ 3 ; b p t ⇔ x − 1 2 + 2 + x − 1 > 3 − x 2 + 2 + 3 − x
Xét f t = t 2 + 2 + t với t ≥ 0 . Có f ' t = t 2 t 2 + 2 + 1 2 t > 0 , ∀ t > 0
Do đó hàm số đồng biến trên 0 ; + ∞ . 1 ⇔ f x − 1 > f 3 − x ⇔ x − 1 > 3 ⇔ x > 2
So với điều kiện, bpt có tập nghiệm là S = 2 ; 3


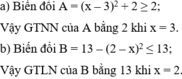

\(A=x^2-6x+9+2\)
\(A=\left(x^2-6x+9\right)+2\)
\(A=\left(x-3\right)^2+2\)
\(A=x^2-6x+11\) <=> \(A=x^2-6x+3^2+2\)
<=> \(A=\left(x-3\right)^2+2>2\)
=> đa thức sau vô nghiệm