
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



a) \(\sqrt{4x}+\sqrt{\dfrac{x}{4}}+\dfrac{1}{2}\sqrt{49x}=6\left(x\ge0\right)\)
\(\Rightarrow2\sqrt{x}+\dfrac{1}{2}\sqrt{x}+\dfrac{7}{2}\sqrt{x}=6\Rightarrow6\sqrt{x}=6\Rightarrow\sqrt{x}=1\Rightarrow x=1\)
b) ĐKXĐ: \(x\ge\dfrac{1}{2}\)
\(\sqrt{18x-9}-0,5\sqrt{2x-1}+\dfrac{1}{2}\sqrt{25\left(2x-1\right)}+\sqrt{49\left(2x-1\right)}=24\)
\(\Rightarrow\sqrt{9\left(2x-1\right)}-0,5\sqrt{2x-1}+\dfrac{5}{2}\sqrt{2x-1}+7\sqrt{2x-1}=24\)
\(\Rightarrow3\sqrt{2x-1}-0,5\sqrt{2x-1}+\dfrac{5}{2}\sqrt{2x-1}+7\sqrt{2x-1}=24\)
\(\Rightarrow12\sqrt{2x-1}=24\Rightarrow\sqrt{2x-1}=2\Rightarrow2x-1=4\Rightarrow x=\dfrac{5}{2}\)
c) \(\sqrt{x^2-2x+1}-7=0\Rightarrow\sqrt{\left(x-1\right)^2}=7\Rightarrow\left|x-1\right|=7\)
\(\Rightarrow\left[{}\begin{matrix}x-1=7\\x-1=-7\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=8\\x=-6\end{matrix}\right.\)
d) \(\dfrac{1}{2}\sqrt{\dfrac{49x}{x+2}}-3\sqrt{\dfrac{x}{4x+8}}-2\sqrt{\dfrac{x}{x+2}}-\sqrt{5}=0\left(\dfrac{x}{x+2}\ge0,x\ne-2\right)\)
\(\Rightarrow\dfrac{7}{2}\sqrt{\dfrac{x}{x+2}}-3\sqrt{\dfrac{x}{4\left(x+2\right)}}-2\sqrt{\dfrac{x}{x+2}}=\sqrt{5}\)
\(\Rightarrow\dfrac{7}{2}\sqrt{\dfrac{x}{x+2}}-\dfrac{3}{2}\sqrt{\dfrac{x}{x+2}}-2\sqrt{\dfrac{x}{x+2}}=\sqrt{5}\)
\(\Rightarrow0=\sqrt{5}\) (vô lý) \(\Rightarrow\) pt vô nghiệm
a) \(\sqrt{4x}+\sqrt{\dfrac{x}{4}}+\dfrac{1}{2}\sqrt{49x}=6\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge0\\2\sqrt{x}+\dfrac{\sqrt{x}}{2}+\dfrac{7}{2}\sqrt{x}=6\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge0\\\sqrt{x}\left(2+\dfrac{1}{2}+\dfrac{7}{2}\right)=6\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge0\\6\sqrt{x}=6\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x\ge0\\\sqrt{x}=1\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x\ge0\\x=1\end{matrix}\right.\) \(\Leftrightarrow x=1\)
Vậy \(S=\left\{1\right\}\)
b) \(\sqrt{18x-9}-0.5\sqrt{2x-1}+\dfrac{1}{2}\sqrt{25\left(2x-1\right)}+\sqrt{49\left(2x-1\right)}=24\)
\(\Leftrightarrow3\sqrt{2x-1}-0,5\sqrt{2x-1}+\dfrac{5}{2}\sqrt{2x-1}+7\sqrt{2x-1}=24\)
\(\Leftrightarrow\left\{{}\begin{matrix}2x-1\ge0\\\sqrt{2x-1}\left(3-0.5+\dfrac{5}{2}+7\right)=49\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge\dfrac{1}{2}\\12\sqrt{2x-1}=24\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x\ge\dfrac{1}{2}\\\sqrt{2x-1}=2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ge\dfrac{1}{2}\\2x-1=4\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x\ge\dfrac{1}{2}\\x=\dfrac{5}{2}\end{matrix}\right.\) \(\Leftrightarrow x=\dfrac{5}{2}\)
Vậy \(S=\left\{\dfrac{5}{2}\right\}\)
c) \(\sqrt{x^2-2x+1}-7=0\) (*)
Ta có \(x^2-2x+1=\left(x-1\right)^2\ge0\forall x\) \(\Rightarrow\sqrt{x^2-2x+1}\ge0\forall x\)
(*) \(\Leftrightarrow\sqrt{\left(x-1\right)^2}-7=0\)
\(\Leftrightarrow\left|x-1\right|-7=0\)
\(\Leftrightarrow x-1-7=0\)
\(\Leftrightarrow x=8\)
Vậy \(S=\left\{8\right\}\)
\(\)d) \(\dfrac{1}{2}\sqrt{\dfrac{49x}{x+2}}-3\sqrt{\dfrac{x}{4x+8}}-2\sqrt{\dfrac{x}{x+2}}-\sqrt{5}=0\) (**)
\(\Leftrightarrow\dfrac{7}{2}\sqrt{\dfrac{x}{x+2}}-\dfrac{3}{2}\sqrt{\dfrac{x}{x+2}}-2\sqrt{\dfrac{x}{x+2}}=\sqrt{5}\)
ĐKXĐ: \(\dfrac{x}{x+2}\ge0\) \(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x\ge0\\x+2>0\end{matrix}\right.\\\left\{{}\begin{matrix}x\le0\\x+2< 0\end{matrix}\right.\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x\ge0\\x>-2\end{matrix}\right.\\\left\{{}\begin{matrix}x\le0\\x< -2\end{matrix}\right.\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}x\ge0\\x< -2\end{matrix}\right.\)
(**) \(\Leftrightarrow\sqrt{\dfrac{x}{x+2}}\left(\dfrac{7}{2}-\dfrac{3}{2}-2\right)=\sqrt{5}\)
\(\Leftrightarrow0\sqrt{\dfrac{x}{x+2}}=\sqrt{5}\)
\(\Leftrightarrow0=\sqrt{5}\) ( vô lý )
Vậy phương trình trên vô nghiệm

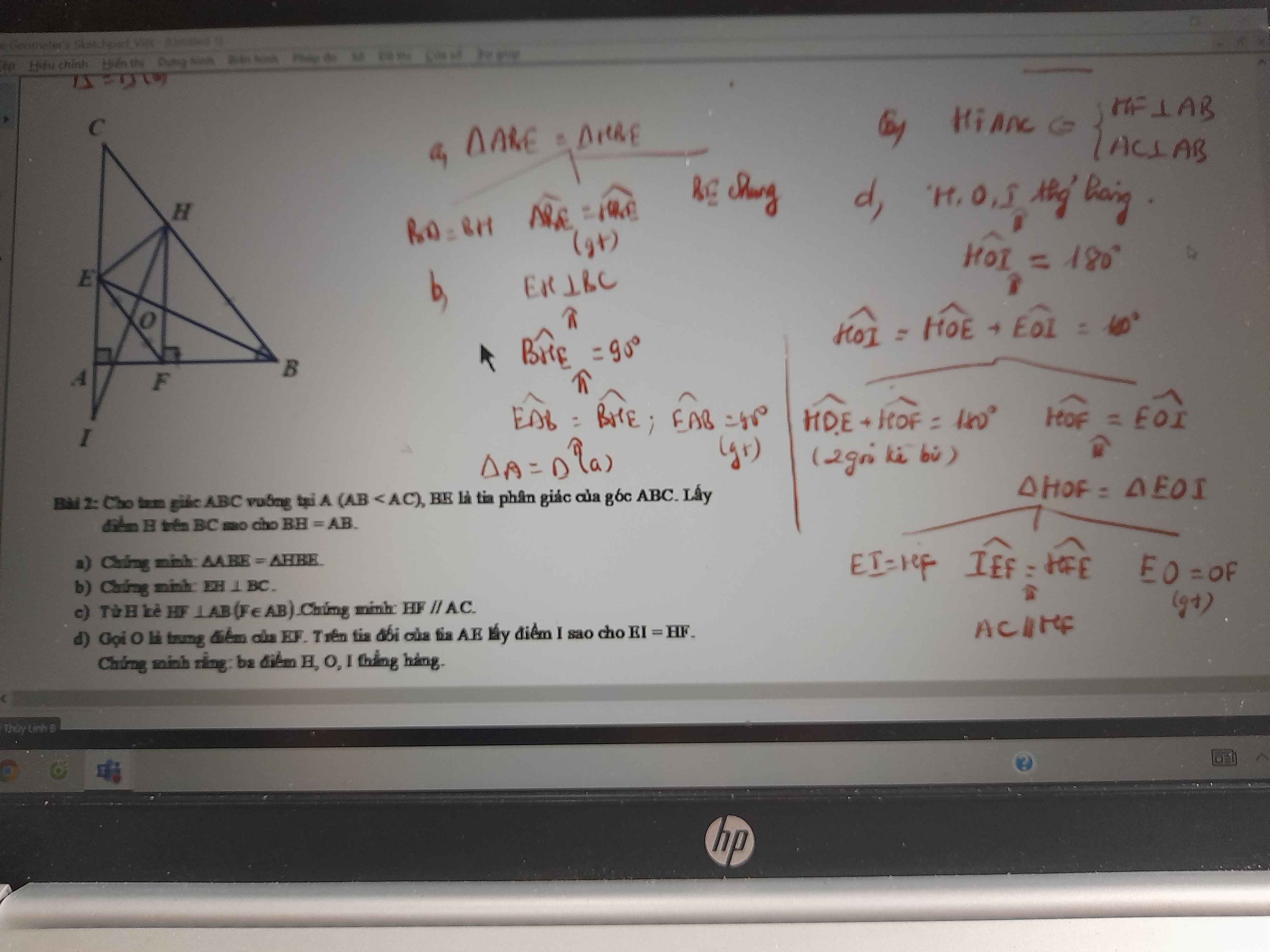
a: Xét ΔABE và ΔHBE có
BA=BH
\(\widehat{ABE}=\widehat{HBE}\)
BE chung
Do đó: ΔABE=ΔHBE
b: Ta có: ΔABE=ΔHBE
nên \(\widehat{BAE}=\widehat{BHE}=90^0\)
hay HE\(\perp\)BC

Bài 2:
Bài giải
Người ta xếp 200 cái bánh vào mỗi hộp mà trong mỗi hộp có 5 cái, vậy xếp được số hộp là:
200 : 5 = 40 ( hộp bánh )
Có số thùng bánh là:
40 : 8 = 5 ( thùng bánh )
Đáp số: 5 thùng bánh.

khuyến cáo ko nên gạt xuống.
Đồ ngu đồ ăn hại cút mịa mài đê :D

Gọi số đó là abc (số có 3 chữ số)
Vì abc là bội của 18 nên abc phải chia hết cho 18
=> abc phải chia hết cho 9
mà 1 <= a+b+c <= 27 (do a,b,c nhận các giá trị tự nhiên từ 1 đến 9 )
=> a+b+c nhận 1 trong 3 số 9; 18; 27 (1)
Vì các chữ số của nó lần lượt tỉ lệ theo 1:2:3
=> a:b:c = 1:2:3
\(\Rightarrow\dfrac{a}{1}=\dfrac{b}{c}=\dfrac{c}{3}\)
Áp dụng tính chất của dãy tỉ số bằng nhau ta có
\(\dfrac{a}{1}=\dfrac{b}{2}=\dfrac{c}{3}=\dfrac{a+b+c}{1+2+3}=\dfrac{a+b+c}{6}\left(2\right)\)
Từ (1) và (2) suy ra (a+b+c) = 18 (chia hết cho 6)
\(\Rightarrow\dfrac{a}{1}=\dfrac{b}{2}=\dfrac{c}{3}=\dfrac{a+b+c}{6}=\dfrac{18}{6}=3\)
\(\Rightarrow\dfrac{a}{1}=3\Rightarrow a=3\)
\(\dfrac{b}{2}=3\Rightarrow b=6\)
\(\dfrac{c}{3}=3\Rightarrow c=9\)
Nhưng vì số đó chia hết cho 18 nên chữ số hàng đơn vị phải là số chẵn
=> Chữ số hàng đơn vị là 6
Vậy ta có 2 chữ số thoả mãn là 396 và 936

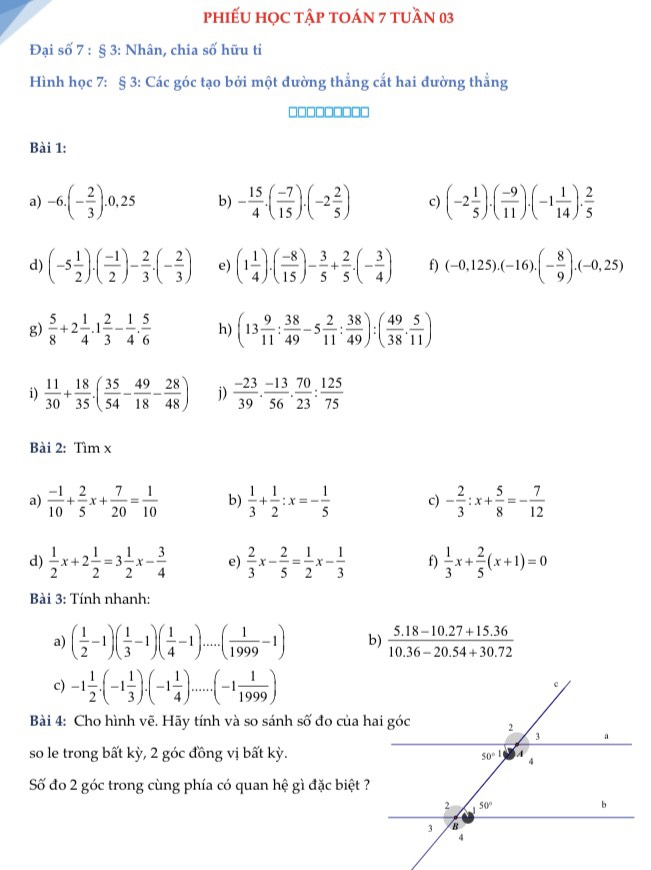
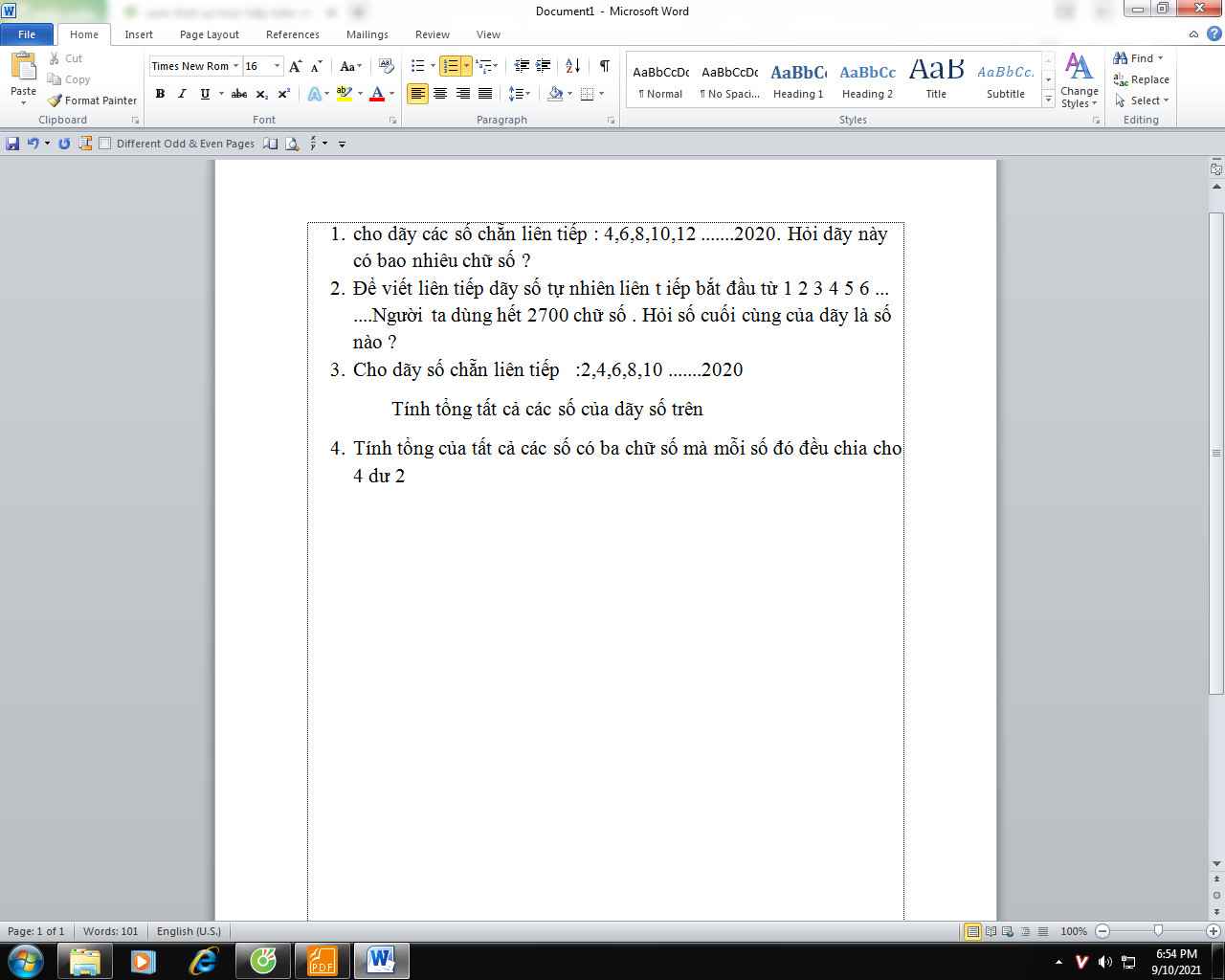



 giúp mik làm bài 2, 3 đi
giúp mik làm bài 2, 3 đi




bài 5
Nhân phá tung ra ta có :
\(3x^2+2x+x^2+2x+1-4x^2+25=-12\Leftrightarrow4x=-38\Leftrightarrow x=-\frac{19}{2}\)
bài 6.
ta có : \(2n^2+5n+2-3=\left(2n+1\right)\left(n+2\right)-3\text{ chia hết cho }2n+1\)
Khi 3 chia hết cho 2n+1 hay \(2n+1\in\left\{\pm1,\pm3\right\}\Rightarrow n\in\left\{-2,-1,0,1\right\}\)
Bài 5.
\(x\left(3x+2\right)+\left(x+1\right)^2-\left(2x-5\right)\left(2x+5\right)=-12\)
\(\Leftrightarrow3x^2+2x+x^2+2x+1-4x^2+25=-12\)
\(\Leftrightarrow4x=-38\)
\(\Leftrightarrow x=-\frac{38}{5}\).
Bài 6.
\(\left(2n^2+5n-1\right)⋮\left(2n-1\right)\Leftrightarrow\left(4n^2+10n-2\right)⋮\left(2n-1\right)\)(1)
Có \(4n^2+10n-2=4n^2-4n+1+14n-7+4=\left(2n-1\right)^2+7\left(2n-1\right)+4\)
Do đó (1) tương đương với \(4⋮\left(2n-1\right)\Leftrightarrow2n-1\in\left\{-1,1\right\}\)(vì \(2n-1\)là số lẻ)
\(\Leftrightarrow n\in\left\{0,1\right\}\).