K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
TD
1
29 tháng 9 2015
Đặt \(\sqrt[3]{7-x}=a;\sqrt[3]{5-x}=b\) ( a + b \(\ne\) 0)
=> a3 + b3 = 12 - 2x = 2(6 - x) ; a3 - b3 = 2
PT <=> \(\frac{a-b}{a+b}=\frac{a^3+b^3}{2}\) <=> (a3 + b3)(a+ b) = 2(a - b)
Thế 2 = a3 - b3 ta được:
(a3 + b3)(a+ b) = (a3 - b3)(a - b)
<=> a4 + a3b + ab3 + b4 = a4 - a3b - ab3 + b4
<=> a3b + ab3 = - a3b - ab3
<=> 2(a3b + ab3) = 0 <=> ab.(a2+ b2) = 0 <=> ab = 0 hoặc a2 + b2 = 0
+) ab = 0 => a = 0 hoặc b = 0
Nếu a = 0 thì b3 = - 2 => \(b=-\sqrt[3]{2}\)
Nếu b = 0 thì a3 = 2 => \(a=\sqrt[3]{2}\)
+) a2 + b2 = 0 => a = b = 0 => Loại (vì a + b khác 0)
Vậy a = 0 hoặc b = 0
a = 0 => x = 7
b = 0 => x = 5
Vậy...........
17 tháng 2 2020
Ta có: \(x\left(x+7\right)=-6\)
\(\Leftrightarrow x;x+7\inƯ\left(-6\right)\)
\(\Leftrightarrow x;x+7\in\left\{1;-1;2;-2;3;-3;6;-6\right\}\)
*Trường hợp 1:
\(\left\{{}\begin{matrix}x=1\\x+7=-6\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\x=-13\end{matrix}\right.\)
*Trường hợp 2:
\(\left\{{}\begin{matrix}x=-6\\x+7=1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-6\\x=-6\end{matrix}\right.\)
*Trường hợp 3:
\(\left\{{}\begin{matrix}x=-1\\x+7=6\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-1\\x=-1\end{matrix}\right.\)
*Trường hợp 4:
\(\left\{{}\begin{matrix}x=6\\x+7=-1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=6\\x=-6\end{matrix}\right.\)
*Trường hợp 5:
\(\left\{{}\begin{matrix}x=2\\x+7=-3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=2\\x=-10\end{matrix}\right.\)
*Trường hợp 6:
\(\left\{{}\begin{matrix}x=-3\\x+7=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-3\\x=-5\end{matrix}\right.\)
*Trường hợp 7:
\(\left\{{}\begin{matrix}x=-2\\x+7=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-2\\x=-4\end{matrix}\right.\)
*Trường hợp 8:
\(\left\{{}\begin{matrix}x=3\\x+7=-2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=3\\x=-9\end{matrix}\right.\)
Vậy: \(x\in\left\{1;-13;-6;-1;6;2;-10;-3;-5;-2;-4;3;-9\right\}\)
15 tháng 4 2020
\(ĐKXĐ:x\ne5,8\)
\(\frac{6}{x-5}+\frac{x+2}{x-8}=\frac{18}{\left(x-5\right)\left(8-x\right)}-1\)
\(\Rightarrow\frac{6}{x-5}+\frac{x+2}{x-8}=-\frac{18}{\left(x-5\right)\left(x-8\right)}-1\)
\(\Rightarrow6\left(x-8\right)+\left(x+2\right)\left(x-5\right)=-18-\left(x-5\right)\left(x-8\right)\)
\(\Rightarrow x^2+3x-58=-x^2+13x-58\)
\(\Rightarrow2x^2-10x=0\)
\(\Rightarrow2x\left(x-5\right)=0\)
\(\Rightarrow x\in\left\{0,5\right\}\)
GK
1
14 tháng 9 2023
\(a,\dfrac{2x-1}{3}< \dfrac{x+6}{2}\)
\(\Leftrightarrow\dfrac{4x-2}{6}< \dfrac{3x+18}{6}\)
\(\Leftrightarrow4x-2< 3x+18\)
\(\Leftrightarrow4x-3x< 2+18\)
\(\Leftrightarrow x< 20\)
\(b,\dfrac{5\left(x-1\right)}{6}-1>\dfrac{2\left(x+1\right)}{3}\)
\(\Leftrightarrow\dfrac{5x-11}{6}>\dfrac{4x+4}{6}\)
\(\Leftrightarrow5x-11>4x+4\)
\(\Leftrightarrow5x-4x>11+4\)
\(\Leftrightarrow x>15\)
NV
Nguyễn Việt Lâm
Giáo viên
5 tháng 5 2021
ĐKXĐ: \(0\le x\le5\)
Pt tương đương:
\(\sqrt{x+3}+4\sqrt{x}+\sqrt{5-x}=2x+6\)
Ta có:
\(VT=\dfrac{1}{2}.2.\sqrt{x+3}+4.1.\sqrt{x}+\dfrac{1}{2}.2.\sqrt{5-x}\)
\(VT\le\dfrac{1}{4}\left(4+x+3\right)+2\left(1+x\right)+\dfrac{1}{4}\left(4+5-x\right)\)
\(\Rightarrow VT\le2x+6=VP\)
Dấu "=" xảy ra khi và chỉ khi:
\(\left\{{}\begin{matrix}\sqrt{x+3}=2\\\sqrt{x}=1\\\sqrt{5-x}=2\end{matrix}\right.\) \(\Leftrightarrow x=1\)
9 tháng 11 2022
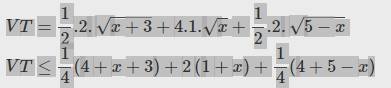
Từ bước trên xuống bước dưới áp dụng công thức nào vậy thầy - Nhờ thầy chỉ rõ hơn.
Trân trọng!
BC
0
BC
0
Cho tam giác ABC vuông ở A đường cao AH,qua B kẻ đường thẳng song song với AH cắt AC tại K,kẻ AM vuông góc với BK tại M,lấy I là trung điểm của KC, gọi E là giao điểm của BI và MH, chứng minh cos^3K.sin k = EH/KC