K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NN
0
20 tháng 3 2017
\(2x^2-4x+5=2\left(x^2-2x+\frac{5}{2}\right)=2\left[\left(x^2-2x+1\right)+\frac{3}{2}\right]=2\left[\left(x-1\right)^2+\frac{3}{2}\right]=2\left(x-1\right)^2+3\ge3\)
\(\Rightarrow x\in\phi\)
NT
0
NT
2
1 tháng 5 2015
f(x) = 0 => x3 - 2x2 - x + 2 = 0
=> x2. (x - 2) - (x - 2) = 0
=> (x2 - 1).(x - 2) = 0 => x2 - 1 = 0 hoặc x - 2 = 0
+) x2 - 1 = 0 => x = 1 hoặc x = -1
+) x - 2 = 0 => x = 2
Vậy đa thức có 3 nghiệm là: -1;1;2
LT
0
NT
4
5 tháng 5 2019
Để đa thức có nghiệm thì \(x^2-2x=0\)
\(\Rightarrow x\left(x-2\right)=0\)
\(\Rightarrow\orbr{\begin{cases}x=0\\x-2=0\end{cases}}\)
\(\Rightarrow\orbr{\begin{cases}x=0\\x=2\end{cases}}\)
=.= hk tốt!!
KT
6 tháng 8 2018
\(P\left(x\right)=2x^3+4x^2-5x-1=0\)
<=> \(2x^3-2x^2+6x^2-6x+x-1=0\)
<=> \(2x^2\left(x-1\right)+6x\left(x-1\right)+x-1=0\)
<=> \(\left(x-1\right)\left(2x^2+6x+1\right)=0\)
<=> \(x-1=0\) (do 2x2 + 6x + 1 khác 0)
<=> \(x=1\)
Vậy....
6 tháng 8 2018
\(P\left(x\right)=2x^3+4x^2-5x-1\)
\(P\left(x\right)=2x^3-2x^2+6x^2-6x+x-1\)
\(P\left(x\right)=2x^2\left(x-1\right)-6x\left(x-1\right)+\left(x-1\right)\)
\(P\left(x\right)=\left(x-1\right)\left(2x^2-6x+1\right)\)
Để P(x) có nghiệm \(\Rightarrow x-1=0\Leftrightarrow x=1\)
Vậy x = 1 là 1 nghiệm của P(x)
AH
Akai Haruma
Giáo viên
22 tháng 4 2021
Lời giải:
$M(x)=(6+4x)(-x+2)=0$
\(\Leftrightarrow \left[\begin{matrix} 6+4x=0\\ -x+2=0\end{matrix}\right.\Leftrightarrow \left[\begin{matrix} x=-\frac{3}{2}\\ x=2\end{matrix}\right.\)
Vậy nghiệm của đa thức $M(x)$ là $x=\frac{-3}{2}$ và $x=2$
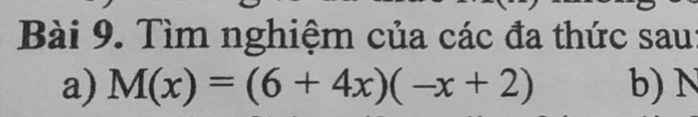
\(3x^3+4x^2+2x+1=0\)
\(\Leftrightarrow\left(3x^3+x^2+x\right)+\left(3x^2+x+1\right)=0\)
\(\Leftrightarrow x\left(3x^2+x+1\right)+1\left(3x^2+x+1\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(3x^2+x+1\right)=0\)
Ta có:\(3x^2+x+1=3\left(x^2+x.\frac{1}{3}+\frac{1}{3}\right)\)
\(=3\left(x^2+2.x.\frac{1}{6}+\frac{1}{36}-\frac{1}{36}+\frac{1}{3}\right)\)
\(=3\left[\left(x+\frac{1}{6}\right)^2+\frac{11}{36}\right]\ge3.\frac{11}{36}=\frac{11}{12}>0\forall x\)
Do đó x + 1 = 0 tức là x = -1
\(3x^3+3x^2+x^2+x+x+1=0\)
\(3x^2.\left(x+1\right)+x.\left(x+1\right)+\left(x+1\right)=0\)
\(\left(x+1\right).\left(3x^2+x+1\right)=0\)
+)\(3x^2+x+1=0\Leftrightarrow3.\left(x^2+x+\frac{1}{3}\right)=0\Leftrightarrow3.\left(x+\frac{1}{6}\right)^2+\frac{11}{12}=0\left(loai\right)\)
+) x+1=0 <=> x=-1