AP
2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
11 tháng 1 2022
a: \(\Leftrightarrow\left(2m-4\right)^2-4\left(m^2-3\right)>=0\)
\(\Leftrightarrow4m^2-16m+16-4m^2+12>=0\)
=>-16m>=-28
hay m<=7/4
b: \(\Leftrightarrow16m^2-4\left(2m-1\right)\left(2m+3\right)=0\)
\(\Leftrightarrow16m^2-4\left(4m^2+4m-3\right)=0\)
=>4m-3=0
hay m=3/4
c: \(\Leftrightarrow\left(4m-2\right)^2-4\cdot4\cdot m^2< 0\)
=>-16m+4<0
hay m>1/4
NV
Nguyễn Việt Lâm
Giáo viên
22 tháng 4 2021
Ta có:
\(a-b+c=4-\left(m^2+2m-15\right)+\left(m+1\right)^2-20\)
\(=-m^2-2m+19+m^2+2m+1-20\)
\(=0\)
\(\Rightarrow\) Phương trình đã cho luôn luôn có 2 nghiệm: \(\left[{}\begin{matrix}x=-1\\x=\dfrac{20-\left(m+1\right)^2}{4}\end{matrix}\right.\)
TH1: \(\left\{{}\begin{matrix}x_1=-1\\x_2=5-\dfrac{\left(m+1\right)^2}{4}\end{matrix}\right.\)
\(\Rightarrow1+5-\dfrac{\left(m+1\right)^2}{4}+2019=0\)
\(\Leftrightarrow\left(m+1\right)^2=8100\Rightarrow\left[{}\begin{matrix}m+1=90\\m+1=-90\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}m=89\\m=-91\end{matrix}\right.\)
TH2: \(\left\{{}\begin{matrix}x_1=5-\dfrac{\left(m+1\right)^2}{4}\\x_2=-1\end{matrix}\right.\)
\(\Rightarrow\left[5-\dfrac{\left(m+1\right)^2}{4}\right]^2-1+2019=0\)
\(\Leftrightarrow\left[5-\dfrac{\left(m+1\right)^2}{4}\right]^2+2018=0\) (vô nghiệm do vế trái luôn dương)
Vậy \(\left[{}\begin{matrix}m=89\\m=-91\end{matrix}\right.\)
30 tháng 7 2021
b) Thay x=2 vào pt, ta được:
\(4\left(m^2-1\right)-4m+m^2+m+4=0\)
\(\Leftrightarrow4m^2-4-4m+m^2+m+4=0\)
\(\Leftrightarrow5m^2-3m=0\)
\(\Leftrightarrow m\left(5m-3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m=0\\m=\dfrac{3}{5}\end{matrix}\right.\)
Áp dụng hệ thức Vi-et, ta được:
\(x_1+x_2=\dfrac{2m}{m^2-1}\)
\(\Leftrightarrow\left[{}\begin{matrix}x_2+2=0\\x_2+2=\dfrac{6}{5}:\left(\dfrac{36}{25}-1\right)=\dfrac{30}{11}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x_2=-2\\x_2=\dfrac{8}{11}\end{matrix}\right.\)
5 tháng 1 2022
a: \(\Leftrightarrow\left(2m+1\right)^2-4\left(m^2-3\right)=0\)
\(\Leftrightarrow4m^2+4m+1-4m^2+12=0\)
=>4m=-13
hay m=-13/4
c: \(\Leftrightarrow\left(2m-2\right)^2-4m^2>=0\)
\(\Leftrightarrow4m^2-8m+4-4m^2>=0\)
=>-8m>=-4
hay m<=1/2
26 tháng 12 2021
\(a,\Leftrightarrow\Delta'\ge0\\ \Leftrightarrow\left(m+2\right)^2-\left(m^2-4\right)\ge0\\ \Leftrightarrow m^2+4m+4-m^2+4\ge0\\ \Leftrightarrow4m+8\ge0\\ \Leftrightarrow m\ge-2\\ b,\Leftrightarrow\Delta'=0\Leftrightarrow m=-2\)
HP
12 tháng 8 2021
b) phương trình có 2 nghiệm \(\Leftrightarrow\Delta'\ge0\)
\(\Leftrightarrow\left(m-1\right)^2-\left(m-1\right)\left(m+3\right)\ge0\)
\(\Leftrightarrow m^2-2m+1-m^2-3m+m+3\ge0\)
\(\Leftrightarrow-4m+4\ge0\)
\(\Leftrightarrow m\le1\)
Ta có: \(x_1^2+x_1x_2+x_2^2=1\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=1\)
Theo viet: \(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=2\left(m-1\right)\\x_1x_2=\dfrac{c}{a}=m+3\end{matrix}\right.\)
\(\Leftrightarrow\left[-2\left(m-1\right)^2\right]-2\left(m+3\right)=1\)
\(\Leftrightarrow4m^2-8m+4-2m-6-1=0\)
\(\Leftrightarrow4m^2-10m-3=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m_1=\dfrac{5+\sqrt{37}}{4}\left(ktm\right)\\m_2=\dfrac{5-\sqrt{37}}{4}\left(tm\right)\end{matrix}\right.\Rightarrow m=\dfrac{5-\sqrt{37}}{4}\)
15 tháng 2 2022
b: Thay x=-5 vào pt, ta được:
\(m+25+65=0\)
hay m=-90
Theo đề, ta có: \(x_1+x_2=13\)
nên \(x_2=18\)
c: Thay x=-3 vào pt, ta được:
\(18+3\left(m+4\right)+m=0\)
=>4m+30=0
hay m=-15/2
Theo đề, ta có: \(x_1\cdot x_2=-\dfrac{m}{2}=\dfrac{15}{4}\)
hay \(x_2=-1.25\)
19 tháng 5 2020
a) PT có nghiệm kép nếu
\(\hept{\begin{cases}m-1\ne0\\\Delta'=\left(m-1\right)^2+m\left(m-1\right)=0\end{cases}\Leftrightarrow\hept{\begin{cases}m\ne1\\\left(m-1\right)\left(2m-1\right)=0\end{cases}\Leftrightarrow}m=\frac{1}{2}}\)
Vậy \(m=\frac{1}{2}\)thì pt có nghiệm kép
\(x_1=x_2=-\frac{b}{2a}=-\frac{2\left(m-1\right)}{2\left(m-1\right)}=-1\)
b) Để pt có nghiệm phân biệt đều âm thì
\(\hept{\begin{cases}m-1\ne0\\\Delta'=\left(m-1\right)\left(2m-1\right)>0\end{cases}}\)
\(\hept{\begin{cases}x_1\cdot x_2=-\frac{m}{m-1}>0\\x_1+x_2=\frac{2\left(m-1\right)}{m-1}< 0\end{cases}}\)
\(\Rightarrow\orbr{\begin{cases}m>1\\m< \frac{1}{2}\end{cases}}\)và \(0< m< 1\)
Vậy 0<m<\(\frac{1}{2}\)
PN
19 tháng 5 2020
định gõ ấn f5 cái thì thấy bạn làm xong r :((
giải nhanh quá !
24 tháng 6 2021
Để pt(1) có hai nghiệm trái dấu thì -(2m+1)<0
\(\Leftrightarrow2m+1>0\)
\(\Leftrightarrow2m>-1\)
hay \(m>-\dfrac{1}{2}\)
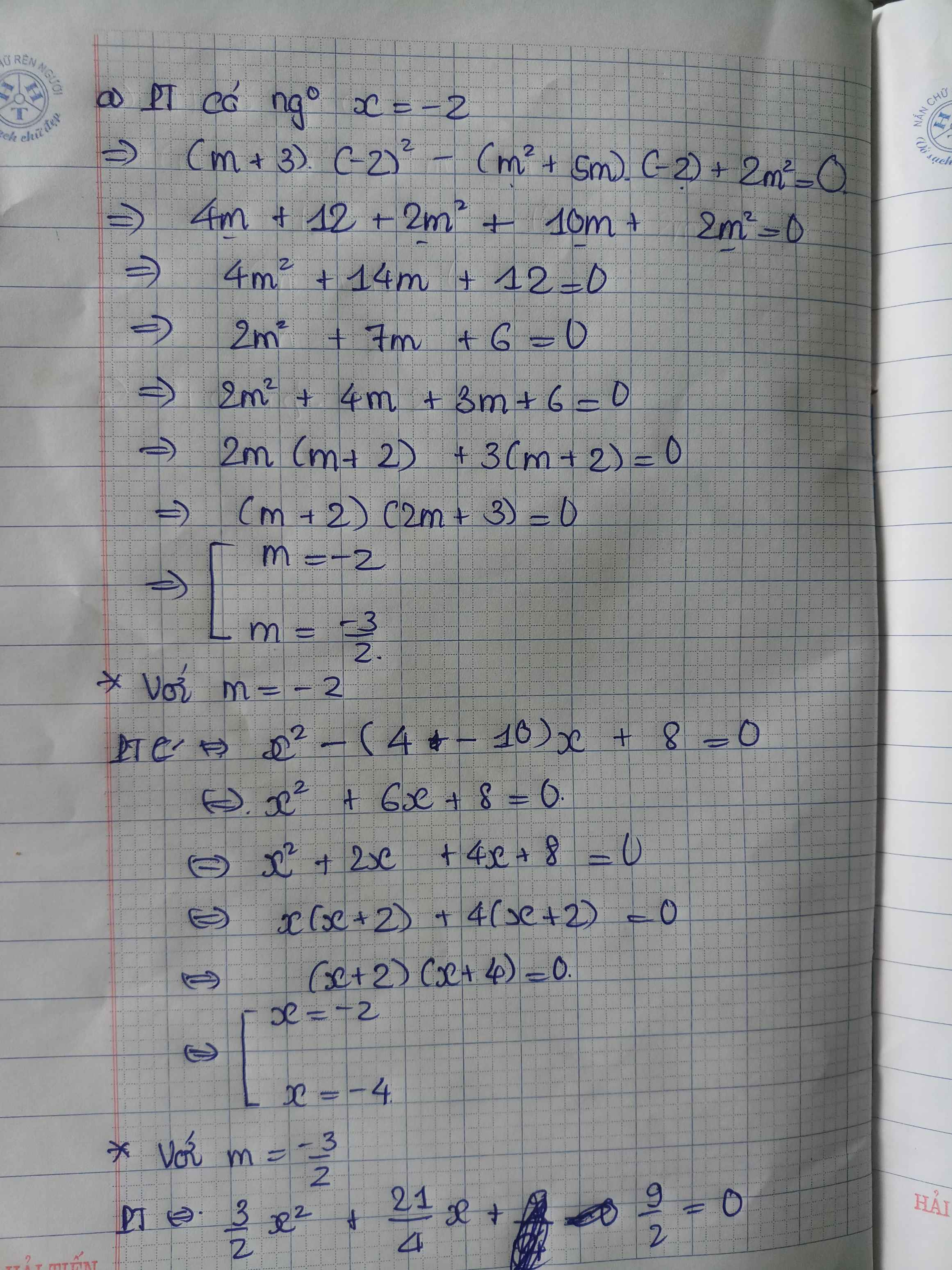
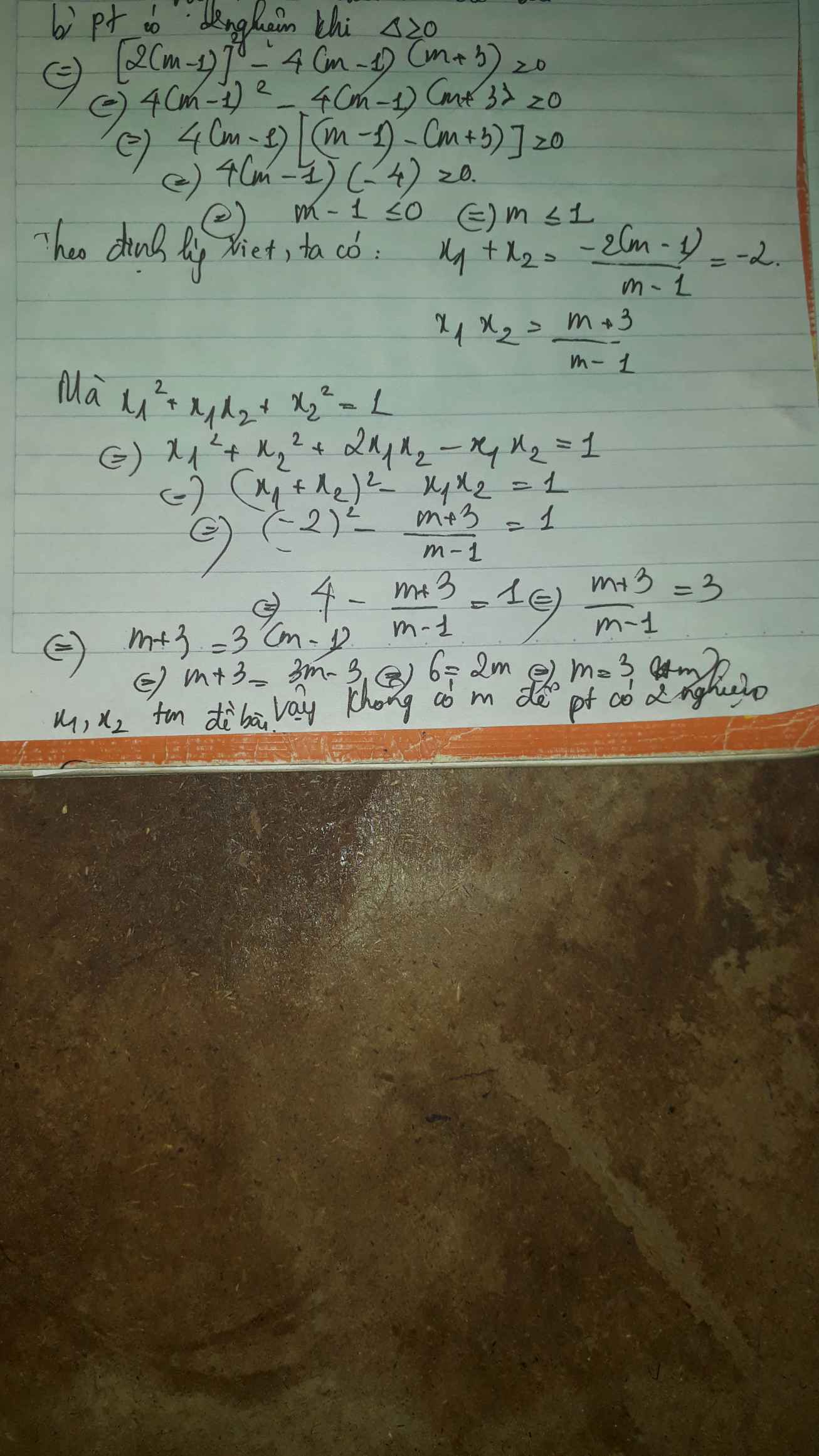
\(\Delta\ge0\Rightarrow-m^2+m+3\ge0\)
\(m^2-m-3\le0\Leftrightarrow\left(m-\dfrac{1-\sqrt{13}}{2}\right)\left(m-\dfrac{1+\sqrt{13}}{2}\right)\le0\)
\(\Rightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}m-\dfrac{1-\sqrt{13}}{2}\ge0\\m-\dfrac{1+\sqrt{13}}{2}\le0\end{matrix}\right.\\\left\{{}\begin{matrix}m-\dfrac{1-\sqrt{13}}{2}\le0\\m-\dfrac{1+\sqrt{13}}{2}\ge0\end{matrix}\right.\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}m\ge\dfrac{1-\sqrt{13}}{2}\\m\le\dfrac{1+\sqrt{13}}{2}\end{matrix}\right.\\\left\{{}\begin{matrix}m\le\dfrac{1-\sqrt{13}}{2}\\m\ge\dfrac{1+\sqrt{13}}{2}\end{matrix}\right.\end{matrix}\right.\)\(\Rightarrow\left[{}\begin{matrix}\dfrac{1-\sqrt{13}}{2}\le m\le\dfrac{1+\sqrt{13}}{2}\left(1\right)\\\dfrac{1+\sqrt{13}}{2}\le m\le\dfrac{1-\sqrt{13}}{2}\left(2\right)\end{matrix}\right.\)
(2) vô lý => chọn (1)
Vậy .... đc chưa :))))
De phuong trinh co 2 nghiem thi \(\Delta\ge0\)
\(4^2-4\left(m^2-m+1\right)\ge0\)
\(< =>16-4m^2+4m-4\ge0\)
\(< =>4m^2-4m-12\le0\)
\(< =>\left(2m-1\right)^2\le13\)
\(< =>-\sqrt{13}\le2m-1\le\sqrt{13}\)
\(< =>\dfrac{1-\sqrt{13}}{2}\le m\le\dfrac{\sqrt{13}+1}{2}\)