K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
KC
3
2 tháng 7 2017
Sorry nhá mk nhầm dấu + nên kq sai :
Ta có : (x + 3)(x - 11) + 2003
= x2 - 8x + 1970
= x2 - 8x + 16 + 1954
= (x - 4)2 + 1954
Mà (x - 4)2 \(\ge0\forall x\)
Nên : (x - 4)2 + 1954 \(\ge1954\forall x\)
Vậy GTNN của biểu thức là : 1954 khi và chỉ khi x = 4
2 tháng 7 2017
Ta có : (x + 3)(x - 11) + 2003
= x2 - 8x + 33 + 2003
= x2 - 8x + 2026
= x2 - 8x + 16 + 2010
= (x - 4)2 + 2010
Mà (x - 4)2 \(\ge0\forall x\)
Nên : (x - 4)2 + 2010 \(\ge2010\forall x\)
Vậy GTNN của biểu thức là : 2010 khi và chỉ khi x = 4
5 tháng 10 2021
\(A=\left(2x-1\right)^2+9\ge9\\ A_{min}=9\Leftrightarrow x=\dfrac{1}{2}\\ B=2\left(x^2-2\cdot\dfrac{3}{4}x+\dfrac{9}{16}\right)+\dfrac{1}{8}=2\left(x-\dfrac{3}{4}\right)^2+\dfrac{1}{8}\ge\dfrac{1}{8}\\ B_{min}=\dfrac{1}{8}\Leftrightarrow x=\dfrac{3}{4}\\ C=\left(4x^2+4xy+y^2\right)+2\left(2x+y\right)+1+\left(y^2+4y+4\right)-4\\ C=\left[\left(2x+y\right)^2+2\left(2x+y\right)+1\right]+\left(y+2\right)^2-4\\ C=\left(2x+y+1\right)^2+\left(y+2\right)^2-4\ge-4\\ C_{min}=-4\Leftrightarrow\left\{{}\begin{matrix}2x=-1-y\\y=-2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-\dfrac{3}{2}\\y=-2\end{matrix}\right.\)
\(D=\left(3x-1-2x\right)^2=\left(x-1\right)^2\ge0\\ D_{min}=0\Leftrightarrow x=1\\ G=\left(9x^2+6xy+y^2\right)+\left(y^2+4y+4\right)+1\\ G=\left(3x+y\right)^2+\left(y+2\right)^2+1\ge1\\ G_{min}=1\Leftrightarrow\left\{{}\begin{matrix}3x=-y\\y=-2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{2}{3}\\y=-2\end{matrix}\right.\)
5 tháng 10 2021
\(H=\left(x^2-2xy+y^2\right)+\left(x^2+2x+1\right)+\left(2y^2+4y+2\right)+2\\ H=\left(x-y\right)^2+\left(x+1\right)^2+2\left(y+1\right)^2+2\ge2\\ H_{min}=2\Leftrightarrow\left\{{}\begin{matrix}x=y\\x=-1\\y=-1\end{matrix}\right.\Leftrightarrow x=y=-1\)
Ta luôn có \(\left(x-y\right)^2+\left(y-z\right)^2+\left(z-x\right)^2\ge0\)
\(\Leftrightarrow2x^2+2y^2+2z^2-2xy-2yz-2xz\ge0\\ \Leftrightarrow x^2+y^2+z^2\ge xy+yz+xz\\ \Leftrightarrow x^2+y^2+z^2+2xy+2yz+2xz\ge3xy+3yz+3xz\\ \Leftrightarrow\left(x+y+z\right)^2\ge3\left(xy+yz+xz\right)\\ \Leftrightarrow\dfrac{3^2}{3}\ge xy+yz+xz\\ \Leftrightarrow K\le3\\ K_{max}=3\Leftrightarrow x=y=z=1\)
UT
1 tháng 8 2021
A=-(x^2-4x-2) =-(x-2)^2+6 =<6
Max A=6 khi x=2
B=-(x^2 -x-2)= -(x-1/2)^2+9/4=<9/4
Max B=9/4 khi x=1/2
1 tháng 8 2021
\(A=-\left(x^2-4x-2\right)=-\left(x^2-4x+4-6\right)=-\left(x-2\right)^2+6\le6\)
dấu"=" xảy ra<=>x=2
\(B=-\left(x^2-x-2\right)=-\left(x^2-2.\dfrac{1}{2}x+\dfrac{1}{4}-\dfrac{9}{4}\right)\)
\(=-\left(x-\dfrac{1}{2}\right)^2+\dfrac{9}{4}\le\dfrac{9}{4}\) dấu""=" xảy ra<=>x=1/2
VA
4
MH
1 tháng 9 2021
a)A=4(x+11/8)^2 -153/16
Min A=-153/16 khi x=-11/8
b)B=3(x-1/3)^2 -4/3
Min B=-4/3 khi x=1/3
1 tháng 9 2021
Bài 1:
a) \(A=4x^2+11x-2=\left(4x^2+11x+\dfrac{121}{16}\right)-\dfrac{153}{16}=\left(2x+\dfrac{11}{4}\right)^2-\dfrac{153}{16}\ge-\dfrac{153}{16}\)
\(minA=-\dfrac{153}{16}\Leftrightarrow x=-\dfrac{11}{8}\)
b) \(B=3x^2-2x-1=3\left(x^2-\dfrac{2}{3}x+\dfrac{1}{9}\right)-\dfrac{4}{3}=3\left(x-\dfrac{1}{3}\right)^2-\dfrac{4}{3}\ge-\dfrac{4}{3}\)
\(minB=-\dfrac{4}{3}\Leftrightarrow x=\dfrac{1}{3}\)
Bài 2:
a) \(A=-x^2+3x-1=-\left(x^2-3x+\dfrac{9}{4}\right)+\dfrac{5}{4}=-\left(x-\dfrac{3}{2}\right)^2+\dfrac{5}{4}\le\dfrac{5}{4}\)
\(maxA=\dfrac{5}{4}\Leftrightarrow x=\dfrac{3}{2}\)
b) \(B=-x^2-4x+7=-\left(x^2+4x+4\right)+11=-\left(x+2\right)^2+11\le11\)
\(maxB=11\Leftrightarrow x=-2\)
NV
Nguyễn Việt Lâm
Giáo viên
24 tháng 9 2019
\(A=\left(y-2\right)^2+y^2=5y^2-4y+4\)
\(A=5\left(y^2-2.\frac{2}{5}y+\frac{4}{25}\right)+\frac{16}{5}=5\left(y-\frac{2}{5}\right)^2+\frac{16}{5}\ge\frac{16}{5}\)
\(\Rightarrow A_{min}=\frac{16}{5}\) ;
\(A_{max}\) không tồn tại
NT
Tìm GTLN, GTNN của biểu thức sau
\(1,A=\left(x-1\right)^2-10\)
\(2,B=-|x-1|-2\left(2y-1\right)^2+100\)
2
12 tháng 5 2022
1: \(A=\left(x-1\right)^2-10\ge-10\)
Dấu '=' xảy ra khi x=1
2: \(B=-\left|x-1\right|-2\cdot\left(2y-1\right)^2+100\le100\)
Dấu '=' xảy ra khi x=1 và y=1/2
12 tháng 5 2022
`(x-1)^2 >=0 => (x-1)^2 - 10 >= -10`
Dấu bằng xảy ra khi `x = 1`.
Vì `-|x-1| <=0, -2(2y-1)^2 <= 0`
`=> -|x-1| - 2(2y-1)^2 + 100 <= 100`
Dấu bằng xảy ra `<=> x = 1, y = 1/2`.
4 tháng 1 2022
A=(x+2)^2+5
(x+2)^2≥0
Dấu = xay ra ⇔x=-2
Vậy GTNN của A=5<=>x=-2
4 tháng 1 2022
B=(x-2)^2+9
(x-2)^2≥0
Dấu = xay ra ⇔x=2
Vậy GTNN của B=9<=>x=2
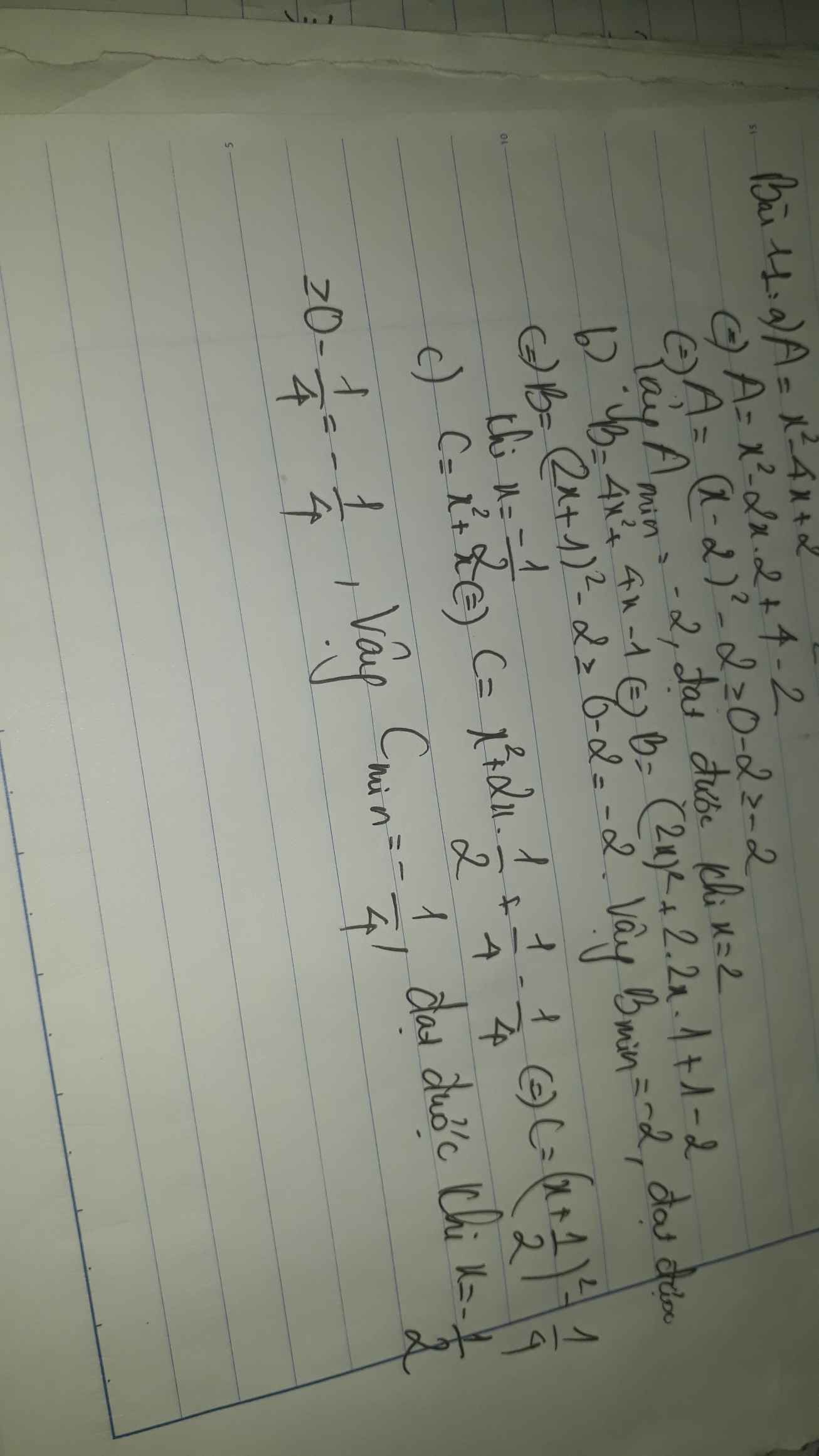
\(A_{max}\Leftrightarrow\frac{x}{\left(x+10\right)^2}\)lớn nhất \(\Rightarrow\left(x+10\right)^2\)nhỏ nhất \(\Rightarrow x+10\)nhỏ nhất
Mà mẫu luôn dương \(\Rightarrow\)tử phải luôn dương tức \(x\ge0\)
\(\Rightarrow x+10\)nhỏ nhất ( với \(x\ge0\)) \(\Leftrightarrow x=0\)
Thay vào ta có \(\frac{0}{10^2}=0\)
Vậy \(A_{max}=0\Leftrightarrow x=0\)
Câu B tương tự và cũng = 0 nhé
CHúc bạn học tốt nha