Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: \(M=3x^5y^3-3x^5y^3-4x^4y^3+2x^4y^3+7xy^2=-2x^4y^3+7xy^2\)
b: \(P\left(x\right)=2x^3-2x+x^2-x^3+3x+2=x^3+x^2+x+2\)
c: \(M\left(x\right)=-3x^4y^3+10+xy\)
\(a)M=3x^5y^3-4x^4y^3+2x^4y^3+7xy^2-3x^5y^3\)
\(M=\left(3x^5y^3-3x^5y^3\right)+\left(-4x^4y^3+2x^4y^3\right)+7xy^2\)
\(M=-2x^4y^3+7xy^2\)
\(\text{Bậc là:}7\)
\(b)P\left(x\right)=2x^3-2x+x^2-x^3+3x+2\)
\(P\left(x\right)=\left(2x^3-x^3\right)+\left(-2x+3x\right)+x^2+2\)
\(P\left(x\right)=x^3+x+x^2+2\)
\(P\left(x\right)=x^3+x^2+x+2\)
\(\text{Bậc là:}3\)
\(M=\left(6x^6y-6x^6y\right)+\left(x^4y^3-4x^4y^3\right)+10+xy\)
\(M=-3x^4y^3+10+xy\)
\(\text{Bậc là:}7\)

Lời giải:
$2x^3-4x^2+a-10=2x^2(x-2)+a-10$
$\Rightarrow$ để $2x^3-4x^2+a-10$ chia hết cho $x-2$ thì $a-10=0$
$\Leftrightarrow a=10$

a: \(P\left(x\right)=-5x^3+3x^2+2x+5\)
\(Q\left(x\right)=-5x^3+6x^2+2x+5\)
b: \(H\left(x\right)=P\left(x\right)+Q\left(x\right)=-10x^3+9x^2+4x+10\)
\(H\left(\dfrac{1}{2}\right)=-10\cdot\dfrac{1}{8}+\dfrac{9}{4}+2+10=13\)
c: Q(x)-P(x)=6
\(\Leftrightarrow3x^2=6\)
hay \(x\in\left\{\sqrt{2};-\sqrt{2}\right\}\)

`a)`
`A(x) + B(x) = 2x - 4x^2 + 1 + x^3 - 4x^2 + 5 - 2x`
`= x^3 - ( 4x^2 + 4x^2 ) + ( 2x - 2x ) + ( 1+ 5 )`
`= x^3 - 8x^2 + 6`
__________________________________________________________
`b)`
`P(x) + B(x) = A(x)`
`=>P(x) = A(x) - B(x)`
`=>P(x) = 2x - 4x^2 + 1 + x^3 + 4x^2 - 5 + 2x`
`=>P(x) = x^3 + ( -4x^2 + 4x^2 ) + ( 2x + 2x ) + ( 1 - 5 )`
`=>P(x) = x^3 + 4x - 4`

N(x) = 2x + x3 + x2 - 4x - x3
= x2 - 2x
N(x) = 0 <=> x2 - 2x = 0
<=> x(x - 2) = 0
<=> x = 0 hoặc x - 2 = 0
<=> x = 0 hoặc x = 2
Vậy nghiệm của N(x) là 0 và 2
\(N\left(x\right)=2x+x^3+x^2-4x-x^3=x^2-2x=x\left(x-2\right)\)
Để N(x) có nghiệm => x(x-2)=0
\(\Leftrightarrow\orbr{\begin{cases}x=0\\x-2=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=0\\x=2\end{cases}}}\)
Vậy x=0; x=2

a) f(x) = x(x - 5) + 2(x - 5)
x(x - 5) + 2(x - 5) = 0
<=> (x - 5)(x - 2) = 0
x - 5 = 0 hoặc x - 2 = 0
x = 0 + 5 x = 0 + 2
x = 5 x = 2
=> x = 5 hoặc x = 2
a, f(x) có nghiệm
\(\Leftrightarrow x\left(x-5\right)+2\left(x-5\right)=0\)
\(\Rightarrow\left(x-5\right)\left(x+2\right)=0\)
\(\Rightarrow\orbr{\begin{cases}x-5=0\\x+2=0\end{cases}}\Rightarrow\orbr{\begin{cases}x=5\\x=-2\end{cases}}\)
->tự kết luận.
b1, để g(x) có nghiệm thì:
\(g\left(x\right)=2x\left(x-2\right)-x^2+5+4x=0\)
\(\Rightarrow2x^2-4x-x^2+5+4x=0\)
\(\Rightarrow x^2+5=0\)
Do \(x^2\ge0\forall x\)nên\(x^2+5\ge5\forall x\)
suy ra: k tồn tại \(x^2+5=0\)
Vậy:.....
b2,
\(f\left(x\right)=x\left(x-5\right)+2\left(x-5\right)\)
\(=x^2-5x+2x-10\)
\(=x^2-3x-10\)
\(f\left(x\right)-g\left(x\right)=x^2+5-\left(x^2-3x-10\right)\)
\(=x^2+5-x^2+3x-10=3x-5\)
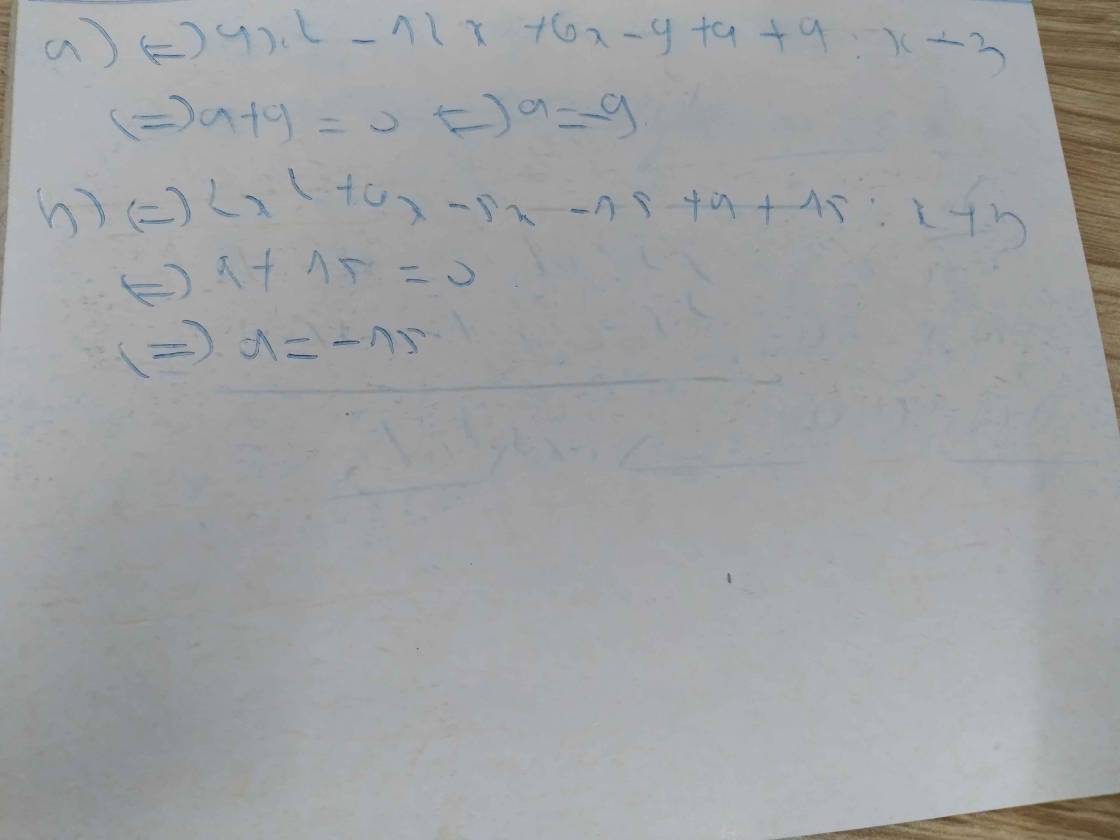
\(2x^3-4x^2+3x+a-10=2x^3-4x^2+3x-6+a-4\)
\(=\left(2x^3-4x^2\right)+\left(3x-6\right)+a-4\)
\(=2x^2\left(x-2\right)+3\left(x-2\right)+a-4\)
\(\Rightarrow\left(2x^3-4x^2+3x+a-10\right):\left(x-2\right)\)
\(=\left[2x^2\left(x-2\right)+3\left(x-2\right)+a-4\right]:\left(x-2\right)\)
\(=2x^2+3+\dfrac{a-4}{x-2}\)
Để đa thức đã cho chia hết cho \(x-2\) thì \(a-4=0\)
\(\Rightarrow a=4\)