K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NT
1
27 tháng 11
đặt x^2+ax+b= (x-1)(x-m)
x^2+ax+b/x^2-1 = x-m/x+1
lim x-m/x+1=-1/2 suy ra 1-m/2=-1/2 nên m = 3
x^2+ax+b= (x-1)(x-3)=x^2-4x+3 suy ra a=-4, b=3
NV
Nguyễn Việt Lâm
Giáo viên
22 tháng 10 2021
Do d' là ảnh của d qua phép tịnh tiến nên d' cùng phương với d
\(\Rightarrow\) Phương trình d' có dạng: \(x-2y+c=0\)
Chọn \(A\left(-1;0\right)\) là 1 điểm thuộc d
Gọi \(A'\left(x';y'\right)\) là ảnh của A qua phép tịnh tiến \(\overrightarrow{v}\Rightarrow A'\in d'\)
\(\left\{{}\begin{matrix}x'=-1+\left(-1\right)=-2\\y'=0+3=3\end{matrix}\right.\) \(\Rightarrow A'\left(-2;3\right)\)
Thế vào pt d':
\(-2-2.3+c=0\Rightarrow c=8\)
Vậy pt d' có dạng: \(x-2y+8=0\)
TB
1
HP
23 tháng 9 2021
j, ĐK: \(x\ne\dfrac{\pi}{6}+\dfrac{k\pi}{2}\)
\(tan\left(\dfrac{\pi}{3}+x\right)-tan\left(\dfrac{\pi}{6}+2x\right)=0\)
\(\Leftrightarrow tan\left(\dfrac{\pi}{3}+x\right)=tan\left(\dfrac{\pi}{6}+2x\right)\)
\(\Leftrightarrow\dfrac{\pi}{3}+x=\dfrac{\pi}{6}+2x+k\pi\)
\(\Leftrightarrow x=\dfrac{\pi}{6}+k\pi\left(l\right)\)
\(\Rightarrow\) vô nghiệm.
HP
6 tháng 9 2021
1.
ĐK: \(\left\{{}\begin{matrix}\dfrac{1+sinx}{1-sinx}\ge0\\1-sinx\ne0\end{matrix}\right.\Leftrightarrow1-sinx\ne0\Leftrightarrow sinx\ne1\Leftrightarrow x=\dfrac{\pi}{2}+k2\pi\)
TN
1
18 tháng 1 2023
\(lim\left(\sqrt[3]{n^3+4}-\sqrt[3]{n^3-1}\right)\)
\(=lim\left(\sqrt[3]{1+\dfrac{4}{n^3}}-\sqrt[3]{1-\dfrac{1}{n^3}}\right)=\sqrt[3]{1}-\sqrt[3]{1}=0\)
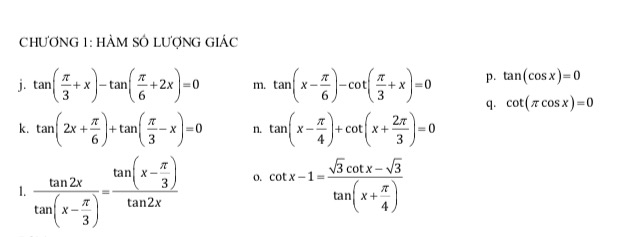
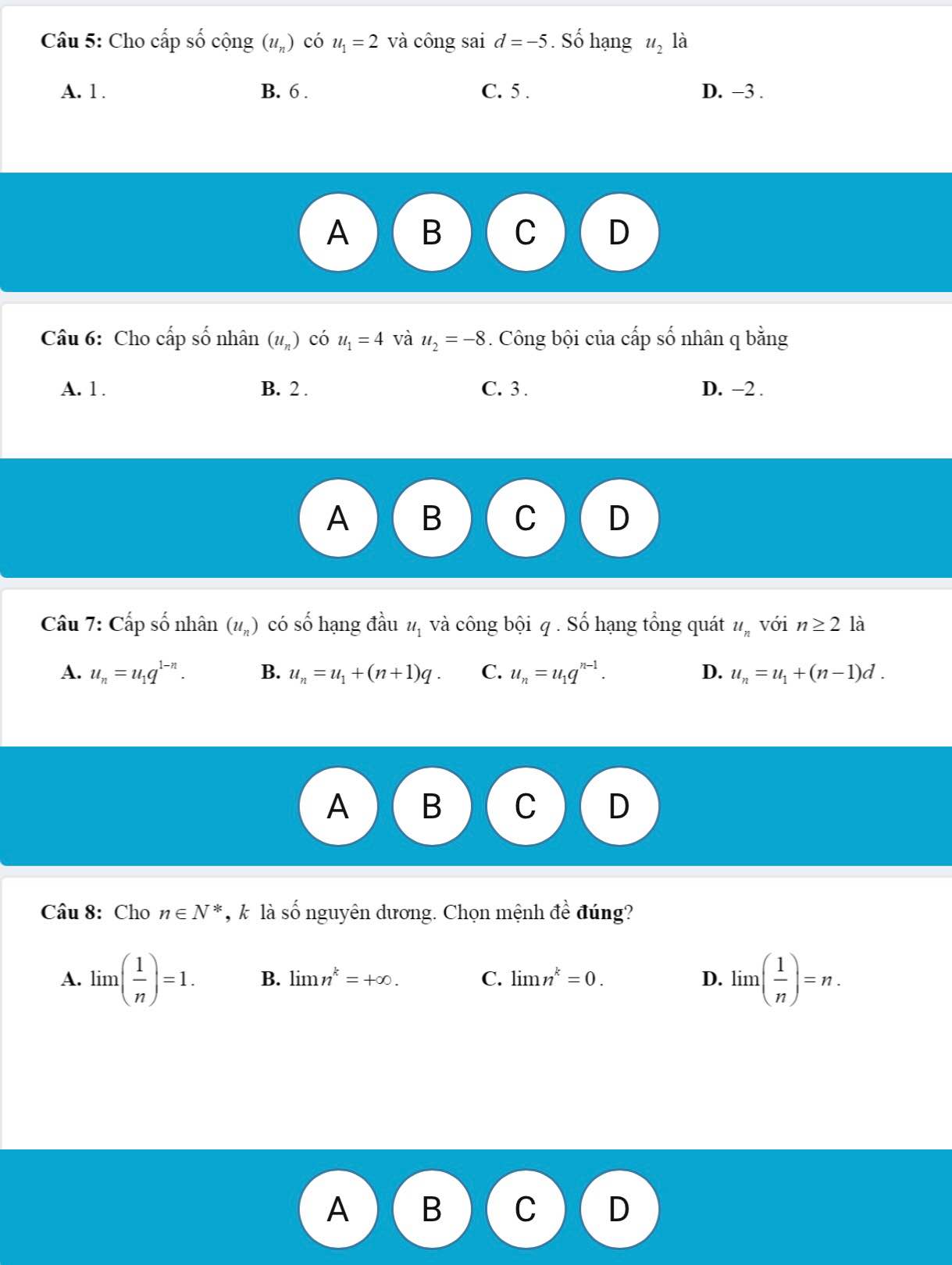
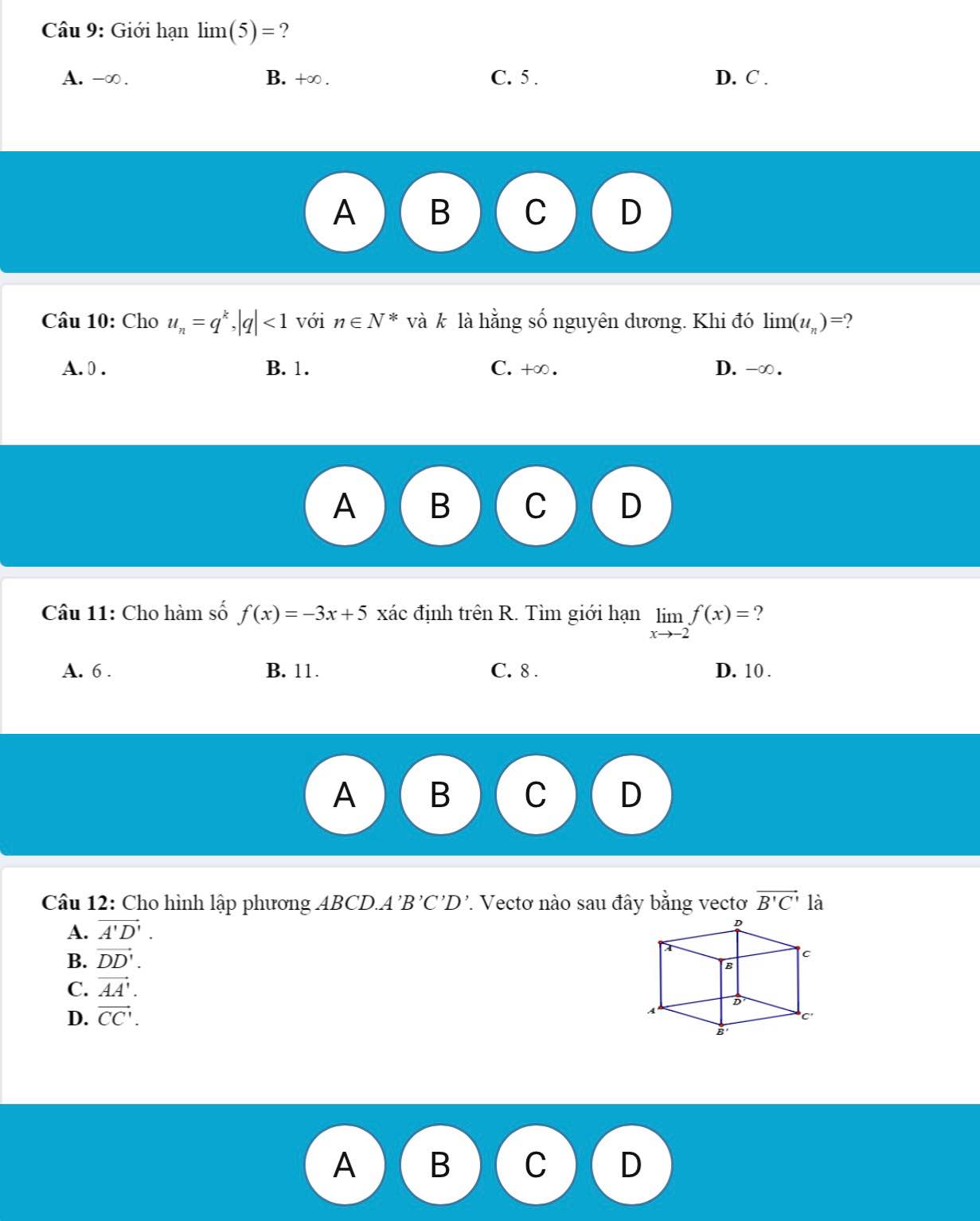
Giúp em với ạ. Cần rất gấp ạ. Em cảm ơn ạ
NT
1
ĐK: \(x\ne\dfrac{\pi}{4}+k\pi;x\ne\dfrac{\pi}{2}+k2\pi\)
\(tan2x.tanx=1\)
\(\Leftrightarrow\dfrac{sin2x.sinx}{cos2x.cosx}=1\)
\(\Leftrightarrow sin2x.sinx=cos2x.cosx\)
\(\Leftrightarrow\dfrac{1}{2}\left(cosx-cos3x\right)=\dfrac{1}{2}\left(cos3x+cosx\right)\)
\(\Leftrightarrow cos3x=0\)
\(\Leftrightarrow3x=\dfrac{\pi}{2}+k\pi\)
\(\Leftrightarrow x=\dfrac{\pi}{6}+\dfrac{k\pi}{3}\)