K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
26 tháng 10 2023
6:
a: ĐKXĐ: x<>0
\(\dfrac{x^3+3x^2+3x+1}{x^2+x}\)
\(=\dfrac{\left(x+1\right)^3}{x\left(x+1\right)}=\dfrac{\left(x+1\right)^2}{x}\)
b: ĐKXĐ: x<>1
\(\dfrac{x^3-3x^2+3x-1}{2x-2}\)
\(=\dfrac{\left(x-1\right)^3}{2\left(x-1\right)}=\dfrac{\left(x-1\right)^2}{2}\)
c: ĐKXĐ: x<>-2
\(\dfrac{x^2+4x+4}{2x+4}\)
\(=\dfrac{\left(x+2\right)^2}{2\left(x+2\right)}\)
\(=\dfrac{x+2}{2}\)
d: ĐKXĐ: x<>-2
\(\dfrac{\left(x-1\right)\left(-x-2\right)}{x+2}\)
\(=\dfrac{\left(-x+1\right)\left(x+2\right)}{x+2}=-x+1\)
e: ĐKXĐ: x<>-y
\(\dfrac{x^2-y^2}{x+y}=\dfrac{\left(x-y\right)\left(x+y\right)}{x+y}=x-y\)
g: ĐKXĐ: \(x\notin\left\{2;-2\right\}\)
\(\dfrac{-3x^2-6x}{4-x^2}=\dfrac{3x^2+6x}{x^2-4}\)
\(=\dfrac{3x\left(x+2\right)}{\left(x+2\right)\cdot\left(x-2\right)}=\dfrac{3x}{x-2}\)
7:
a: \(\dfrac{2}{5x^3y^2}=\dfrac{2\cdot4}{20x^3y^2}=\dfrac{8}{20x^3y^2}\)
\(\dfrac{3}{4xy}=\dfrac{3\cdot5\cdot x^2y}{20x^3y^2}=\dfrac{15x^2y}{20x^3y^2}\)
b: \(\dfrac{x}{x^2-2xy+y^2}=\dfrac{x}{\left(x-y\right)^2}\)
\(\dfrac{x}{x^2-xy}=\dfrac{x}{x\left(x-y\right)}=\dfrac{1}{x-y}=\dfrac{\left(x-y\right)}{\left(x-y\right)^2}\)
c: \(\dfrac{1}{x+2}=\dfrac{6}{6\left(x+2\right)}\)
\(\dfrac{2}{2x+4}=\dfrac{2}{2\left(x+2\right)}=\dfrac{1}{x+2}=\dfrac{6}{6\left(x+2\right)}\)
\(\dfrac{3}{3x+6}=\dfrac{3}{3\left(x+2\right)}=\dfrac{6}{6\left(x+2\right)}\)
d:
\(\dfrac{2}{2x-6}=\dfrac{2}{2\left(x-3\right)}=\dfrac{1}{x-3};\dfrac{3}{3x-9}=\dfrac{3}{3\left(x-3\right)}=\dfrac{1}{x-3}\)
\(\dfrac{2}{2x-6}=\dfrac{1}{x-3}=\dfrac{x+3}{\left(x-3\right)\left(x+3\right)}\)
\(\dfrac{3}{3x-9}=\dfrac{1}{x-3}=\dfrac{x+3}{\left(x-3\right)\left(x+3\right)}\)
\(\dfrac{1}{x+3}=\dfrac{x-3}{\left(x+3\right)\left(x-3\right)}\)
14 tháng 12 2021
\(PT\left(1\right)=\dfrac{3\left(x+1\right)\left(4x-4\right)}{6x\left(x+3\right)\left(x+1\right)}\\ PT\left(2\right)=\dfrac{2\left(x-3\right)\left(x+3\right)}{6x\left(x+1\right)\left(x+3\right)}\)
22 tháng 2 2022
a: \(\dfrac{-7}{x^2-4}=\dfrac{-7}{\left(x-2\right)\left(x+2\right)}=\dfrac{-14}{2\left(x-2\right)\left(x+2\right)}\)
\(\dfrac{11}{2x+4}=\dfrac{11}{2\left(x+2\right)}=\dfrac{11\left(x-2\right)}{2\left(x+2\right)\left(x-2\right)}\)
b: \(\dfrac{2}{9x^2-1}=\dfrac{2}{\left(3x-1\right)\left(3x+1\right)}\)
\(\dfrac{4x}{1-3x}=\dfrac{-4x}{3x-1}=\dfrac{-4x\left(3x+1\right)}{\left(3x-1\right)\left(3x+1\right)}\)
c: \(\dfrac{3}{x+2}=\dfrac{6\left(x^2-2x+4\right)}{2\left(x+2\right)\left(x^2-2x+4\right)}\)
\(\dfrac{x+1}{x^3+8}=\dfrac{2x+2}{2\left(x+1\right)\left(x^2-2x+4\right)}\)
\(\dfrac{x+2}{2\left(x+2\right)}=\dfrac{\left(x+2\right)\left(x^2-2x+4\right)}{2\left(x+2\right)\left(x^2-2x+4\right)}\)
29 tháng 7 2021
cho mình hỏi là giữa khác phân số với nhua là phải có dấu như là công, trừ, nhân hay chia chứ?
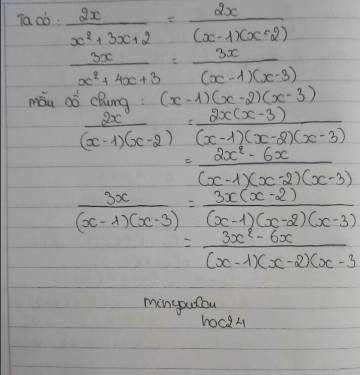
\(\frac{3x}{2x+4};\frac{x+3}{x^2-4}\)
Ta ó : \(2x+4=2\left(x+2\right)\)
\(x^2-4=\left(x-2\right)\left(x+2\right)\)
MTC : \(2\left(x-2\right)\left(x+2\right)\)
\(\frac{3x}{2x+4}=\frac{3x}{2\left(x+2\right)}=\frac{3x\left(x-2\right)}{2\left(x+2\right)\left(x-2\right)}=\frac{3x^2-6x}{2\left(x+2\right)\left(x-2\right)}\)
\(\frac{x+3}{x^2-4}=\frac{x+3}{\left(x-2\right)\left(x+2\right)}=\frac{2\left(x+3\right)}{2\left(x-2\right)\left(x+2\right)}=\frac{2x+6}{2\left(x-2\right)\left(x+2\right)}\)
\(\hept{\begin{cases}\frac{3x}{2x+4}=\frac{3x}{2\left(x+2\right)}\\\frac{x+3}{x^2-4}=\frac{x+3}{\left(x-2\right)\left(x+2\right)}\end{cases}}\)
MTC : 2( x - 2 )( x + 2 )
=> \(\hept{\begin{cases}\frac{3x}{2x+4}=\frac{3x}{2\left(x+2\right)}=\frac{3x\left(x-2\right)}{2\left(x-2\right)\left(x+2\right)}=\frac{3x^2-6x}{2\left(x-2\right)\left(x+2\right)}\\\frac{x+3}{x^2-4}=\frac{x+3}{\left(x-2\right)\left(x+2\right)}=\frac{2\left(x+3\right)}{2\left(x-2\right)\left(x+2\right)}=\frac{2x+6}{2\left(x-2\right)\left(x+2\right)}\end{cases}}\)