Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Kẻ \(AH\perp BC\)
Áp dụng hệ thức lượng: \(\dfrac{1}{AH^2}=\dfrac{1}{AB^2}+\dfrac{1}{AC^2}=\dfrac{4}{3a^2}\Rightarrow AH=\dfrac{a\sqrt{3}}{2}\)
\(tan\widehat{SHA}=\dfrac{2}{\sqrt{3}}=\dfrac{SA}{AH}\Rightarrow SA=\dfrac{AH.2}{\sqrt{3}}=a\)
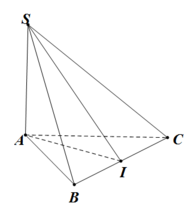
Gọi M là trung điểm BC và N là trung điểm SA, dựng hình chữ nhật AMIN \(\Rightarrow\) I là tâm mặt cầu ngoại tiếp
\(AN=\dfrac{1}{2}SA=\dfrac{a}{2}\) ; \(AM=\dfrac{1}{2}BC=\dfrac{1}{2}\sqrt{AB^2+AC^2}=a\)
\(\Rightarrow R=IA=\sqrt{AM^2+AN^2}=\dfrac{a\sqrt{5}}{2}\)
\(V=\dfrac{4}{3}\pi R^3=...\)

\(BC=\sqrt{AB^2+AC^2}=2a\)
Gọi M là trung điểm BC \(\Rightarrow AM=\dfrac{1}{2}BC=a\)
GỌi N là trung điểm SA \(\Rightarrow AN=\dfrac{1}{2}SA=a\)
Dựng hình chữ nhật AMIN \(\Rightarrow\) I là tâm mặt cầu ngoại tiếp
\(R=IA=\sqrt{AM^2+AN^2}=a\sqrt{2}\)
\(\Rightarrow V=\dfrac{4}{3}\pi R^3=...\)

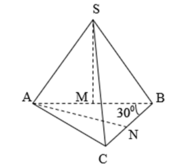
Gọi O là tâm đáy \(\Rightarrow SO\perp\left(ABCD\right)\)
Gọi M là trung điểm AB \(\Rightarrow AB\perp OM\Rightarrow AB\perp\left(SOM\right)\)
\(\Rightarrow\widehat{SMO}\) là góc giữa mặt bên và đáy hay \(\widehat{SMO}=60^0\)
\(SO=OM.tan\widehat{SMO}=\dfrac{a}{2}.tan60^0=\dfrac{a\sqrt{3}}{2}\)
\(V=\dfrac{1}{3}SO.S_{ABCD}=\dfrac{1}{3}.\dfrac{a\sqrt{3}}{2}.a^2=\dfrac{a^3\sqrt{3}}{6}\)
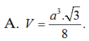
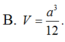

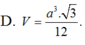


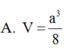
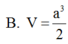

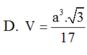
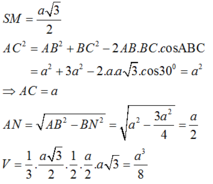
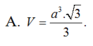
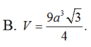
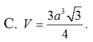
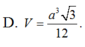
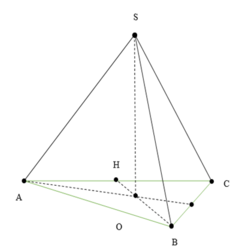
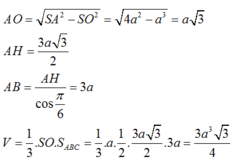
Đáp án C