K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
26 tháng 11 2017
Áp dụng BĐT Bunhiacopski ta có:
\((x^2+y^2+z^2)(1^2+1^2+1^2)\ge(x.1+y.1+z.1)^2\)
<=>3(\(x^2+y^2+z^2)\ge3^2\)
<=>\(x^2+y^2+z^2\ge3\)
Dấu "=" xảy ra <=> x=y=z=1
Vậy minA=3<=>x=y=z=1
KK
26 tháng 8 2021
a. x2 - 2x
⇔ x(x - 2)
b. 3x - 6y
⇔ 3(x - 2y)
c. 5(x + 3y) - 15x(x + 3y)
⇔ (5 - 15x)(x + 3y)
d. 3(x - y) - 5x(y - x)
⇔ 3(x - y) + 5x(x - y)
⇔ (3 + 5x)(x - y)
20 tháng 9 2021
a: Xét ΔADM và ΔCBN có
AD=CB
\(\widehat{DAM}=\widehat{BCN}\)
AM=CN
Do đó: ΔADM=ΔCBN
Suy ra: DM=BN
3 tháng 5 2021
Em cần giúp câu nào hả em? Em nên chụp 1-2 ý cho 1 lần hỏi nhá, như thế mọi người sẽ dễ dàng giúp em hơn
PN
12 tháng 5 2021
13
a, \(3x-4=-x+8\)
\(< =>3x+x=8+4\)
\(< =>4x=12\)
\(< =>x=\frac{12}{4}=3\)
b, \(\frac{2x+1}{6}+\frac{x-7}{12}=10\)
\(< =>\frac{2\left(2x+1\right)}{12}+\frac{x-7}{12}=\frac{120}{12}\)
\(< =>4x+2+x-7=120\)
\(< =>5x=120+5=125\)
\(< =>x=\frac{125}{5}=\frac{5^3}{5}=5^2=25\)
TK
2
9 tháng 12 2021
\(a,=\dfrac{4xy-1-2xy+1}{5x^2y}=\dfrac{6xy}{5x^2y}=\dfrac{6}{5x}\\ b,=\dfrac{x^2+8x-2x+8}{x\left(x-4\right)\left(x+4\right)}=\dfrac{\left(x+2\right)\left(x+4\right)}{x\left(x-4\right)\left(x+4\right)}=\dfrac{x+2}{x\left(x-4\right)}\\ c,=\dfrac{x^2+3x-x+1}{x\left(x+1\right)\left(x-1\right)}=\dfrac{\left(x+1\right)^2}{x\left(x-1\right)\left(x+1\right)}=\dfrac{x+1}{x\left(x-1\right)}\\ d,=\dfrac{x-3-x-3-2x}{\left(x-3\right)\left(x+3\right)}=\dfrac{-2\left(x+3\right)}{\left(x-3\right)\left(x+3\right)}=\dfrac{2}{3-x}\\ e,=\dfrac{x+1-1}{x+1}=\dfrac{x}{x+1}\\ f,=\dfrac{3x+5-5+9x}{6x^2y}=\dfrac{12x}{6xy}=\dfrac{2}{y}\)
9 tháng 12 2021
\(g,=\dfrac{x^2+6x-2x+4}{x\left(x+2\right)\left(x-2\right)}=\dfrac{\left(x+2\right)^2}{x\left(x+2\right)\left(x-2\right)}=\dfrac{x+2}{x\left(x-2\right)}\\ h,=\dfrac{3x+1-3x+1+2x-3}{\left(3x-1\right)\left(3x+1\right)}=\dfrac{2x-1}{\left(3x-1\right)\left(3x+1\right)}\\ j,=\dfrac{5x+30+x^2-30}{x\left(x+6\right)}=\dfrac{x^2+5x}{x^2+6x}\\ k,=\dfrac{\left(x-7\right)\left(x+7\right)}{2x+1}\cdot\dfrac{-3}{x-7}=\dfrac{-3\left(x+7\right)}{2x+1}\\ l,=\dfrac{x\left(3x-2\right)}{x^2-1}\cdot\dfrac{\left(x^2+1\right)\left(x^2-1\right)}{\left(3x-2\right)^3}=\dfrac{x\left(x^2+1\right)}{\left(3x-2\right)^2}\)
TV
1
NV
Nguyễn Việt Lâm
Giáo viên
11 tháng 12 2021
\(A=\dfrac{x^3-2x^2-15x}{x-5}=\dfrac{x\left(x^2-2x-15\right)}{x-5}=\dfrac{x\left(x+3\right)\left(x-5\right)}{x-5}=x\left(x+3\right)\)
\(A=x^2+3x=\left(x^2+3x+\dfrac{9}{4}\right)-\dfrac{9}{4}=\left(x+\dfrac{3}{2}\right)^2-\dfrac{9}{4}\ge-\dfrac{9}{4}\)
\(A_{min}=-\dfrac{9}{4}\)
18 tháng 9 2021
\(a,=\left(x+4\right)^3=\left(6+4\right)^3=10^3=1000\\ b,=x^3-1+x^3-27=2x^3-28\\ =2\left(-1\right)^3-28=-2-28=-30\\ c,=\left[4x-6-4\left(3-x\right)\right]\left[4x-64++4\left(3-x\right)\right]=6\left(8x-18\right)=12\left(4x-9\right)\\ =12\left(-7\cdot4-9\right)=12\left(-37\right)=-444\)
DT
Tìm GTNN của A=\(x^4-6x^3+12x^2-12x+2021\)
Giúp mk vs ạ mk đang cần gấp ai nhanh mk sẽ vote cho ạ :<
1
7 tháng 12 2021
\(Sửa:A=x^4-6x^3+13x^2-12x+2021\\ A=\left(x^4-6x^3+9x^2\right)+4\left(x^2-3x\right)+4+2017\\ A=\left(x^2-3x\right)^2+4\left(x^2-3x\right)+4+2017\\ A=\left(x^2-3x+2\right)^2+2017\ge2017\\ A_{min}=2017\Leftrightarrow x^2-3x+2=0\Leftrightarrow\left(x-2\right)\left(x-1\right)=0\Leftrightarrow\left[{}\begin{matrix}x=1\\x=2\end{matrix}\right.\)
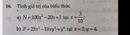
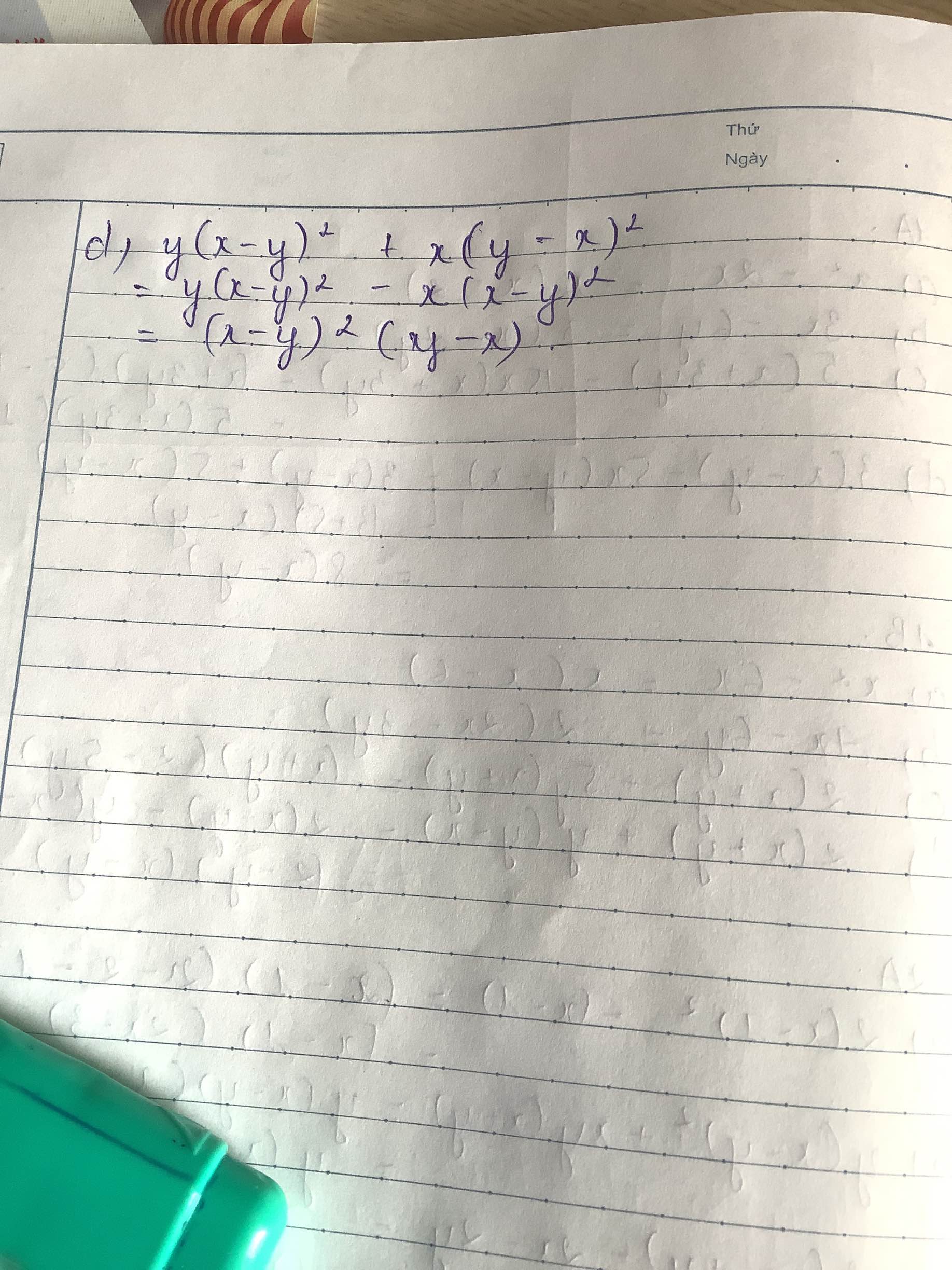
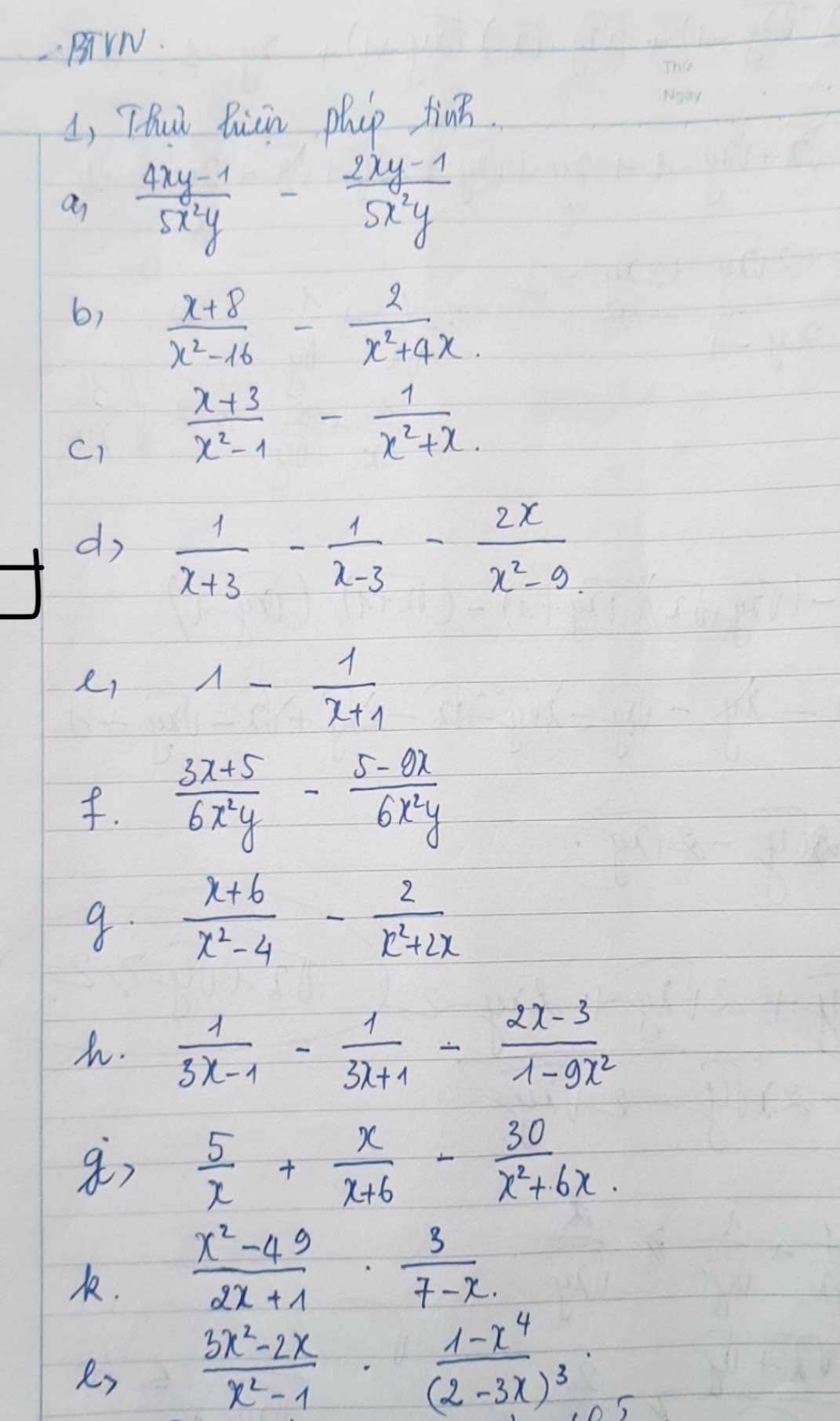
ta có
a.\(N=\left(10x\right)^2-2.\left(10x\right)+1=\left(10x-1\right)^2=\left(3-1\right)^2=4\text{ khi }x=\frac{3}{10}\)
b.\(P=\left(5x\right)^2-2.5xy^2+y^4=\left(5x-y^2\right)^2=\left(5.5-4^2\right)=9^2=81\)