K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
15 tháng 9 2021
1: \(100-x^2=\left(10-x\right)\left(10+x\right)\)
2: \(b^2-a^2=\left(b-a\right)\left(b+a\right)\)
3: \(\left(3y\right)^2-\left(4x\right)^2=\left(3y-4x\right)\left(3y+4x\right)\)
NV
Nguyễn Việt Lâm
Giáo viên
21 tháng 12 2022
\(-2xy\left(4xy+9x^2+4y\right)=-2xy.4xy+\left(-2xy\right).9x^2+\left(-2xy\right).4y\)
\(=-8x^2y^2-18x^3y-8xy^2\)
\(x\left(x+8y\right)-y\left(8x-y^2\right)=x^2+8xy-8xy+y^3\)
\(=x^2+y^3\)
T
17 tháng 11 2023
\(\dfrac{4x+2}{4x-2}+\dfrac{3-6x}{6x-6}\left(dkxd:x\ne\dfrac{1}{2};x\ne1\right)\)
\(=\dfrac{2\left(2x+1\right)}{2\left(2x-1\right)}+\dfrac{3\left(1-2x\right)}{6\left(x-1\right)}\)
\(=\dfrac{2x+1}{2x-1}+\dfrac{1-2x}{2\left(x-1\right)}\)
\(=\dfrac{2x+1}{2x-1}+\dfrac{1-2x}{2x-2}\)
\(=\dfrac{\left(2x+1\right)\left(2x-2\right)}{\left(2x-1\right)\left(2x-2\right)}+\dfrac{\left(1-2x\right)\left(2x-1\right)}{\left(2x-1\right)\left(2x-2\right)}\)
\(=\dfrac{4x^2-2x-2}{\left(2x-1\right)\left(2x-2\right)}+\dfrac{-4x^2+4x-1}{\left(2x-1\right)\left(2x-2\right)}\)
\(=\dfrac{4x^2-2x-2-4x^2+4x-1}{\left(2x-1\right)\left(2x-2\right)}\)
\(=\dfrac{2x-3}{\left(2x-1\right)\left(2x-2\right)}\)
\(=\dfrac{2x-3}{4x^2-6x+2}\)
TG
2
LM
28 tháng 6 2021
a): ta có AB^2 + AC^2 = 30^2 = 900 <=> AB = √(900 - AC^2)
AB:AC = 3:4 <=> AB = 3 * AC / 4
=> √(900 - AC^2) = 3 * AC / 4
<=> 900 - AC^2 = 9 * AC^2 / 16
<=> 14400 - 16 * AC^2 = 9 * AC^2
<=> 14400 = 25 * AC^2
<=> 576 = AC^2
<=> AC = 24
=> AB = 24 / 4 * 3 = 18
TP
1
18 tháng 4 2021
\(\dfrac{x+2}{x-3}< 0\)vì \(x+2>x-3\)
\(\left\{{}\begin{matrix}x+2>0\\x-3< 0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x>-2\\x< 3\end{matrix}\right.\)<=> -2 < x < 3
KL
1
19 tháng 12 2021
a: Xét tứ giác ABDC có
N là trung điểm của BC
N là trung điểm của AD
Do đó: ABDC là hình bình hành
mà \(\widehat{BAC}=90^0\)
nên ABDC là hình chữ nhật
AT
1
3 tháng 1 2017
Áp dụng định lý Bê-du, số dư của x100 cho x+1 = x- (-1) là (-1)100=1
Vậy ....
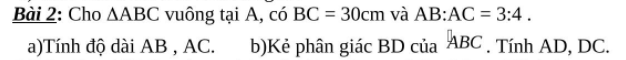
a: \(=\dfrac{5xy-4y+3xy+4y}{2x^2y^3}=\dfrac{8xy}{2x^2y^3}=\dfrac{4}{xy^2}\)
b: \(=\dfrac{\left(x^2-1\right)\left(x^2+1\right)-2x\left(x^2-1\right)}{x^2-1}=x^2-2x+1\)
c: \(=\dfrac{3x-x+6}{x\left(2x+6\right)}=\dfrac{2x+6}{x\left(2x+6\right)}=\dfrac{1}{x}\)