
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


5:
B=(x+y)^2-2xy
=225-2*(-100)=425
6:
a: C=(39+61)^2=100^2=10000
b: D=50^2-50^2+1=1

\(ĐK:x\ne\dfrac{1}{2};x\ne1;x\ne\dfrac{3}{2};x\ne2;x\ne\dfrac{5}{2}\\ PT\Leftrightarrow\dfrac{1}{\left(2x-1\right)\left(x-1\right)}+\dfrac{1}{\left(x-1\right)\left(3x-2\right)}+\dfrac{1}{\left(3x-2\right)\left(x-2\right)}+\dfrac{1}{\left(x-2\right)\left(5x-2\right)}=\dfrac{4}{21}\\ \Leftrightarrow2\left[\dfrac{\dfrac{1}{2}}{\left(x-\dfrac{1}{2}\right)\left(x-1\right)}+\dfrac{\dfrac{1}{2}}{\left(x-1\right)\left(x-\dfrac{3}{2}\right)}+\dfrac{\dfrac{1}{2}}{\left(x-\dfrac{3}{2}\right)\left(x-2\right)}+\dfrac{\dfrac{1}{2}}{\left(x-2\right)\left(x-\dfrac{5}{2}\right)}\right]=\dfrac{4}{21}\)
\(\Leftrightarrow\dfrac{1}{x-1}-\dfrac{1}{x-\dfrac{1}{2}}+\dfrac{1}{x-\dfrac{3}{2}}-\dfrac{1}{x-1}+\dfrac{1}{x-2}-\dfrac{1}{x-\dfrac{3}{2}}+\dfrac{1}{x-\dfrac{5}{2}}-\dfrac{1}{x-2}=\dfrac{2}{21}\\ \Leftrightarrow\dfrac{1}{x-1}-\dfrac{1}{x-\dfrac{5}{2}}=\dfrac{2}{21}\\ \Leftrightarrow\dfrac{x-\dfrac{5}{2}-x+1}{\left(x-1\right)\left(x-\dfrac{5}{2}\right)}=\dfrac{2}{21}\\ \Leftrightarrow\dfrac{-\dfrac{3}{2}}{x^2-\dfrac{7}{2}x+\dfrac{5}{2}}=\dfrac{2}{21}\\ \Leftrightarrow x^2-\dfrac{7}{2}x+\dfrac{5}{2}=-\dfrac{63}{4}\\ \Leftrightarrow4x^2-14x+10=-63\\ \Leftrightarrow4x^2-14x+73=0\\ \Leftrightarrow x\in\varnothing\)


Bài 2
a) 3x(x - 1) - 3(x - 1) = 0
(x - 1)(3x - 3) = 0
3(x - 1)(x - 1) = 0
3(x - 1)² = 0
x - 1 = 0
x = 1
b) x² - x = 0
x(x - 1) = 0
x = 0 hoặc x - 1 = 0
*) x - 1 = 0
x = 1
Vậy x = 0; x = 1
c) 25x² - 100x = 0
25x(x - 4) = 0
25x = 0 hoặc x - 4 = 0
*) 25x = 0
x = 0
*) x - 4 = 0
x = 4
Vậy x = 0; x = 4
d) (2x - 1)² - 64 = 0
(2x - 1 - 8)(2x - 1 + 8) = 0
(2x - 9)(2x + 7) = 0
*) 2x - 9 = 0
2x = 9
x = 9/2
*) 2x + 7 = 0
2x = -7
x = -7/2
Vậy x = -7/2; x = 9/2

Câu 3:
a: \(\left(x+2\right)^2=x^2+4x+4\)
b: \(\left(x+3\right)^2=x^2+6x+9\)
c: \(\left(x-3\right)^2=x^2-6x+9\)
d: \(\left(x-7\right)^2=x^2-14x+49\)
e: \(x^2-6x+9=\left(x-3\right)^2\)
f: \(x^2-8x+16=\left(x-4\right)^2\)
g: \(=\left(x-10\right)\left(x+10\right)\)
h: \(=\left(x-11\right)\left(x+11\right)\)

1) Xét hình thang ADCB(AD//CB) có
M là trung điểm của AB
K là trung điểm của DC
Do đó: MK là đường trung bình của hình thang ADCB(Định nghĩa đường trung bình của tam giác)
Suy ra: MK//AD//BC và \(MK=\dfrac{AD+BC}{2}\)(Định lí 4 về đường trung bình của tam giác)
2) Xét ΔABC có
M là trung điểm của AB
N là trung điểm của AC
Do đó: MN là đường trung bình của ΔABC(Định nghĩa đường trung bình của tam giác)
Suy ra: MN//BC và \(MN=\dfrac{BC}{2}\)(Định lí 2 về đường trung bình của tam giác)
3) Ta có: MN//BC(cmt)
MK//BC(cmt)
mà MN và MK có điểm chung là M
nên M,N,K thẳng hàng(đpcm)


Bài 3:
a:Ta có: \(\left|x+\dfrac{3}{4}\right|-\dfrac{1}{2}=0\)
\(\Leftrightarrow\left|x+\dfrac{3}{4}\right|=\dfrac{1}{2}\)
\(\Leftrightarrow\left[{}\begin{matrix}x+\dfrac{3}{4}=-\dfrac{1}{2}\\x+\dfrac{3}{4}=\dfrac{1}{2}\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{-5}{4}\\x=-\dfrac{1}{4}\end{matrix}\right.\)
b: Ta có: \(23-\left(2x+3\right)^2=-2\)
\(\Leftrightarrow\left(2x+3\right)^2=25\)
\(\Leftrightarrow\left[{}\begin{matrix}2x+3=5\\2x+3=-5\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}2x=2\\2x=-8\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=1\\x=-4\end{matrix}\right.\)
Bài 2:
a: Ta có: \(\left(x-2\right)\left(x+3\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=2\\x=-3\end{matrix}\right.\)
b: Ta có: \(x^3+4x=0\)
\(\Leftrightarrow x\left(x^2+4\right)=0\)
hay x=0



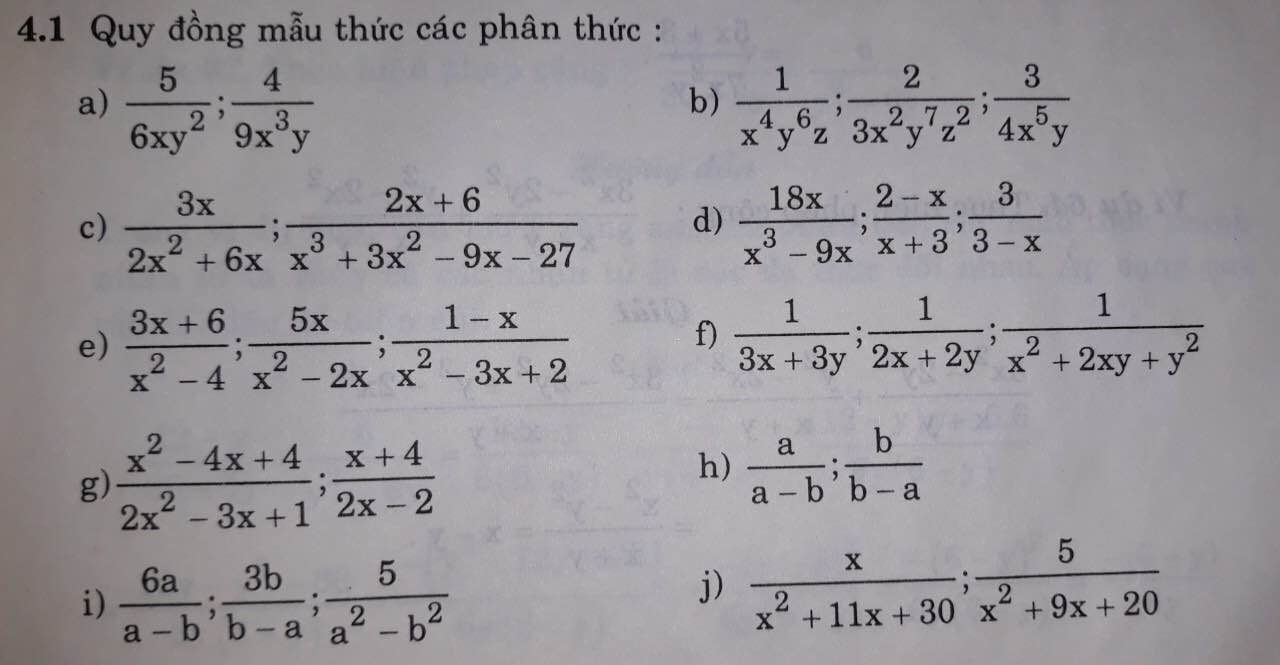
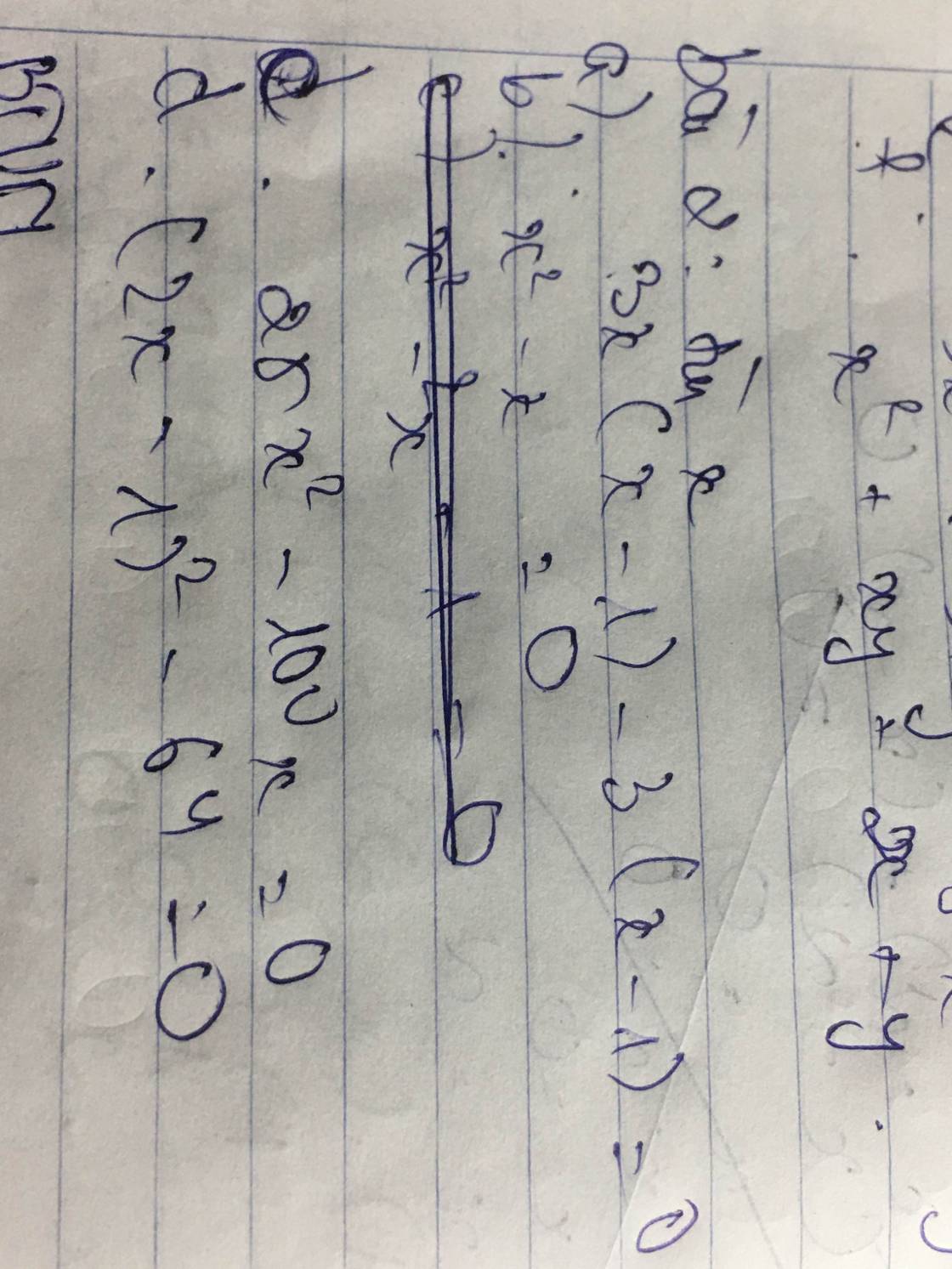



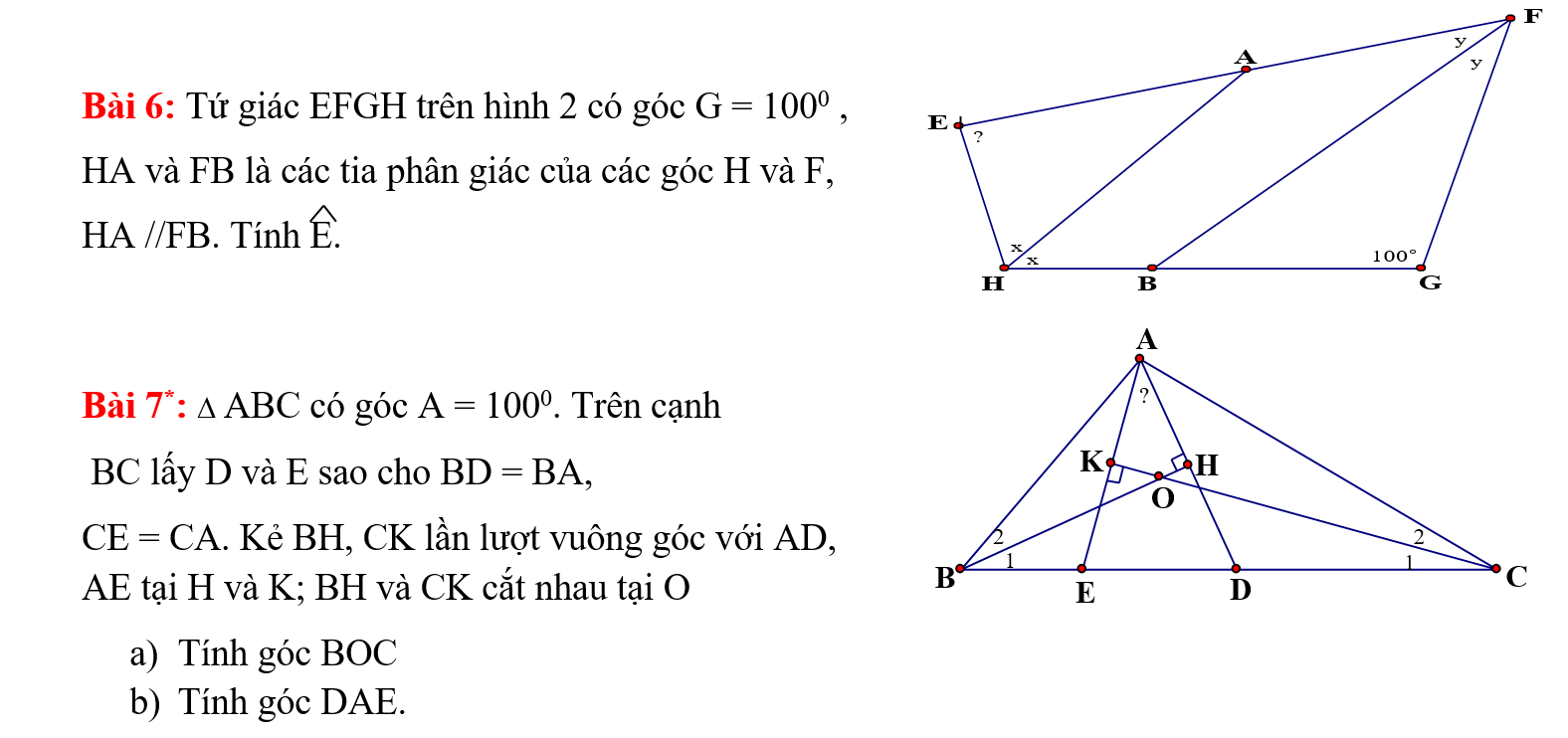

bài 10
b) x3-5x-14=x(x2-5x-14)=x(x2+7x-2x-14)=x[(x2-2x)+(7x-14)]=x[x(x-2)+7(x-2)]=x(x-2)(x+7)
\(\Rightarrow\left[{}\begin{matrix}x=0\\x-2=0\\x+7=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=2\\x=-7\end{matrix}\right.\)
Bài 10:
c: Ta có: \(x^3-5x^2-14x\)
\(=x\left(x^2-5x-14\right)\)
\(=x\left(x-7\right)\left(x+2\right)\)