K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
KV
5 tháng 5 2023
A = 1/(5.6) + 1/(6.7) + ... + 1/(24.25)
= 1/5 - 1/6 + 1/6 - 1/7 + ... + 1/24 - 1/25
= 1/5 - 1/25
= 4/25
B = 2/(1.3) + 2/(3.5) + 2/(5.7) + ... + 2/(99.101)
= 1 - 1/3 + 1/3 - 1/5 + 1/5 - 1/7 + ... + 1/99 - 1/101
= 1 - 1/101
= 100/101
5 tháng 5 2023
`a) A = 1/(5.6) + 1/(6.7)+...+1/(24.25)`
`= 1/5 - 1/6 + 1/6 - 1/7 +...+1/24-1/25`
`= 1/5-1/25`
`= 5/25 - 1/25`
`= 4/25`
Vậy:`A = 4/25`
`b) B = 2/(1.3)+2/(3.5)+...+2/(99.101)`
`= 1- 1/3 + 1/3 - .... +1/99-1/101`
`= 1 - 1/101`
`= 100/101`
Vậy: `B = 100/101`
TK
2
21 tháng 11 2021
\(a^3b-ab^3=ab\left(a^2-b^2\right)=ab\left(a^2-ab+ab-b^2\right)=ab\left(a-b\right)\left(a+b\right)\)
Với a hoặc b chẵn \(\Leftrightarrow ab\left(a-b\right)\left(a+b\right)⋮2\)
Với a và b lẻ \(\Leftrightarrow\left(a-b\right)⋮2\Leftrightarrow ab\left(a-b\right)\left(a+b\right)⋮2\)
Vậy \(ab\left(a-b\right)\left(a+b\right)⋮2,\forall a,b\left(1\right)\)
Với a hoặc b chia hết cho 3 thì \(ab\left(a-b\right)\left(a+b\right)⋮3\)
Với \(a=3k+1;b=3q+1\Leftrightarrow\left(a-b\right)=3\left(k-q\right)⋮3\)
\(\Leftrightarrow ab\left(a-b\right)\left(a+b\right)⋮3\)
Với \(a=3k+1;b=3q+2\Leftrightarrow\left(a+b\right)=\left(3k+1+3q+2\right)=3\left(k+q+1\right)⋮3\)
\(\Leftrightarrow ab\left(a-b\right)\left(a+b\right)⋮3\)
Mà a,b có vai trò tương đương nên \(ab\left(a-b\right)\left(a+b\right)⋮3,\forall a,b\left(2\right)\)
\(\left(1\right)\left(2\right)\Leftrightarrowđpcm\)
21 tháng 11 2021
Ta có : a3b -ab3
=a3b -ab -ab3 +ab
=ab (a2 -1) -ab (b2 -1)
=ab (a-1)(a+1) -ab (b-1)(b+1)
Vì a (a-1)(a+1) là 3 số tự nhiên liên tiếp nên chia hết cho 6 .Tương tự b (b-1)(b+1) cũng chia hết cho 6
=> a3b -ab3 chia hết cho 6 (đpcm )
1 tháng 7 2023
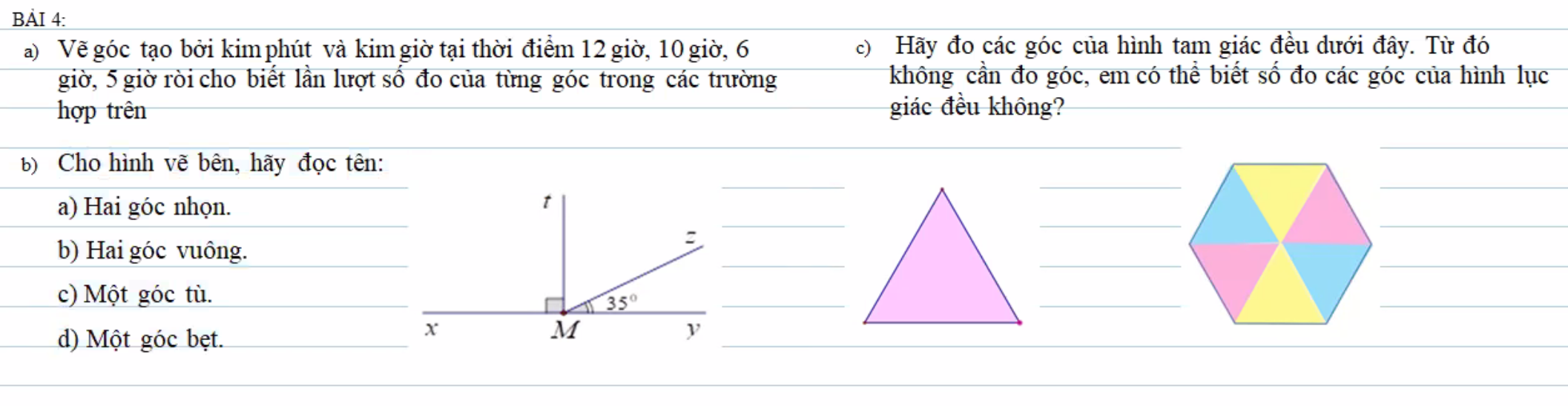
a: 12h: 0 độ
10h: 60 đọ
6h: 180 độ
5h: 150 độ
b:
a: góc nhọn: góc yMz; góc tMz
b: góc vuông: góc yMt, góc xMt
c: góc tù: góc xMz
d: góc bẹt: góc xMy
BT
2
BT
1
PK
1
2 tháng 11 2021
Bài 4:
\(a,\Rightarrow5⋮x\Rightarrow x\inƯ\left(5\right)=\left\{1;5\right\}\\ b,\Rightarrow x-2+7⋮x-2\\ \Rightarrow x-2\inƯ\left(7\right)=\left\{1;7\right\}\\ \Rightarrow x\in\left\{3;9\right\}\\ c,\Rightarrow3\left(x+1\right)+4⋮x+1\\ \Rightarrow x+1\inƯ\left(4\right)=\left\{1;2;4\right\}\\ \Rightarrow x\in\left\{0;1;3\right\}\\ d,\Rightarrow10x+6⋮2x-1\\ \Rightarrow5\left(2x-1\right)+11⋮2x-1\\ \Rightarrow2x-1\inƯ\left(11\right)=\left\{1;11\right\}\\ \Rightarrow x\in\left\{1;6\right\}\\ e,\Rightarrow x\left(x+3\right)+11⋮x+3\\ \Rightarrow x+3\inƯ\left(11\right)=\left\{1;11\right\}\\ \Rightarrow x=8\left(x\in N\right)\\ f,\Rightarrow x\left(x+3\right)+2\left(x+3\right)+5⋮x+3\\ \Rightarrow x+3\inƯ\left(5\right)=\left\{1;5\right\}\\ \Rightarrow x=2\left(x\in N\right)\)
TK
2
18 tháng 6 2023
3:
a: =8/24+9/24-14/24=3/24=1/8
b: =-12/56+35/56-28/56=-5/56
c: =9/36-24/36-22/36=-37/36
d: \(=\dfrac{6}{24}+\dfrac{10}{24}-\dfrac{21}{24}-\dfrac{1}{13}=\dfrac{-5}{24}-\dfrac{1}{13}=\dfrac{-89}{24\cdot13}\)
BT
3
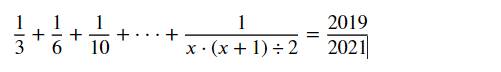
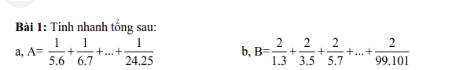
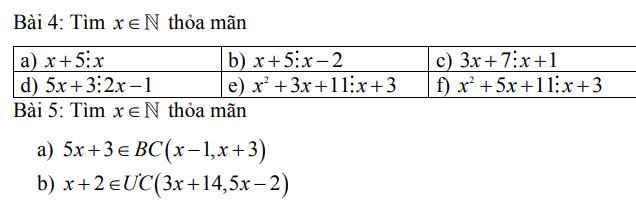
\(\dfrac{1}{3}+\dfrac{1}{6}+\dfrac{1}{10}+...+\dfrac{1}{\dfrac{x\left(x+1\right)}{2}}=\dfrac{2019}{2021}\)
=>\(\dfrac{2}{6}+\dfrac{2}{12}+\dfrac{2}{20}+...+\dfrac{2}{x\left(x+1\right)}=\dfrac{2019}{2021}\)
=>\(2\left(\dfrac{1}{6}+\dfrac{1}{12}+...+\dfrac{1}{x\left(x+1\right)}\right)=\dfrac{2019}{2021}\)
=>\(\dfrac{1}{2}-\dfrac{1}{3}+\dfrac{1}{3}-\dfrac{1}{4}+...+\dfrac{1}{x}-\dfrac{1}{x+1}=\dfrac{2019}{4042}\)
=>\(\dfrac{1}{2}-\dfrac{1}{x+1}=\dfrac{2019}{4042}\)
=>\(\dfrac{1}{x+1}=\dfrac{1}{2}-\dfrac{2019}{4042}=\dfrac{2021-2019}{4042}=\dfrac{2}{4042}=\dfrac{1}{2021}\)
=>x+1=2021
=>x=2020
\(\Leftrightarrow\dfrac{2}{6}+\dfrac{2}{12}+\dfrac{2}{20}+...+\dfrac{2}{x\left(x+1\right)}=\dfrac{2019}{2021}\)
\(\Leftrightarrow\dfrac{2}{2.3}+\dfrac{2}{3.4}+\dfrac{2}{4.5}+...+\dfrac{2}{x\left(x+1\right)}=\dfrac{2019}{2021}\)
\(\Leftrightarrow2\left(\dfrac{1}{2}-\dfrac{1}{3}+\dfrac{1}{3}-\dfrac{1}{4}+\dfrac{1}{4}-\dfrac{1}{5}+...+\dfrac{1}{x}-\dfrac{1}{x+1}\right)=\dfrac{2019}{2021}\)
\(\Leftrightarrow2\left(\dfrac{1}{2}-\dfrac{1}{x+1}\right)=\dfrac{2019}{2021}\)
\(\Leftrightarrow1-\dfrac{2}{x+1}=\dfrac{2019}{2021}\)
\(\Leftrightarrow\dfrac{2}{x+1}=1-\dfrac{2019}{2021}\)
\(\Leftrightarrow\dfrac{2}{x+1}=\dfrac{2}{2021}\)
\(\Rightarrow x+1=2021\)
\(\Rightarrow x=2020\)