
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


6:
\(2^{225}=\left(2^3\right)^{75}=8^{75}\)
\(3^{150}=\left(3^2\right)^{75}=9^{75}\)
mà 8<9
nên \(2^{225}< 3^{150}\)
4: \(\left|5x+3\right|>=0\forall x\)
=>\(-\left|5x+3\right|< =0\forall x\)
=>\(-\left|5x+3\right|+5< =5\forall x\)
Dấu = xảy ra khi 5x+3=0
=>x=-3/5
1:
\(\left(2x+1\right)^4>=0\)
=>\(\left(2x+1\right)^4+2>=2\)
=>\(M=\dfrac{3}{\left(2x+1\right)^4+2}< =\dfrac{3}{2}\)
Dấu = xảy ra khi 2x+1=0
=>x=-1/2

1: \(\sqrt{11}\) là số vô tỉ
2:
a: 4,9(18)=4,91818...
mà 4,91818<4,928
nên 4,9(18)<4,928
b: 4,315<4,318
=>-4,315>-4,318
=>-4,315...>-4,318...
c: \(\sqrt{3}=\sqrt{\dfrac{6}{2}}< \sqrt{\dfrac{7}{2}}\)
3:
a: \(6=\sqrt{3};-1,7=-\sqrt{2,89}\)
0<2,89<3
=>\(0< \sqrt{2,89}< \sqrt{3}\)
=>\(-\sqrt{3}< -\sqrt{2,89}< 0\)
0<35<36<47
=>\(0< \sqrt{35}< \sqrt{36}< \sqrt{47}\)
=>\(-\sqrt{3}< -\sqrt{2,89}< 0< \sqrt{35}< \sqrt{36}< \sqrt{47}\)
=>\(-\sqrt{3}< -\sqrt{2,89}< 0< \sqrt{35}< 6< \sqrt{47}\)
b: \(-\sqrt{2\dfrac{1}{3}}=-\sqrt{2,\left(3\right)}\)
\(-1,5=-\sqrt{2,25}\)
2,25<2,3<2,(3)
=>\(\sqrt{2.25}< \sqrt{2.3}< \sqrt{2.\left(3\right)}\)
=>\(0>-1.5>-\sqrt{2.3}>-\sqrt{2\dfrac{1}{3}}\)
\(0< \sqrt{5\dfrac{1}{6}}=\sqrt{5,1\left(6\right)}< \sqrt{5,3}\)
=>\(\sqrt{5.3}>\sqrt{5\dfrac{1}{6}}>0>-1.5>-\sqrt{2.3}>-\sqrt{2\dfrac{1}{3}}\)

a) Ta có:
\(\widehat{yOu}+\widehat{xOy}=180^o\) (kề bù)
\(\Rightarrow\widehat{yOu}=180^o-\widehat{xOy}\)
\(\Rightarrow\widehat{yOu}=180^o-60^o=120^o\)
Mà: \(\widehat{xOt}+\widehat{tOu}=180^o\) (kề bù)
\(\Rightarrow\widehat{xOt}=180^o-\widehat{tOu}\)
\(\Rightarrow\widehat{xOt}=180^o-30^o=150^o\)
b) Ta có:
\(\widehat{xOy}+\widehat{yOt}+\widehat{tOu}=\widehat{xOu}\)
\(\Rightarrow\widehat{yOt}=\widehat{xOu}-\widehat{xOy}-\widehat{tOu}\)
\(\Rightarrow\widehat{yOt}=180^o-60^o-30^o\)
\(\Rightarrow\widehat{yOt}=90^o\)

`#1231.2021`
`1.`
Ta có:
`y` tỉ lệ nghịch với `x` theo hệ số tỉ lệ `-4`
`=> y = (-4)/x` `(1)`
`x` tỉ lệ nghịch với `z` theo hệ số tỉ lệ `3/4`
`=> x = 3/4 \div z` `(2)`
Thay `(2)` vào `(1)`
`=> y = (-4)/(3/4 \div z) => y = -16/3 * z`
Vậy, `y` và `z` tỉ lệ thuận với nhau theo hệ số tỉ lệ `-16/3`
`=> A.`



Bài 16
a) \(A=\dfrac{n+1}{n+2}\)
Gọi ƯCLN(n+1;n+2) là x ( \(x\in N\) *)
\(\Rightarrow\) \(\left\{{}\begin{matrix}\left(n+1\right)⋮x\\\left(n+2\right)⋮x\end{matrix}\right.\)
\(\Rightarrow\) \(\left(n+2\right)-\left(n+1\right)\) \(⋮x\)
\(\Rightarrow\) \(1\) \(⋮x\)
\(\Rightarrow\) x = 1 \(\Rightarrow\) ƯCLN(n+1;n+2)=1
Vậy A là phân số tối giản ( vì có ƯCLN = 1)
b) \(B=\dfrac{n+1}{3n+4}\)
Gọi ƯCLN(n+1;3n+4) là d ( \(d\in N\) *)
\(\Rightarrow\) \(\left\{{}\begin{matrix}n+1⋮d\\3n+4⋮d\end{matrix}\right.\)
\(\Rightarrow\) \(\left\{{}\begin{matrix}3n+3⋮d\\3n+4⋮d\end{matrix}\right.\)
\(\Rightarrow\) (3n+4)-(3n+3) chia hết cho d
\(\Rightarrow\) \(1⋮d\)
\(\Rightarrow\) d =1
Vậy B là phân số tối giản.
Mấy phần kia tương tự
c: Gọi d=ƯCLN(3n+2;5n+3)
=>3n+2 chia hết cho d và 5n+3 chia hết cho d
=>15n+10 chia hết cho d và 15n+9 chia hết cho d
=>1 chia hết cho d
=>ƯCLN(3n+2;5n+3)=1
=>PSTG
d: Gọi d=ƯCLN(12n+1;30n+2)
=>12n+1 và 30n+2 đều chia hết cho d
=>60n+5 chia hết cho d và 60n+4 chia hết cho d
=>1 chia hết cho d
=>d=1
=>PSTG

 giúp em với ạ em đang cần gấp ạ. Bài nào làm đc trc thì làm trc giúp em với ạ
giúp em với ạ em đang cần gấp ạ. Bài nào làm đc trc thì làm trc giúp em với ạ giúp em 3 bài này với ạ em đang cần gấp chiều em hc ròi ạ ai làm đc bài nào thì gửi luôn giúp em ạ
giúp em 3 bài này với ạ em đang cần gấp chiều em hc ròi ạ ai làm đc bài nào thì gửi luôn giúp em ạ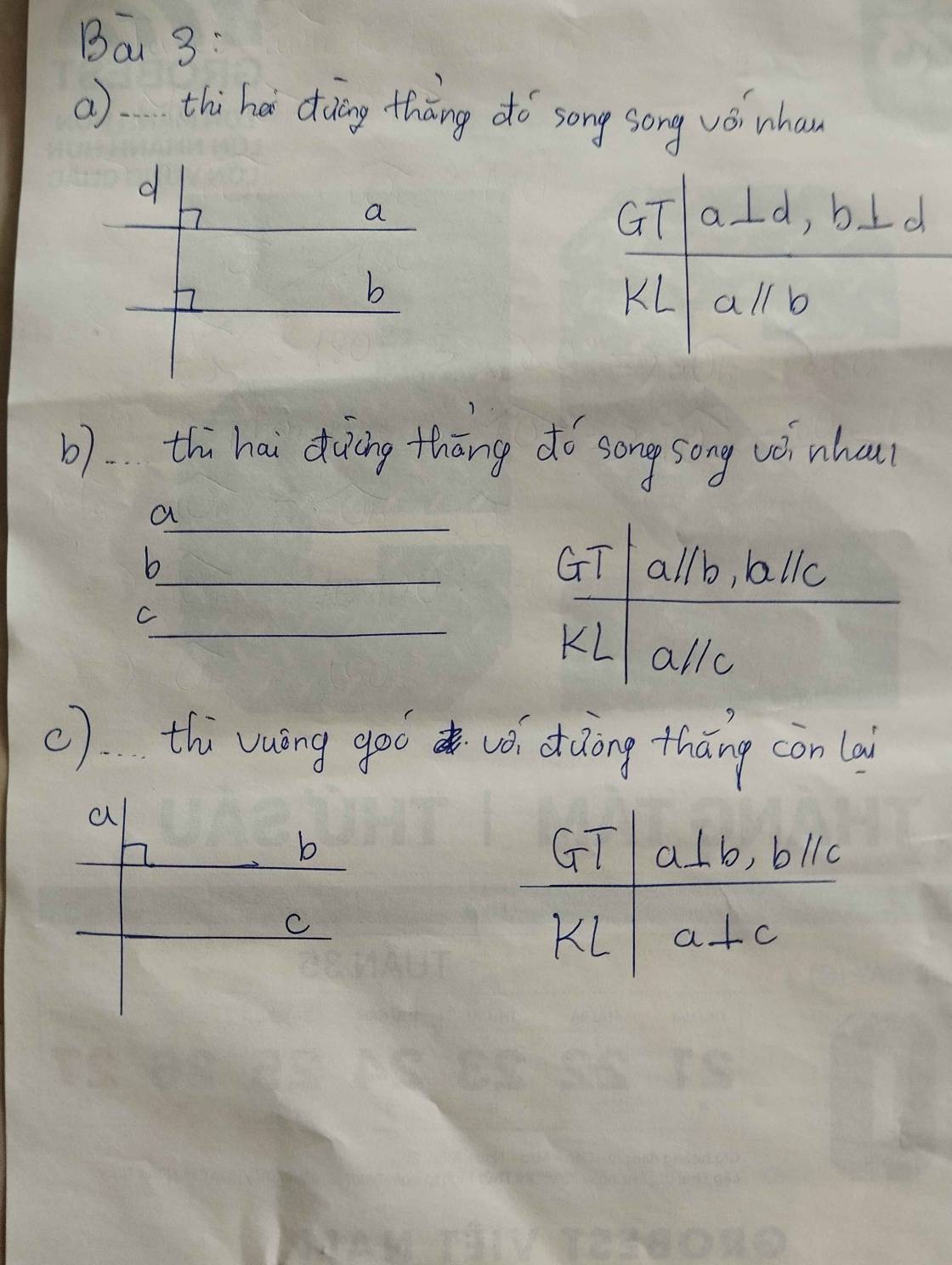

 giúp em với ạ em cần gấp ạ
giúp em với ạ em cần gấp ạ giúp em với ạ em cần gấp ạ
giúp em với ạ em cần gấp ạ

 giúp em với ạ nhanh em cần ạ :33
giúp em với ạ nhanh em cần ạ :33 giúp em với ạ em đang cần gấp ạ
giúp em với ạ em đang cần gấp ạ giúp em với ạ em đang cần gấp ạ :(
giúp em với ạ em đang cần gấp ạ :(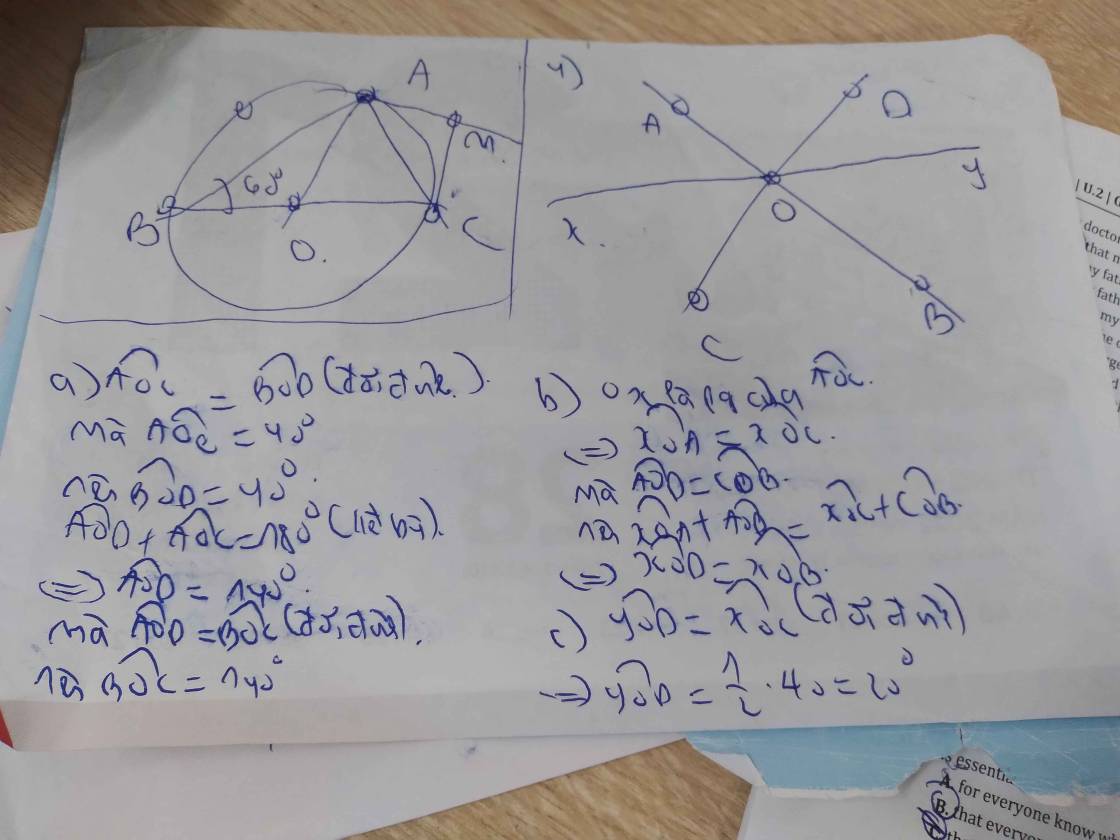

`Answer:`
\(A=\frac{1}{2}x^2y^3-4xy+5\)
Thay `x=-2` và `y=2/3` vào đa thức `A`, ta được:
\(A=\frac{1}{2}.\left(-2\right)^2.\frac{2}{3}^3-4.\left(-2\right).\frac{2}{3}+5\)
\(=\frac{1}{2}.4.\frac{8}{27}-4.-2.\frac{2}{3}+5\)
\(=\frac{16}{27}-\frac{-16}{3}+5\)
\(=\frac{295}{27}\)