K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NV
Nguyễn Việt Lâm
Giáo viên
10 tháng 4 2021
d. \(\dfrac{\pi}{2}< a;b< \pi\Rightarrow sina>0;sinb>0\)
\(sina=\sqrt{1-cos^2a}=\dfrac{4}{5}\Rightarrow tana=\dfrac{sina}{cosa}=-\dfrac{4}{3}\)
\(sinb=\sqrt{1-cos^2b}=\dfrac{5}{13}\Rightarrow tanb=-\dfrac{5}{12}\)
Vậy:
\(sin\left(a-b\right)=sina.cosb-cosa.sinb=\dfrac{4}{5}.\left(-\dfrac{12}{13}\right)-\left(-\dfrac{3}{5}\right)\left(\dfrac{5}{13}\right)=...\)
\(cos\left(a-b\right)=cosa.cosb-sina.sinb=...\) (bạn tự thay số bấm máy)
\(tan\left(a+b\right)=\dfrac{tana+tanb}{1-tana.tanb}=...\)
\(cot\left(a+b\right)=\dfrac{1}{tan\left(a+b\right)}=\dfrac{1-tana.tanb}{tana+tanb}=...\)
e.
\(0< y< \dfrac{\pi}{2}\Rightarrow cosy>0\Rightarrow cosy=\sqrt{1-sin^2y}=\dfrac{4}{5}\)
\(\Rightarrow tany=\dfrac{siny}{cosy}=\dfrac{3}{4}\)
Vậy: \(tan\left(x+y\right)=\dfrac{tanx+tany}{1-tanx.tany}=...\)
\(cot\left(x-y\right)=\dfrac{1}{tan\left(x-y\right)}=\dfrac{1+tanx.tany}{tanx-tany}=...\)
DT
1
29 tháng 12 2021
Câu 5:
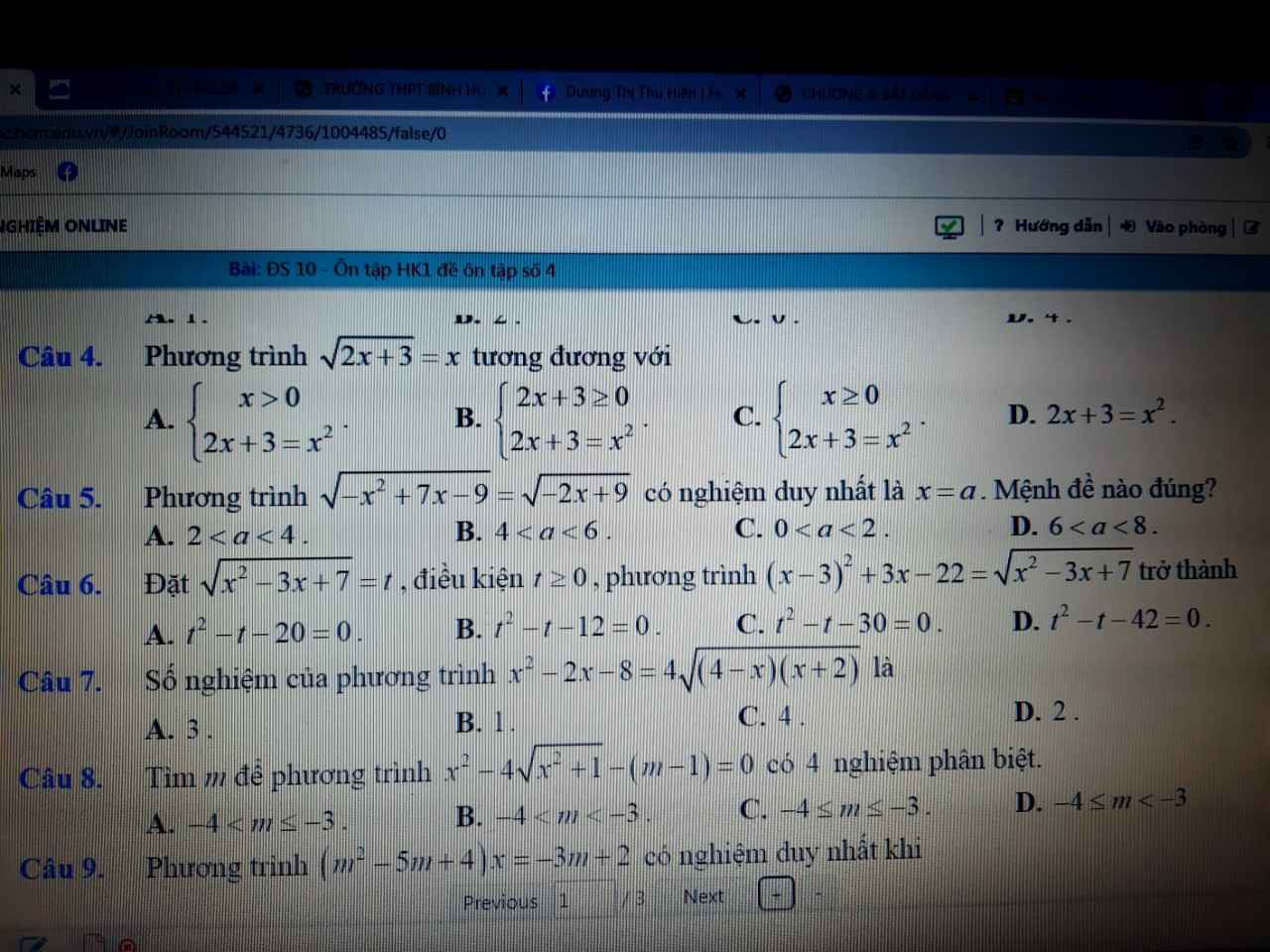
\(\Leftrightarrow-x^2+7x-9+2x-9=0\)
\(\Leftrightarrow x^2-9x+18=0\)
=>x=3
=>Chọn A
HP
24 tháng 5 2021
12.1
Giả sử \(G=\left(m;2m-2\right)\left(m\in R\right)\)
\(\Rightarrow\left\{{}\begin{matrix}x_H=2x_E-x_G=6-m\\y_H=2y_E-y_G=2-2m\end{matrix}\right.\)
\(\Rightarrow H=\left(6-m;2-2m\right)\)
Mà \(H\in d_2\Rightarrow6-m+2-2m+3=0\Leftrightarrow m=\dfrac{11}{3}\)
\(\Rightarrow G=\left(\dfrac{11}{3};\dfrac{16}{3}\right)\)
\(\Rightarrow\Delta:8x-y-24=0\)
HP
24 tháng 5 2021
12.2
Giả sử \(A=\left(m;-m-1\right)\left(m\in R\right)\)
Ta có: \(\vec{AM}=\dfrac{1}{3}\vec{MB}\)
\(\Rightarrow\left\{{}\begin{matrix}x_M-x_A=\dfrac{1}{3}\left(x_B-x_M\right)\\y_M-y_A=\dfrac{1}{3}\left(y_B-y_M\right)\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}1-m=\dfrac{1}{3}\left(x_B-1\right)\\m+1=\dfrac{1}{3}.y_B\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_B=4-3m\\y_B=3m+3\end{matrix}\right.\)
\(\Rightarrow B=\left(4-3m;3m+3\right)\)
Mà \(B\in d_2\Rightarrow4-3m-2\left(3m+3\right)+2=0\Leftrightarrow m=0\)
\(\Rightarrow A=\left(0;-1\right)\)
\(\Rightarrow d:x-y-1=0\)
8 tháng 5 2021
a, Ta có : \(\sin^2x+\cos^2x=1\)
\(\Rightarrow\sin x=\sqrt{1-\cos^2x}=\left|\dfrac{\sqrt{15}}{4}\right|\)
Mà \(0< x< \dfrac{\pi}{2}\)
\(\Rightarrow\sin x=\dfrac{\sqrt{15}}{4}\)
Ta lại có : \(\left\{{}\begin{matrix}\sin2x=2\sin x\cos x=\dfrac{\sqrt{15}}{8}\\\cos2x=2\cos^2x-1=-\dfrac{7}{8}\end{matrix}\right.\)
Vậy ...
c, Ta có : \(\tan2x=\dfrac{2\tan x}{1-\tan^2x}=\dfrac{4}{3}=\dfrac{\sin2x}{\cos2x}\)
- Ta có HPT : \(\left\{{}\begin{matrix}\sin^22x+\cos^22x=1\\3\sin2x-4\cos2x=0\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}\sin2x=\left|\dfrac{4}{5}\right|\\\cos2x=\left|\dfrac{3}{5}\right|\end{matrix}\right.\)
Lại có : \(\pi< x< \dfrac{3}{2}\pi\)
\(\Rightarrow\left\{{}\begin{matrix}\sin2x=\dfrac{4}{5}\\\cos2x=\dfrac{3}{5}\end{matrix}\right.\)
Vậy ...
NV
Nguyễn Việt Lâm
Giáo viên
5 tháng 5 2021
\(sin\left(\dfrac{\pi}{2}-x\right)+cot^2x=cosx+\dfrac{cos^2x}{sin^2x}=cosx+\dfrac{cos^2x}{1-cos^2x}=a+\dfrac{a^2}{1-a^2}\)
\(=\dfrac{-a^3+a^2+a}{1-a^2}\)
\(\Rightarrow\left\{{}\begin{matrix}m=-1\\n=1\\\end{matrix}\right.\) \(\Rightarrow P=-3\)
26 tháng 11 2021
Câu 12:
b) mx2+2(m+3)x+m=0
(a = m; b = 2(m+3); c = m)
PT có 2 nghiệm cùng dấu ⇔ \([_{P>0}^{\Delta\ge0}\)
⇔ \([_{\dfrac{c}{a}}^{b^2-4ac}\)⇔\([_{\dfrac{m}{m}>0}^{[2\left(m+3\right)]^2-4.m.m\ge0}\)⇔\([_{1>0\left(LĐ\right)}^{4\left(m+3\right)^2-4m^2\ge0}\)⇔\(4\left(m^2+6m+9\right)-4m^2\ge0\)
⇔4m2+24m+36-4m2≥0 ⇔ 24m+36≥0⇔ m ≥ \(-\dfrac{3}{2}\)
Vậy với m ≥\(-\dfrac{3}{2}\)thì PT có 2 nghiệm cùng dấu.
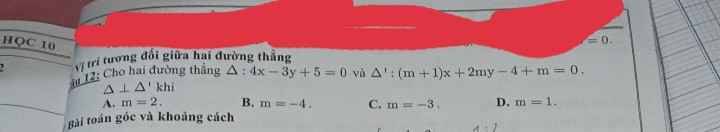
10.
ĐKXĐ: \(\left\{{}\begin{matrix}2x+10\ge0\\-x-5\ge0\\-2x-7\ge0\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x\ge-5\\x\le-5\\x\le-\dfrac{7}{2}\end{matrix}\right.\)
\(\Rightarrow\) Không tồn tại x thỏa mãn
Vậy BPT đã cho vô nghiệm
12.
\(\Leftrightarrow\dfrac{3}{x+3}-\dfrac{3x+4}{x\left(x+4\right)}\ge0\Leftrightarrow\dfrac{-x-12}{x\left(x+3\right)\left(x+4\right)}\ge0\)
\(\Rightarrow\left[{}\begin{matrix}-12\le x< -4\\-3< x< 0\end{matrix}\right.\)
\(x=\left\{-12;-11;...-5;-2;-1\right\}\) có 10 số nguyên