PL
3
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NM
2
25 tháng 11 2023
ĐKXĐ: \(x\in R\)
\(3x^2-5x+6=2x\cdot\sqrt{x^2-x+2}\)
=>\(3x^2-6x+x-2+8=2\cdot\sqrt{x^4-x^3+2x^2}\)
=>\(\left(x-2\right)\left(3x+1\right)=2\cdot\left(\sqrt{x^4-x^3+2x^2}-4\right)\)
\(\Leftrightarrow\left(x-2\right)\left(3x+1\right)=2\cdot\dfrac{x^4-x^3+2x^2-16}{\sqrt{x^4-x^3+2x^2}+4}\)
=>\(\left(x-2\right)\left(3x+1\right)=2\cdot\dfrac{x^4-2x^3+x^3-2x^2+4x^2-8x+8x-16}{\sqrt{x^4-x^3+2x^2}+4}\)
=>\(\left(x-2\right)\left(3x+1\right)=\dfrac{2\left(x-2\right)\left(x^3+x^2+4x+8\right)}{\sqrt{x^4-x^3+2x^2}+4}\)
=>\(\left(x-2\right)\left[\left(3x+1\right)-\dfrac{2\left(x^3+x^2+4x+8\right)}{\sqrt{x^4-x^3+2x^2}+4}\right]=0\)
=>x-2=0
=>x=2(nhận)
25 tháng 11 2023
\(3x^2-5x+6=2x\sqrt{x^2-x+2}\)
\(\Leftrightarrow\left[x^2-2x\sqrt{x^2-x+2}+\left(x^2-x+2\right)\right]+\left(x^2-4x+4\right)=0\)
\(\Leftrightarrow\left(x-\sqrt{x^2-x+2}\right)^2+\left(x-2\right)^2=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=\sqrt{x^2-x+2}\\x-2=0\end{matrix}\right.\Leftrightarrow x=2\)
Thử lại ta thấy nghiệm \(x=2\) thỏa phương trình ban đầu.
NP
1
NV
Nguyễn Việt Lâm
Giáo viên
26 tháng 7 2021
ĐKXĐ: \(x\ge-\dfrac{4}{5}\)
Đặt \(\sqrt{5x+4}=t\ge0\Rightarrow x=\dfrac{t^2-4}{5}\)
Pt trở thành:
\(\dfrac{t^2-4}{5}-t=2\)
\(\Leftrightarrow t^2-5t-14=0\Rightarrow\left[{}\begin{matrix}t=7\\t=-2< 0\left(loại\right)\end{matrix}\right.\)
\(\Rightarrow\sqrt{5x+4}=7\)
\(\Rightarrow5x+4=49\)
\(\Rightarrow x=9\)
LA
29 tháng 3 2022
1. 3x( x - 2 ) - ( x - 2 ) = 0
<=> ( x-2).(3x-1) = 0 => x = 2 hoặc x = \(\dfrac{1}{3}\)
2. x( x-1 ) ( x2 + x + 1 ) - 4( x - 1 )
<=> ( x - 1 ).( x (x^2 + x + 1 ) - 4 ) = 0
(phần này tui giải được x = 1 thôi còn bên kia giải ko ra nha )
3 \(\left\{{}\begin{matrix}\sqrt{5}x-2y=7\\\sqrt{5}x-5y=10\end{matrix}\right.\)<=> \(\left\{{}\begin{matrix}y=-1\\x=\sqrt{5}\end{matrix}\right.\)
AH
29 tháng 3 2022
\(1. 3x^2 - 7x +2=0\)
=>\(Δ=(-7)^2 - 4.3.2\)
\(= 49-24 = 25\)
Vì 25>0 suy ra phương trình có 2 nghiệm phân biệt:
\(x_1\)=\(\dfrac{-\left(-7\right)+\sqrt{25}}{2.3}=\dfrac{7+5}{6}=2\)
\(x_2\)=\(\dfrac{-\left(-7\right)-\sqrt{25}}{2.3}=\dfrac{7-5}{6}=\dfrac{1}{3}\)
13 tháng 6 2020
\(PT\Leftrightarrow6\left(x+\sqrt{6x^2+6}\right)=-5x^2-2\sqrt{5}x-1\)
\(\Leftrightarrow6\left(x+\sqrt{6x^2+6}\right)=-\left(\sqrt{5}x+1\right)^2\)
\(\Rightarrow x+\sqrt{6x^2+6}\le0\)
KT
1
28 tháng 10 2023
a: ĐKXĐ: \(\left\{{}\begin{matrix}x-3>=0\\5-x>=0\end{matrix}\right.\)
=>3<=x<=5
\(\sqrt{x-3}+\sqrt{5-x}=2\)
=>\(\sqrt{x-3}-1+\sqrt{5-x}-1=0\)
=>\(\dfrac{x-3-1}{\sqrt{x-3}+1}+\dfrac{5-x-1}{\sqrt{5-x}+1}=0\)
=>\(\left(x-4\right)\left(\dfrac{1}{\sqrt{x-3}+1}-\dfrac{1}{\sqrt{5-x}+1}\right)=0\)
=>x-4=0
=>x=4
DQ
4
10 tháng 9 2016
a/ PT <=> (x2 - 6x + 9) + (x - \(\sqrt{3x}\)) + (3 - \(\sqrt{3x}\)) = 0
<=> (\(\sqrt{x}-\sqrt{3}\))(\(\sqrt{3}x+x\sqrt{x}-3\sqrt{x}-3\sqrt{3}\)) + √x(\(\sqrt{x}-\sqrt{3}\)) + \(\sqrt{3}\left(\sqrt{3}-\sqrt{x}\right)\)= 0
<=> x = 3
BH
1
9 tháng 3 2022
a: \(\Leftrightarrow\left(x-\sqrt{5}\right)^2=0\)
\(\Leftrightarrow x-\sqrt{5}=0\)
hay \(x=\sqrt{5}\)
b: \(\Leftrightarrow4x^4-9x^2+4x^2-9=0\)
\(\Leftrightarrow4x^2-9=0\)
=>x=3/2hoặc x=-3/2
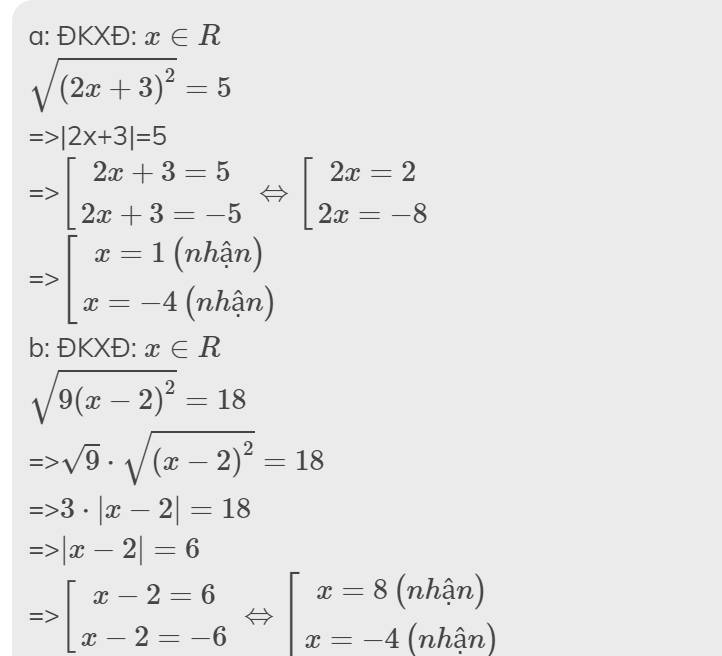
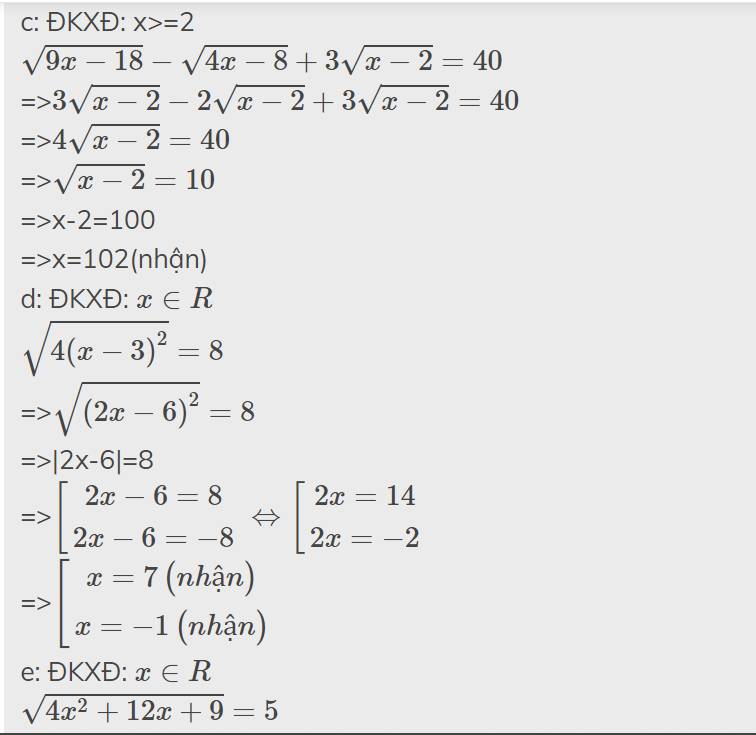
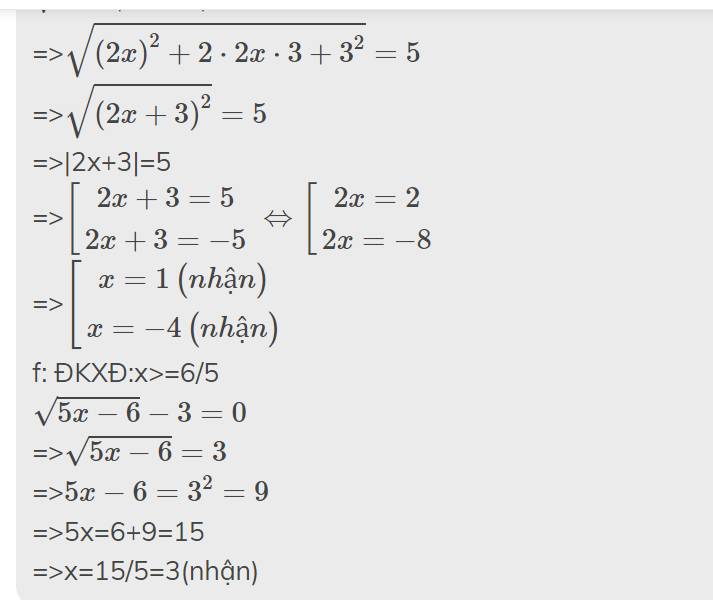
\(Pt\Leftrightarrow\sqrt[5]{27}x^{10}+2\sqrt[5]{27}=5x^6\)
Áp dụng bất đẳng thức AM-GM cho 5 số dương:
\(VT=\frac{\sqrt[5]{27}x^{10}}{3}+\frac{\sqrt[5]{27}x^{10}}{3}+\frac{\sqrt[5]{27}x^{10}}{3}+\sqrt[5]{27}+\sqrt[5]{27}\ge5\sqrt[5]{\frac{27x^{30}}{27}}=5x^6=VF\)
Dấu = xảy ra khi \(\frac{\sqrt[5]{27}x^{10}}{3}=\sqrt[5]{27}\Leftrightarrow x^{10}=3\Leftrightarrow\orbr{\begin{cases}x=\sqrt[10]{3}\\x=-\sqrt[10]{3}\end{cases}}\)
mk có cách lm = mt chứ tính= tay thì chịu