K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
DU
5
26 tháng 1 2021
\(\left(8x+5\right)\left(8x+7\right)\left(8x+6\right)^2=72\)
Đặt \(8x+5=t\left(t\ge0\right)\)
\(t\left(t+2\right)\left(t+1\right)^2-72=0\)
\(\Leftrightarrow t\left(t+1\right)\left(t+2\right)\left(t+1\right)-72=0\)
\(\Leftrightarrow\left(t^2+t\right)\left(t^2+3t+2\right)-72=0\)
\(\Leftrightarrow t^4+3t^3+2t^2+t^3+3t^2+2t-72=0\)
\(\Leftrightarrow t^4+4t^3+5t^2+2t-72=0\)
\(\Leftrightarrow\left(t^2+2t+9\ne0\right)\left(t+4\right)\left(t-2\right)=0\Leftrightarrow t=-4;2\)
hay \(8x+5=-4\Leftrightarrow x=-\frac{9}{8}\)( trường hợp 1 )
\(8x+5=2\Leftrightarrow x=-\frac{3}{8}\)( trưởng hợp 2 )
Vậy tập nghiệm của phương trình là S = { -9/8 ; -3/8 }
26 tháng 1 2021
\(\left(8x+5\right)\cdot\left(8x+7\right)\cdot\left(8x+6\right)^2=72\)
Đặt \(t=8x+6\)
\(Pt\Leftrightarrow\left(t-1\right)\left(t+1\right)t^2-72=0\)
\(\Leftrightarrow\left(t^2-1\right)t^2-72=0\Leftrightarrow t^4-t^2-72=0\)
\(\Leftrightarrow\left(t^2-9\right)\left(t^2+8\right)=0\Leftrightarrow\orbr{\begin{cases}t^2=9\\t^2=-8\end{cases}\Leftrightarrow\orbr{\begin{cases}t=3\\t=-3\end{cases}}}\)
\(\Leftrightarrow\orbr{\begin{cases}8x+6=3\\8x+6=-3\end{cases}\Leftrightarrow\orbr{\begin{cases}x=-\frac{3}{8}\\x=-\frac{9}{8}\end{cases}}}\)
Vậy....
TN
3 tháng 3 2016
x=-9;
x = -(căn bậc hai(3)*căn bậc hai(37)*i+27)/6;
x = (căn bậc hai(3)*căn bậc hai(37)*i-27)/6;
29 tháng 8 2021
a: Ta có: \(3x+5\le4x-9\)
\(\Leftrightarrow-x\le-14\)
\(\Leftrightarrow x\ge14\)
b: Ta có: \(6-2x< 6-x\)
\(\Leftrightarrow-x< 0\)
hay x>0
c: Ta có: \(7\left(x-1\right)+5>-3x\)
\(\Leftrightarrow7x-7+5+3x>0\)
\(\Leftrightarrow10x>2\)
hay \(x>\dfrac{1}{5}\)
TB
1
23 tháng 11 2017
3(x+5)(x+6)(x+7)=8(x+6)-48 (1)
Đặt x+6=t
(1) <=> 3t(t-1)(t+1)=8t-48
<=> 3t3-11t+48=0
<=> (x+3)(3x2-9x+16) =0
Từ sau tự làm đi nghại ghi
18 tháng 3 2021
x2-4x+7 = 0 ⇔ x2 -4x + 4 + 3 = 0
⇔ (x-2)2+3=0 ⇔ (x-2)2=-3 (vô lí)
Vậy pt vô nghiệm
18 tháng 3 2021
*Chứng minh phương trình \(x^2-4x+7=0\) vô nghiệm
Ta có: \(x^2-4x+7=0\)
\(\Leftrightarrow x^2-4x+4+3=0\)
\(\Leftrightarrow\left(x-2\right)^2+3=0\)
mà \(\left(x-2\right)^2+3\ge3>0\forall x\)
nên \(x\in\varnothing\)(đpcm)
NT
0
10 tháng 5 2023
a:=>3x=15
=>x=5
b: =>8-11x<52
=>-11x<44
=>x>-4
c: \(VT=\left(\dfrac{x^2-\left(x-6\right)^2}{x\left(x+6\right)\left(x-6\right)}\right)\cdot\dfrac{x\left(x+6\right)}{2x-6}+\dfrac{x}{6-x}\)
\(=\dfrac{12x-36}{2x-6}\cdot\dfrac{1}{x-6}-\dfrac{x}{x-6}=\dfrac{6}{x-6}-\dfrac{x}{x-6}=-1\)
PT
1
CM
13 tháng 8 2018
8x + 3(x + 1) > 5x – (2x – 6)
⇔ 8x + 3x + 3 > 5x – 2x + 6
⇔ 8x + 3x – 5x + 2x > 6 – 3 (Chuyển vế, đổi dấu)
⇔ 8x > 3
⇔ 
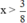(Chia cả hai vế cho 8 > 0, BPT không đổi chiều)
Vậy bất phương trình có nghiệm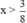 
9 tháng 5 2021
\(\dfrac{x}{2x-6}-\dfrac{x}{2x+2}=\dfrac{2x}{\left(x+1\right)\left(x-3\right)}\left(ĐKXĐ:x\ne-1,x\ne3\right)\)
\(\Leftrightarrow\dfrac{x}{2\left(x-3\right)}-\dfrac{x}{2\left(x+1\right)}=\dfrac{2x}{\left(x+1\right)\left(x-3\right)}\)
\(\Leftrightarrow\dfrac{x\left(x+1\right)}{2\left(x+1\right)\left(x-3\right)}-\dfrac{x\left(x-3\right)}{2\left(x+1\right)\left(x-3\right)}=\dfrac{2x\cdot2}{2\left(x+1\right)\left(x-3\right)}\)
\(\Rightarrow x\left(x+1\right)-x\left(x-3\right)=4x\)
\(\Leftrightarrow x^2+x-x^2+3x=4x\)
\(\Leftrightarrow x^2+x-x^2+3x-4x=0\)
\(\Leftrightarrow0x=0\)
Phương trình có vô số nghiệm , trừ x = -1,x = 3
Vậy ...
\(\dfrac{12x+1}{12}< \dfrac{9x+1}{3}-\dfrac{8x+1}{4}\)
\(\Leftrightarrow12\cdot\dfrac{12x+1}{12}< 12\cdot\dfrac{9x+1}{3}-12\cdot\dfrac{8x+1}{4}\)
\(\Leftrightarrow12x+1< 4\left(9x+1\right)-3\left(8x+1\right)\)
\(\Leftrightarrow12x+1< 36x+4-24x-3\)
\(\Leftrightarrow12x+1< 12x+1\)
\(\Leftrightarrow12x-12x< 1-1\)
\(\Leftrightarrow0x< 0\)
Vậy S = {x | x \(\in R\)}
(x-6 ) (8x -7)