
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


5x3 – x2 – 5x + 1 = 0
⇔ x2(5x – 1) – (5x – 1) = 0
⇔ (x2 – 1)(5x – 1) = 0
⇔ (x – 1)(x + 1)(5x – 1) = 0
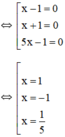
Vậy phương trình có tập nghiệm 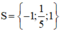

a) 1,2x3 – x2 – 0,2x = 0
⇔ 0,2x.(6x2 – 5x – 1) = 0
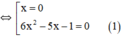
Giải (1): 6x2 – 5x – 1 = 0
có a = 6; b = -5; c = -1
⇒ a + b + c = 0
⇒ (1) có hai nghiệm x1 = 1 và x2 = c/a = -1/6.
Vậy phương trình ban đầu có tập nghiệm 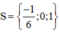
b) 5x3 – x2 – 5x + 1 = 0
⇔ x2(5x – 1) – (5x – 1) = 0
⇔ (x2 – 1)(5x – 1) = 0
⇔ (x – 1)(x + 1)(5x – 1) = 0
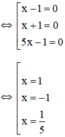
Vậy phương trình có tập nghiệm 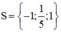

ĐKXĐ: ...
\(\Leftrightarrow x^2-6x+9+x+1-4\sqrt{x+1}+4=0\)
\(\Leftrightarrow\left(x-3\right)^2+\left(\sqrt{x+1}-2\right)^2=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}x-3=0\\\sqrt{x+1}-2=0\end{matrix}\right.\) \(\Rightarrow x=3\)

\(\sqrt{\frac{5x+7}{x+3}}=4\)
ĐKXĐ: \(\left[{}\begin{matrix}x\ge-\frac{7}{5}\\x< -3\end{matrix}\right.\)
\(\Leftrightarrow\frac{5x+7}{x+3}=16\\ \Rightarrow5x+7=16\left(x+3\right)\\ \Leftrightarrow5x+7=16x+48\\ \Leftrightarrow5x-16x=48-7\\ \Leftrightarrow-11x=41\Leftrightarrow x=-\frac{41}{11}\)(T/m ĐKXĐ)
Vậy \(x=-\frac{41}{11}\)

a: Ta có: \(\left\{{}\begin{matrix}3x+2y=14\\5x+3y=1\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}15x+10y=70\\15x+9y=3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=67\\3x=14-2y=14-2\cdot67=-120\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-40\\y=67\end{matrix}\right.\)
b: Ta có: \(\left\{{}\begin{matrix}-x+2y-6=0\\5x-3y-5=0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}-x+2y=6\\5x-3y=5\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}-5x+10y=30\\5x-3y=5\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}7y=35\\2y-x=6\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=5\\y=4\end{matrix}\right.\)


\(x^2-2x-\sqrt{3}+1=0\)
\(\Delta=b^2-4ac=4-4\left(-\sqrt{3}+1\right)=4\sqrt{3}>0\)
\(\rightarrow\)Phương trình có 2 nghiệm phân biệt
Theo vi-ét ta có :
\(\left\{{}\begin{matrix}S=x_1+x_2=-\dfrac{b}{a}=2\\P=x_1x_2=\dfrac{c}{a}=-\sqrt{3}+1\end{matrix}\right.\)
\(M=x_1^2x_2^2-2x_1x_2-x_1-x_2\)
\(=\left(x_1x_2\right)^2-2x_1x_2-\left(x_1+x_2\right)\)
\(=\left(-\sqrt{3}+1\right)^2-2\left(-\sqrt{3}+1\right)-2\)
\(=0\)

pt bậc 4 => có 4 nghiệm.
bấm máy tính tìm nghiệm đẹp (-2 và 3). Chia sơ đồ hoocne.
2 nghiệm đẹp (-2 và 3) được rồi, còn 2 nghiệm còn lại thì giải pt bậc 2 là ra.
kq: x=-2, x=3, x=1/3 , x=-1/2
Ta có \(6x^4-5x^3-38x^2-5x+6=0\Leftrightarrow6x^4+12x^3-17x^3-34x^2-4x^2-8x+3x+6=0\Leftrightarrow6x^3\left(x+2\right)-17x^2\left(x+2\right)-4x\left(x+2\right)+3\left(x+2\right)=0\Leftrightarrow\left(x+2\right)\left(6x^3-17x^2-4x+3\right)=0\Leftrightarrow\left(x+2\right)\left(6x^3-18x^2+x^2-3x-x+3\right)=0\Leftrightarrow\left(x+2\right)\left[6x^2\left(x-3\right)+x\left(x-3\right)-\left(x-3\right)\right]=0\Leftrightarrow\left(x+2\right)\left(x-3\right)\left(6x^2+x-1\right)=0\Leftrightarrow\left(x+2\right)\left(x-3\right)\left(6x^2-2x+3x-1\right)=0\Leftrightarrow\left(x+2\right)\left(x-3\right)\left[2x\left(3x-1\right)+\left(3x-1\right)\right]=0\Leftrightarrow\left(x+2\right)\left(x-3\right)\left(3x-1\right)\left(2x+1\right)=0\Leftrightarrow\)\(\left[{}\begin{matrix}x+2=0\\x-3=0\\3x-1=0\\2x+1=0\end{matrix}\right.\)\(\Leftrightarrow\)\(\left[{}\begin{matrix}x=-2\\x=3\\x=\dfrac{1}{3}\\x=-\dfrac{1}{2}\end{matrix}\right.\)
Vậy S={\(-\dfrac{1}{2};-2;\dfrac{1}{3};3\)}