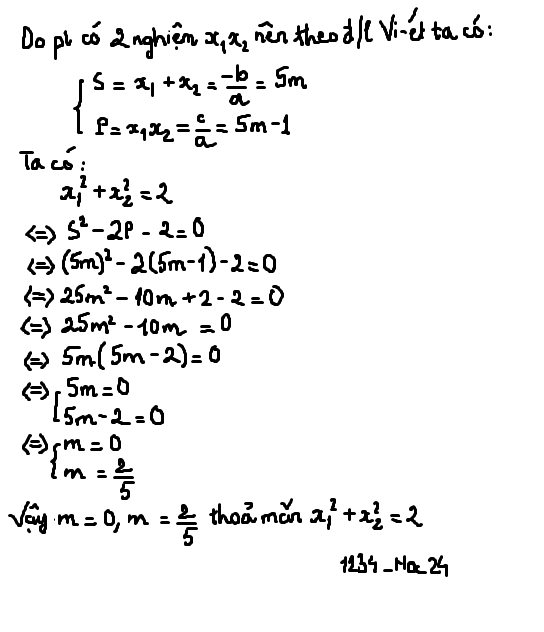Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a=1; b=-4; c=-m^2+3
Δ=(-4)^2-4*1*(-m^2+3)
=16+4m^2-12=4m^2+4>=4>0
=>Phương trình luôn có hai nghiệm phân biệt
5x1+x2=0 và x1+x2=4
=>4x1=-4 và x1+x2=4
=>x1=-1 và x2=5
x1x2=-m^2+3
=>-m^2+3=-5
=>m^2-3=5
=>m^2=8
=>\(m=\pm2\sqrt{2}\)

\(ac=-m^2-1< 0;\forall m\Rightarrow\) phương trình luôn có 2 nghiệm trái dấu với mọi m
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=m\\x_1x_2=-m^2-1\end{matrix}\right.\)
\(x_1^2+x_2^2=3\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=3\)
\(\Leftrightarrow m^2-2\left(-m^2-1\right)=3\)
\(\Leftrightarrow3m^2=1\)
\(\Leftrightarrow m^2=\dfrac{1}{3}\)
\(\Leftrightarrow m=\pm\dfrac{1}{\sqrt{3}}\)
xét delta
m2 + 4m2 + 4 = 5m2 + 4 > 0
=> phương trình luôn có 2 nghiệm x1x2
theo Vi-ét ta có:
\(\left\{{}\begin{matrix}x1+x2=m\\x1x2=-m^2-1\end{matrix}\right.\)
x12 + x22 = 3
<=> ( x1 +x2 )2 - 2x1x2 = 3
<=> m2 + 2m2 + 2 = 3
<=> 3m2 = 1
=> m2 = \(\dfrac{1}{3}\)
=> m = +- \(\dfrac{1}{\sqrt{3}}\)

Lời giải:
Để pt có 2 nghiệm pb thì $\Delta=25-4(m-2)>0\Leftrightarrow m< \frac{33}{4}$
Áp dụng định lý Viet: $x_1+x_2=5$ và $x_1x_2=m-2$
Khi đó:
$x_1^2+4x_1+x_2=9$
$\Leftrightarrow x_1^2+3x_1+(x_1+x_2)=9$
$\Leftrightarrow x_1^2+3x_1+5=9\Leftrightarrow x_1^2+3x_1-4=0$
$\Leftrightarrow (x_1-1)(x_1+4)=0$
$\Leftrightarrow x_1=1$ hoặc $x_1=-4$
$x_1=1$ thì $x_2=4$
$\Rightarrow m-2=x_1x_2=4\Rightarrow m=6$
$x_1=-4$ thì $x_2=9$
$\Rightarrow m-2=x_1x_2=-36\Rightarrow m=-34$
Vì $m< \frac{33}{4}$ nên cả 2 giá trị này đều thỏa

Do phương trình có 2 nghiệm x1, x2
\(\Rightarrow\left\{{}\begin{matrix}S=x_1+x_2=5m\\P=x_1.x_2=5m-1\end{matrix}\right.\)
Ta có:
\(x_1^2+x_2^2=2\)
\(\left(x_1^2+2x_1x_2+x_2^2\right)-2x_1x_2=2\)
\(\left(x_1+x_2\right)^2-2x_1x_2-2=0\)
\(\left(5m^2\right)-2\left(5m-1\right)-2=0\)
\(25m^2-10m+2-2=0\)
\(25m^2-10m=0\)
\(5m\left(5m-2\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}m=0\\m=\dfrac{2}{5}\end{matrix}\right.\)
Vậy ...

Do pt có 2 nghiệm phân biệt \(x_1,x_2\) nên theo đ/l Vi-ét , ta có :
\(\left\{{}\begin{matrix}S=x_1+x_2=-\dfrac{b}{a}=3m\\P=x_1x_2=\dfrac{c}{a}=3m-1\end{matrix}\right.\)
Ta có :
\(x_1^2+x_2^2=6\)
\(\Leftrightarrow S^2+2P-6=0\)
\(\Leftrightarrow\left(3m\right)^2+2\left(3m-1\right)-6=0\)
\(\Leftrightarrow9m^2+6m-2-6=0\)
\(\Leftrightarrow9m^2+6m-8=0\)
\(\Delta=b^2-4ac=6^2-4.9.\left(-8\right)=324>0\)
\(\Rightarrow\)Pt có 2 nghiệm \(m_1,m_2\)
\(\left\{{}\begin{matrix}m_1=\dfrac{-b+\sqrt{\Delta}}{2a}=\dfrac{-6+18}{18}=\dfrac{2}{3}\\m_2=\dfrac{-b-\sqrt{\Delta}}{2a}=\dfrac{-6-18}{18}=-\dfrac{4}{3}\end{matrix}\right.\)
Vậy \(m=\dfrac{2}{3};m=-\dfrac{4}{3}\) thì thỏa mãn \(x_1^2+x_2^2=6\)

\(\Delta=\left(-3m\right)^2-4\left(3m-1\right)\)
\(=9m^2-12m+4=\left(3m-1\right)^2+3>0\)
=> pt luôn có 2 nghiệm phân biệt
Theo hệ thức Vi-ét, ta có: \(\left\{{}\begin{matrix}x_1+x_2=3m\\x_1.x_2=3m-1\end{matrix}\right.\)
\(x_1^2+x_2^2=6\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1.x_2=6\)
\(\Leftrightarrow\left(3m\right)^2-2\left(3m-1\right)=6\)
\(\Leftrightarrow9m^2-6m+2=6\)
\(\Leftrightarrow9m^2-6m-4=0\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{1-\sqrt{5}}{3}\\x=\dfrac{1+\sqrt{5}}{3}\end{matrix}\right.\)