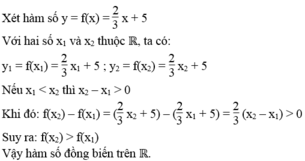Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

`a=m^2+m+1=m^2+2.m. 1/2 + (1/2)^2 + 3/4= (m+1/2)^2 + 3/4 >0 forall m`
`=> a>0 =>` Hàm số luôn đồng biến trên `RR`.
Để hàm số trên đồng biến khi \(m^2+m+1=m^2+m+\dfrac{1}{4}+\dfrac{3}{4}=\left(m+\dfrac{1}{2}\right)^2+\dfrac{3}{4}>0\)
Vậy hàm số luôn đồng biến trên R

\(\dfrac{f\left(x_1\right)-f\left(x_2\right)}{x_1-x_2}=\dfrac{-x_1+1+x_2-1}{x_1-x_2}=-1\)
Vậy: f(x) nghịch biến trên R

Mình chỉ lo bạn không dịch được chữ =)
Cho \(f\left(x\right)=mx-2\) và \(g\left(x\right)=\left(m^2+1\right)x+5\)
\(f\left(x\right)+g\left(x\right)=mx-2+m^2x+x+5\)
\(=mx+m^2x+x+3\)
\(=x\left(m+m^2+1\right)+3\)
Ta có: \(m^2+m+1=\left(m+1\right)^2+\frac{3}{4}>0\forall m\)
\(\Rightarrow f\left(x\right)+g\left(x\right)\) là hàm số bậc nhất đồng biến
\(f\left(x\right)-g\left(x\right)=mx-2-m^2x-x-5\)
\(=x\left(m-m^2-1\right)-5\)
Ta có: \(-m^2+m-1=-m^2+2.\frac{1}{2}m-\frac{1}{4}-\frac{3}{4}=-\left(m-\frac{1}{2}\right)^2-\frac{3}{4}< 0\forall m\)
\(\Rightarrow f\left(x\right)-g\left(x\right)\) là hàm số bậc nhất nghịch biến

Lời giải:
a)
\(f(x)=g(x)\Leftrightarrow 7x=2+5x^2\)
\(\Leftrightarrow 5x^2+2-7x=0\)
\(\Leftrightarrow (5x^2-5x)-(2x-2)=0\)
\(\Leftrightarrow 5x(x-1)-2(x-1)=0\Leftrightarrow (5x-2)(x-1)=0\)
\(\Rightarrow \left[\begin{matrix} x=\frac{2}{5}\\ x=1\end{matrix}\right.\)
b)
Ta có: \(\left\{\begin{matrix} f(-x)=7(-x)=-7x\\ -f(x)=-7x\end{matrix}\right.\Rightarrow f(-x)=-f(x)\)
\(\left\{\begin{matrix} g(-x)=2+5(-x)^2=2+5x^2\\ g(x)=2+5x^2\end{matrix}\right.\Rightarrow g(-x)=g(x)\)
c)
Xét \(x_1< x_2< 0\) đều thuộc TXĐ:
Khi đó:
\(g(x_1)-g(x_2)=2+5x_1^2-(2+5x_2^2)=5x_1^2-5x_2^2=5(x_1-x_2)(x_1+x_2)\)
Vì \(x_1< x_2< 0\Rightarrow x_1-x_2< 0; x_1+x_2< 0\)
Do đó: \(g(x_1)-g(x_2)=5(x_1-x_2)(x_1+x_2)>0\Rightarrow g(x_1)> g(x_2)\)
Vậy hàm số nghịch biến khi $x< 0$
------------
Xét \(x_1> x_2>0\) thuộc TXĐ:
Khi đó:
\(g(x_1)-g(x_2)=(2+5x_1^2)-(2+5x_2^2)=5x_1^2-5x_2^2=5(x_1-x_2)(x_1+x_2)\)
Vì \(x_1> x_2>0\Rightarrow x_1-x_2>0; x_1+x_2>0\)
\(\Rightarrow g(x_1)-g(x_2)>0\Rightarrow g(x_1)> g(x_2)\)
Vậy hàm số đồng biến khi $x>0$

Với x 1 , x 2 là hai giá trị bất kì của x thuộc R, ta có:
y 1 = f( x 1 ) = 4 - 2/5 x 1 ; y 2 = f( x 2 ) = 4 - 2/5 x 2
Nếu x 1 < x 2 thì x 1 - x 2 < 0. Khi đó ta có:
y 1 - y 2 = (4 - 2/5 x 1 ) - (4 - 2/5 x 2 )
= (-2)/5( x 1 - x 2 ) > 0. Suy ra y 1 > y 2
Vậy hàm số đã cho là hàm nghịch biến trên R.

\(\dfrac{f\left(x_1\right)-f\left(x_2\right)}{x_1-x_2}=\dfrac{3x_1-2-3x_2+2}{x_1-x_2}=3\)
Vậy: Hàm số đồng biến trên R

Gia su \(x_1< x_2\)
\(\Rightarrow x_1-x_2< 0\left(1\right)\)
Ta co:
\(f\left(x_1\right)-f\left(x_2\right)=\left(3m^2-7m+5\right)x_1-2011-\left(3m^2-7m+5\right)x_2+2011=\left(x_1-x_2\right)\left(3m^2-7m+5\right)\)Vi la chung minh dong bien nen xet
\(3m^2-7m+5>0\)
Dat \(g\left(m\right)=3m^2-7m+5\)
Ta lai co:
\(\Delta=\left(-7\right)^2-4.3.5=-11< 0\)
Theo dinh li dau tam thuc bac hai thi \(g\left(m\right)\)cung dau voi he so 3
\(\Rightarrow3m^2-7m+5>0\left(2\right)\left(\forall m\right)\)
Tu \(\left(1\right)\)va \(\left(2\right)\)suy ra;
\(\left(x_1-x_2\right)\left(3m^2-7m+5\right)< 0\)
Ma \(f\left(x_1\right)-f\left(x_2\right)=\left(x_1-x_2\right)\left(3m^2-7m+5\right)\)
\(\Rightarrow f\left(x_1\right)< f\left(x_2\right)\)
Vay ham so \(y=f\left(x\right)=\left(3m^2-7m+5\right)x-2011\)dong bien voi moi m

Do x 1 < x 2 nên x 1 − x 2 < 0
Ta có:
f x 1 − f x 2 = 3 x 1 + 1 − 3 x 2 + 1 = 3 x 1 − x 2 < 0 ⇔ f x 1 < f x 2
Vậy hàm số y = 3x + 1 đồng biến trên R