Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Đề bài trên sai. Đề đúng: CM: \(\dfrac{1}{2}.\dfrac{3}{4}.\dfrac{5}{6}...\dfrac{97}{98}.\dfrac{99}{100}>\dfrac{\sqrt{2}}{20}\).

a) \(2x-\dfrac{x-3}{5}-4x+1\le0\)
\(\Leftrightarrow10x-x+3-20x+5\le0\)
\(\Leftrightarrow-11x+8\le0\)
\(\Leftrightarrow x\ge\dfrac{8}{11}\)
\(\Rightarrow x\in\left(\dfrac{8}{11};+\infty\right)\)
b) \(\sqrt{x^2+2}\le x-1\)
\(\Leftrightarrow x^2+2\le x^2-2x+1\) \(\left(x-1\ge\sqrt{x^2+2}\ge\sqrt{2}\Rightarrow x\ge1+\sqrt{2}\right)\)
\(\Leftrightarrow x\le-\dfrac{1}{2}\)
\(\Rightarrow x\in\varnothing\)
c) \(\sqrt{x-1}+\sqrt{5-x}+\dfrac{1}{x-3}>\dfrac{1}{x-3}\) (\(x\in\left[1;5\right]\backslash\left\{3\right\}\))
\(\Leftrightarrow\sqrt{x-1}+\sqrt{5-x}>0\)
\(\Leftrightarrow4+2\sqrt{\left(x-1\right)\left(5-x\right)}>0\) ( luôn đúng )
vậy \(x\in\left[1;5\right]\backslash\left\{3\right\}\)

ĐK \(x\ne-2;-3;-5;-6\)
\(\Leftrightarrow\dfrac{x-1}{x+2}-1-\left(\dfrac{x-2}{x+3}-1\right)=\dfrac{x-4}{x+5}-1-\left(\dfrac{x-5}{x+6}-1\right)\)
\(\Leftrightarrow\dfrac{x-1-x-2}{x+2}-\dfrac{x-2-x-3}{x+3}=\dfrac{x-4-x-5}{x+5}-\dfrac{x-5-x-6}{x+6}\)
\(\Leftrightarrow\dfrac{-3}{x+2}-\dfrac{-5}{x+3}=\dfrac{-9}{x+5}-\dfrac{-11}{x+6}\)
\(\Leftrightarrow\dfrac{3}{x+2}-\dfrac{5}{x+3}=\dfrac{9}{x+5}-\dfrac{11}{x+6}\)
\(\Leftrightarrow\dfrac{3}{x+2}+\dfrac{11}{x+6}=\dfrac{9}{x+5}+\dfrac{5}{x+3}\)
\(\Leftrightarrow\dfrac{3\left(x+6\right)+11\left(x+2\right)}{\left(x+2\right)\left(x+6\right)}=\dfrac{9\left(x+3\right)+5\left(x+5\right)}{\left(x+3\right)\left(x+5\right)}\)
\(\Leftrightarrow\dfrac{14x+40}{\left(x+2\right)\left(x+6\right)}=\dfrac{14x+52}{\left(x+3\right)\left(x+5\right)}\)
\(\Leftrightarrow\left(x+2\right)\left(x+6\right)\left(14x+52\right)=\left(x+3\right)\left(x+5\right)\left(14x+40\right)\)
\(\Leftrightarrow\left(x^2+8x+12\right)\left(14x+52\right)=\left(x^2+8x+15\right)\left(14x+40\right)\)
\(\Leftrightarrow14x\left(x^2+8x+12\right)+52\left(x^2+8x+12\right)=14x\left(x^2+8x+15\right)+40\left(x^2+8x+15\right)\)
\(\Leftrightarrow14x\left(x^2+8x\right)+12.14x+52\left(x^2+8x\right)+52.12=14x\left(x^2+8x\right)+15.14x+40\left(x^2+8x\right)+15.40\)
\(\Leftrightarrow12\left(x^2+8x\right)-42x+24=0\)
\(\Leftrightarrow12x^2+54x+24=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-\dfrac{1}{2}\\x=-4\end{matrix}\right.\)

\(P=sin^22x-\left[2sin\dfrac{x}{2}cos\dfrac{x}{2}\left(cos^4\dfrac{x}{2}-sin^4\dfrac{x}{2}\right)\right]^2\)
\(=sin^22x-\left[sinx\left(cos^2\dfrac{x}{2}-sin^2\dfrac{x}{2}\right)\left(cos^2\dfrac{x}{2}+sin^2\dfrac{x}{2}\right)\right]^2\)
\(=sin^22x-\left[sinx.cosx.1\right]^2\)
\(=sin^22x-\left[\dfrac{1}{2}sin2x\right]^2\)
\(=\dfrac{3}{4}sin^22x=\dfrac{3}{4}\left(1-cos^22x\right)=\dfrac{3}{4}\left(1-\dfrac{1}{4}\right)=\dfrac{9}{16}\)

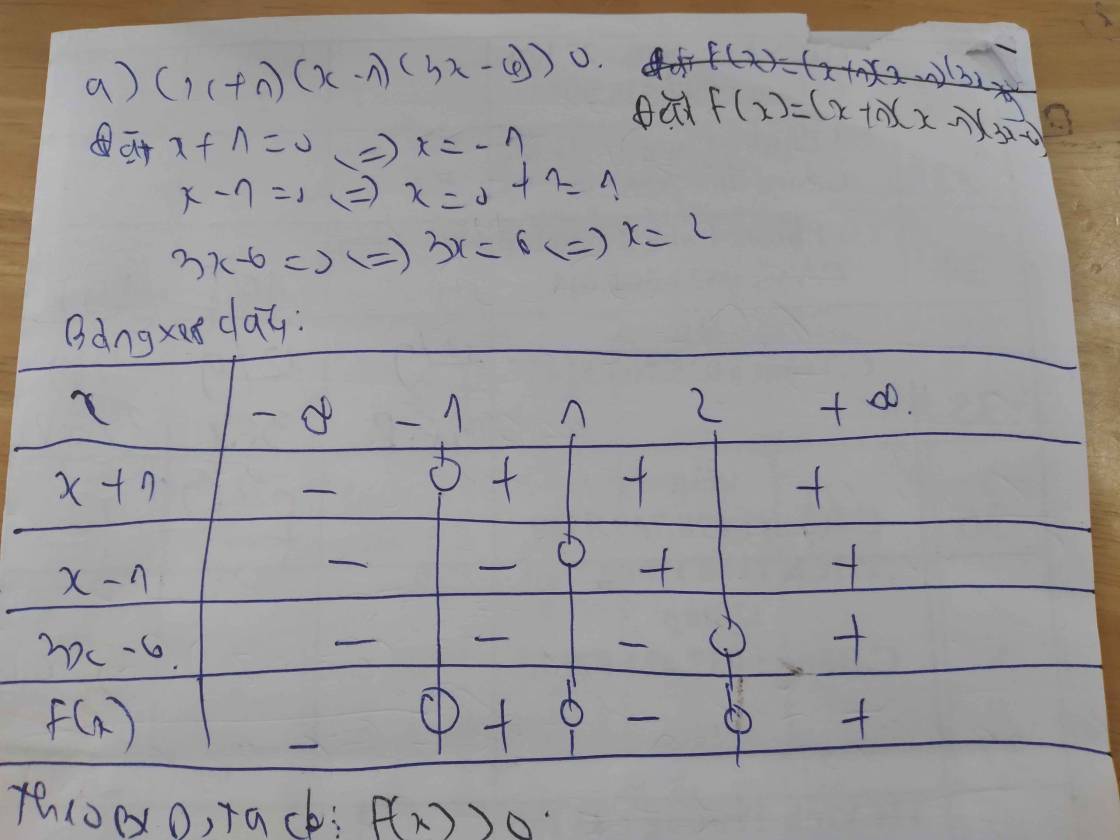
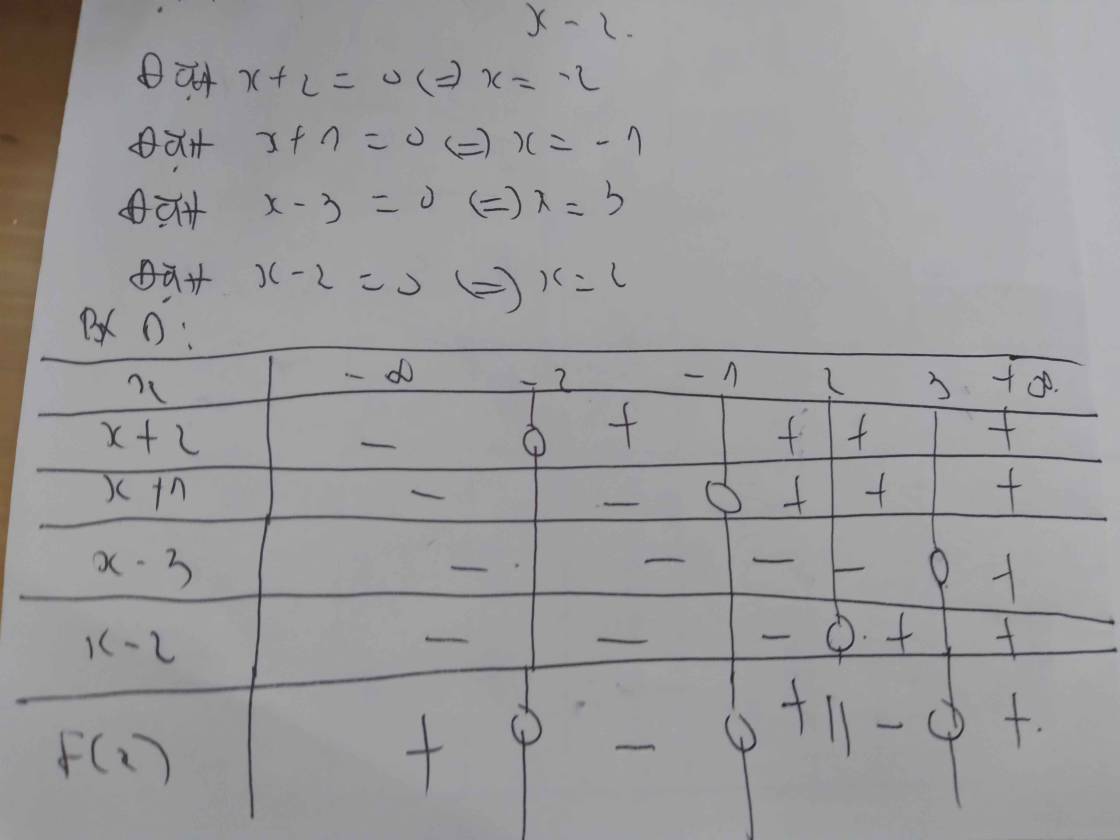
a) \(\left(x+1\right)\left(x-1\right)\left(3x-6\right)>0\)
Lập bảng xét dấu ta được kết quả :
\(Bpt\Leftrightarrow\left[{}\begin{matrix}-1< x< 1\\x>2\end{matrix}\right.\)
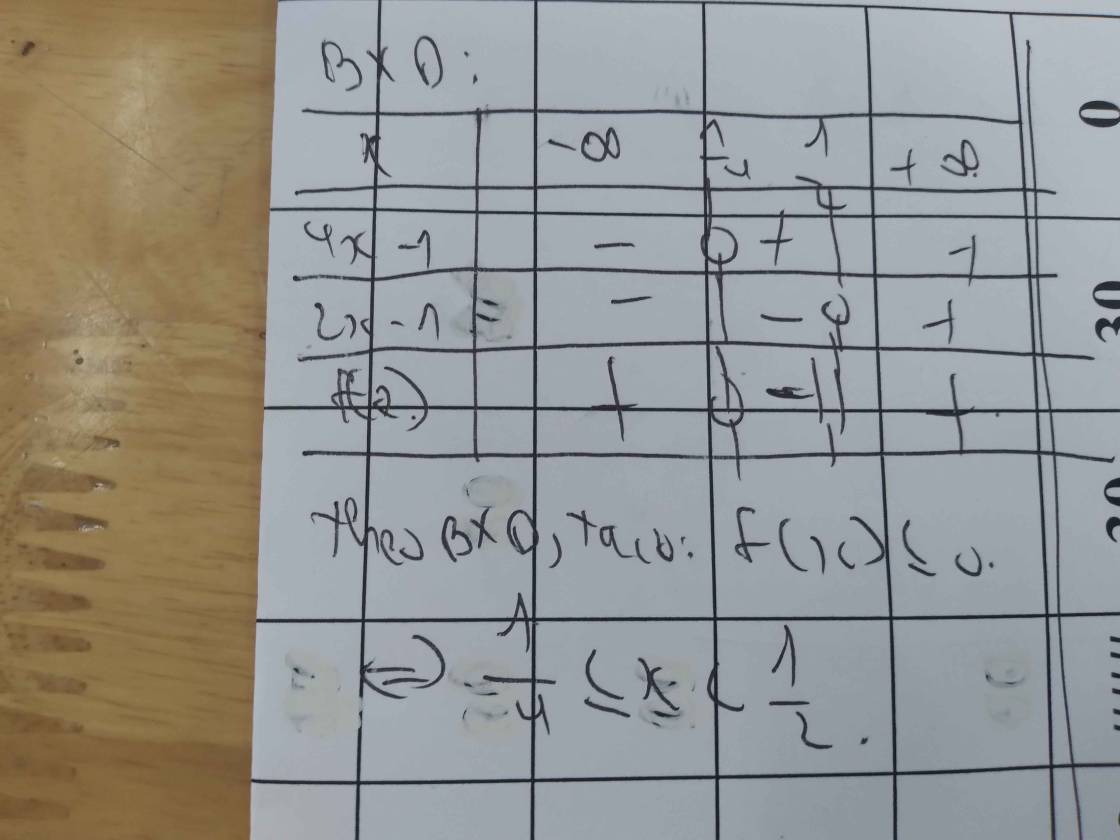
b) \(\dfrac{x+3}{x-2}\le0\)
Lập bảng xét dấu ta được kết quả :
\(Bpt\Leftrightarrow-3\le x< 2\)
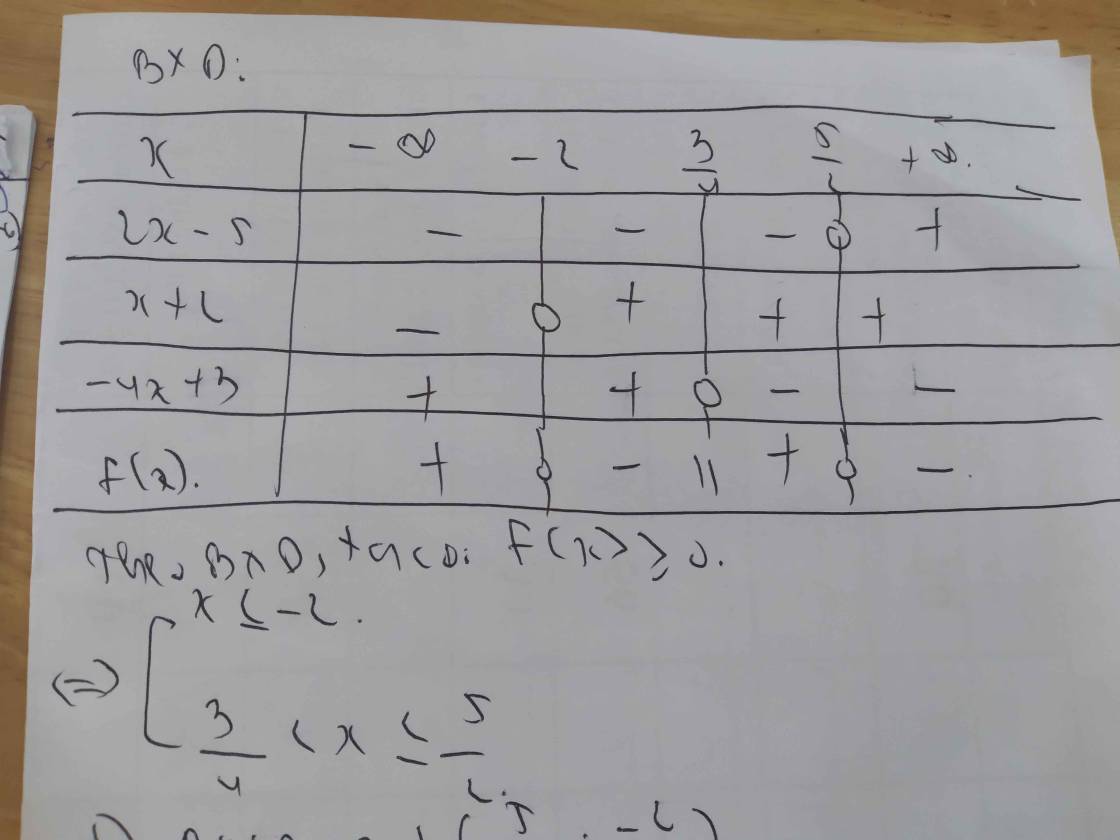
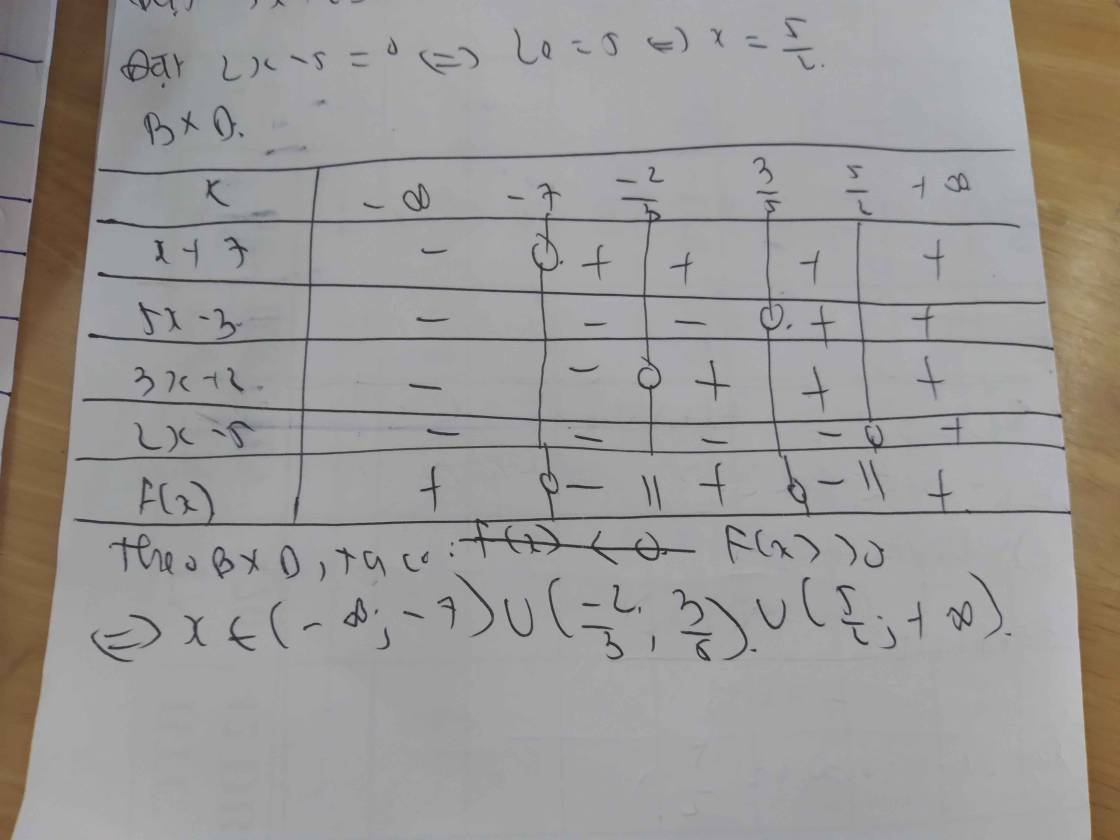
d) \(\dfrac{2x-5}{3x+2}< \dfrac{3x+2}{2x-5}\)
\(\Leftrightarrow\dfrac{2x-5}{3x+2}-\dfrac{3x+2}{2x-5}< 0\)
\(\Leftrightarrow\dfrac{\left(2x-5\right)^2-\left(3x+2\right)^2}{\left(3x+2\right)\left(2x-5\right)}< 0\)
\(\Leftrightarrow\dfrac{\left(2x-5+3x+2\right)\left(2x-5-3x-2\right)}{\left(3x+2\right)\left(2x-5\right)}< 0\)
\(\Leftrightarrow\dfrac{-\left(5x-3\right)\left(x+7\right)}{\left(3x+2\right)\left(2x-5\right)}< 0\)
Lập bảng xét dấu ta được kết quả :
\(Bpt\Leftrightarrow\left[{}\begin{matrix}-7< x< -\dfrac{2}{3}\\\dfrac{5}{3}< x< \dfrac{5}{2}\end{matrix}\right.\)

- Thay từng giá trị vào, ta thấy A. \(\dfrac{15}{4}\) thỏa mãn.

Biểu thức này không tồn tại cả min lẫn max
Nó chỉ tồn tại min khi có thêm điều kiện \(x>2\)

a) \(\dfrac{5+x}{4-x}=\dfrac{1}{2}\)
\(\Leftrightarrow2\left(5+x\right)=4-x\)
\(\Leftrightarrow2\left(5+x\right)-\left(4-x\right)=0\)
\(\Leftrightarrow10+2x-4+x=0\)
\(\Leftrightarrow6+3x=0\)
\(\Leftrightarrow3x=-6\)
\(\Leftrightarrow x=-2\)
Vậy x=-2
b) \(\dfrac{25}{14}=\dfrac{x+7}{x-4}\)
\(\Leftrightarrow25\left(x-4\right)=14\left(x+7\right)\)
\(\Leftrightarrow25\left(x-4\right)-14\left(x+7\right)=0\)
\(\Leftrightarrow25x-100-14x-98=0\)
\(\Leftrightarrow11x-198=0\)
\(\Leftrightarrow11x=198\)
\(\Leftrightarrow x=18\)
Vậy x=18
c) \(\dfrac{3x-5}{x+4}=\dfrac{5}{2}\)
\(\Leftrightarrow2\left(3x-5\right)=5\left(x+4\right)\)
\(\Leftrightarrow2\left(3x-5\right)-5\left(x+4\right)=0\)
\(\Leftrightarrow6x-10-5x-20=0\)
\(\Leftrightarrow x-30=0\)
\(\Leftrightarrow x=30\)
Vậy x=30
d) \(\dfrac{3x-1}{2x+1}=\dfrac{3}{7}\)
\(\Leftrightarrow7\left(3x-1\right)=3\left(2x+1\right)\)
\(\Leftrightarrow7\left(3x-1\right)-3\left(2x+1\right)=0\)
\(\Leftrightarrow21x-7-6x-3=0\)
\(\Leftrightarrow15x-10=0\)
\(\Leftrightarrow15x=10\)
\(\Leftrightarrow x=\dfrac{10}{15}=\dfrac{2}{3}\)
Vậy \(x=\dfrac{2}{3}\)



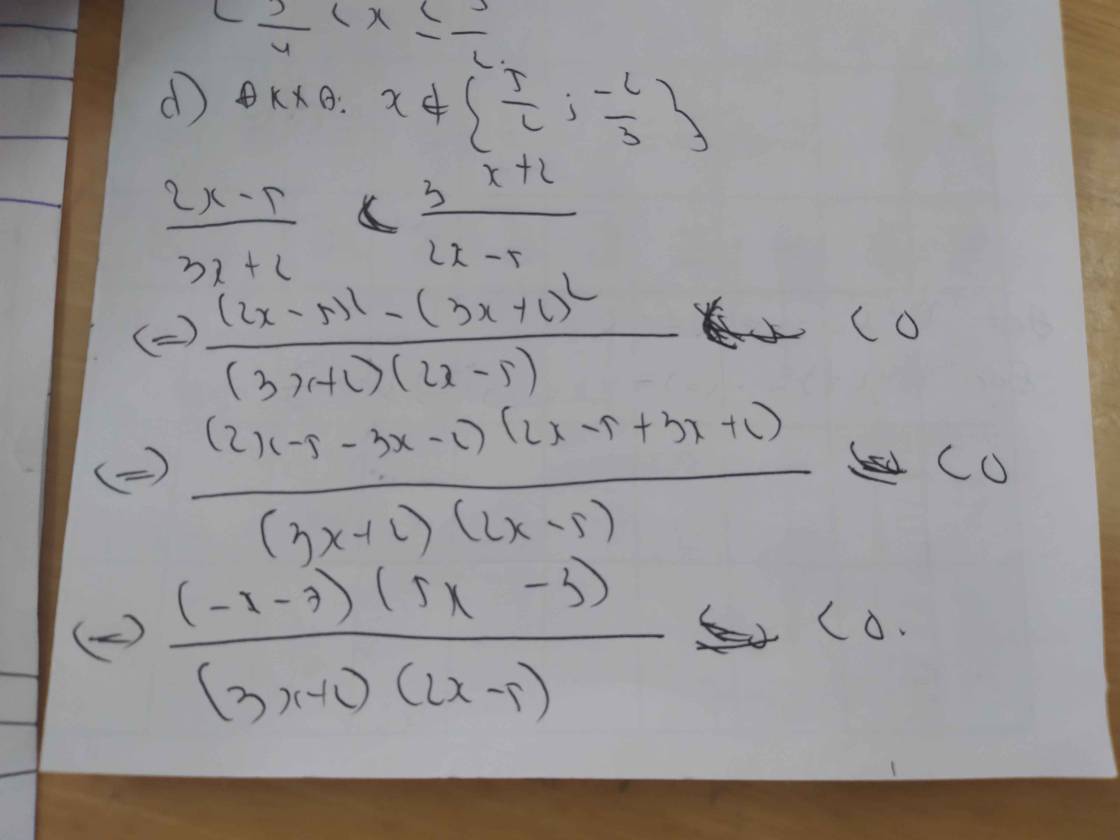

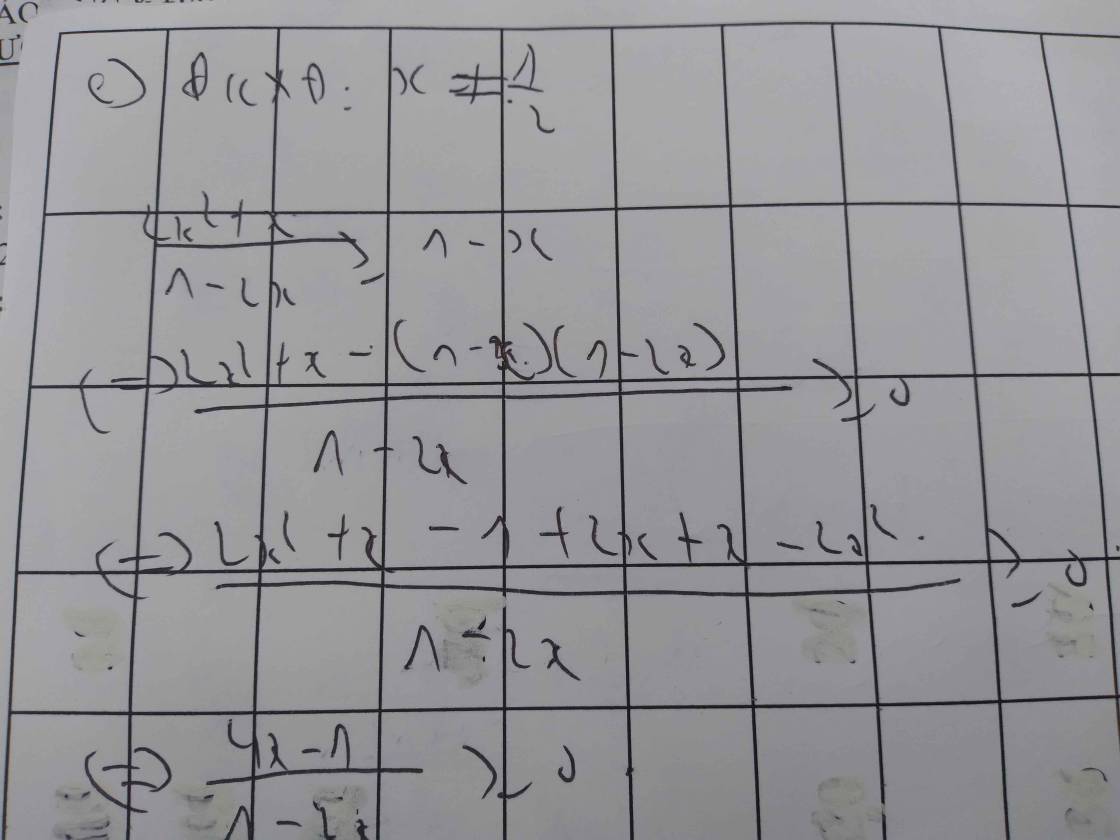

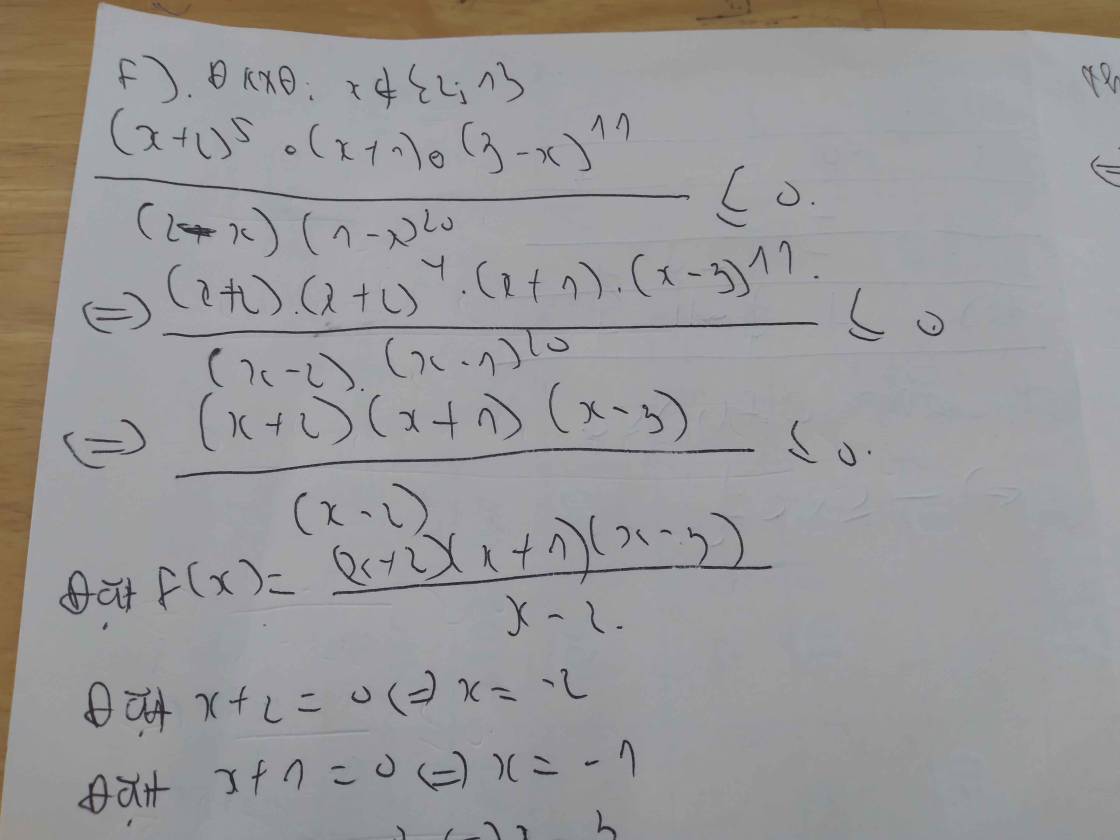

Ta có: \(\dfrac{x+5}{100}+\dfrac{x+5}{99}=\dfrac{x+5}{98}+\dfrac{x+5}{97}\)
=> \(\dfrac{x+5}{100}+\dfrac{x+5}{99}-\dfrac{x+5}{98}-\dfrac{x+5}{97}=0\)
=> \(\left(x+5\right).\left(\dfrac{1}{100}+\dfrac{1}{99}-\dfrac{1}{98}-\dfrac{1}{97}\right)=0\)
=> \(x+5=0\)
=> \(x=-5\)
Vậy x= -5