Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: \(A=\left(2x-1\right)\left(4x^2+2x+1\right)-7\left(x^3+1\right)\)
\(=\left(2x\right)^3-1^3-7x^3-7\)
\(=8x^3-1-7x^3-7=x^3-8\)
b: Thay x=-1/2 vào A, ta được:
\(A=\left(-\dfrac{1}{2}\right)^3-8=-\dfrac{1}{8}-8=-\dfrac{65}{8}\)

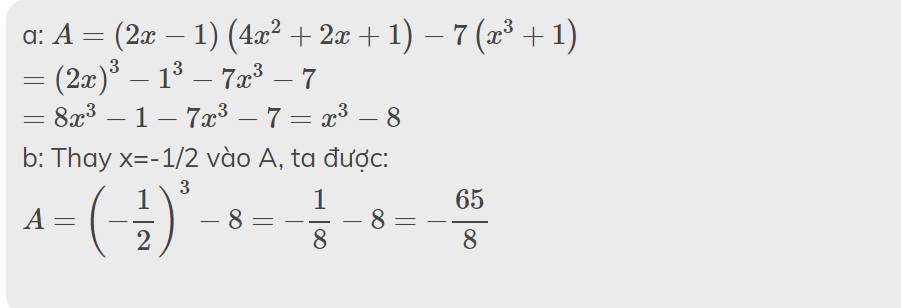
c: \(A=x^3-8=\left(x-2\right)\left(x^2+2x+4\right)\)
Để A là số nguyên tố thì x-2=1
=>x=3

a) Ta có: \(A=\left(1+\dfrac{x^2}{x^2+1}\right):\left(\dfrac{1}{x-1}-\dfrac{2x}{x^3+x-x^2-1}\right)\)
\(=\dfrac{2x^2+1}{x^2+1}:\dfrac{x^2+1-2x}{\left(x-1\right)\left(x^2+1\right)}\)
\(=\dfrac{2x^2+1}{x^2+1}\cdot\dfrac{\left(x-1\right)\left(x^2+1\right)}{\left(x-1\right)^2}\)
\(=\dfrac{2x^2+1}{x-1}\)
b) Thay \(x=-\dfrac{1}{2}\) vào A, ta được:
\(A=\left(2\cdot\dfrac{1}{4}+1\right):\left(\dfrac{-1}{2}-1\right)\)
\(=\dfrac{3}{2}:\dfrac{-3}{2}=-1\)
c) Để A<1 thì A-1<0
\(\Leftrightarrow\dfrac{2x^2+1}{x-1}-1< 0\)
\(\Leftrightarrow\dfrac{2x^2+1-x+1}{x-1}< 0\)
\(\Leftrightarrow\dfrac{2x^2-x+2}{x-1}< 0\)
\(\Leftrightarrow x-1< 0\)
hay x<1

\(a,\)Với \(x\ne-3,x\ne2\) ta có :
\(A=\dfrac{x+2}{x+3}-\dfrac{5}{x^2+x-6}-\dfrac{1}{x-2}\)
\(=\dfrac{x^2-4}{\left(x+3\right)\left(x-2\right)}-\dfrac{5}{\left(x+3\right)\left(x-2\right)}-\dfrac{x+3}{\left(x+3\right)\left(x-2\right)}\)
\(=\dfrac{x^2-4-5-x-3}{\left(x+3\right)\left(x-2\right)}\)
\(=\dfrac{x^2-x-12}{\left(x+3\right)\left(x-2\right)}\)
\(=\dfrac{\left(x-4\right)\left(x+3\right)}{\left(x+3\right)\left(x-2\right)}\)
\(=\dfrac{x-4}{x-2}\)
\(b,\) \(A=-3\Leftrightarrow\dfrac{x-4}{x-2}=-3\)
\(\Leftrightarrow x-4=-3\left(x-2\right)\)
\(\Leftrightarrow x-4+3x-6=0\)
\(\Leftrightarrow4x=10\Rightarrow x=\dfrac{10}{4}=\dfrac{5}{2}\)

a: \(A=\left(\dfrac{x}{x^2-4}+\dfrac{4}{x-2}+\dfrac{1}{x+2}\right):\dfrac{3x+3}{x^2+2x}\)
\(=\dfrac{x+4x+8+x-2}{\left(x-2\right)\left(x+2\right)}\cdot\dfrac{x\left(x+2\right)}{3\left(x+1\right)}\)
\(=\dfrac{6\left(x+1\right)\cdot x\left(x+2\right)}{3\left(x+1\right)\left(x-2\right)\left(x+2\right)}\)
\(=\dfrac{2x}{x-2}\)

a: \(A=4x-3x^2+20-15x-9x^2-12x-4+\left(2x+1\right)^3-\left(8x^3-1\right)\)
\(=-12x^2-23x+16+8x^3+12x^2+6x+1-8x^3+1\)
\(=-17x+18\)
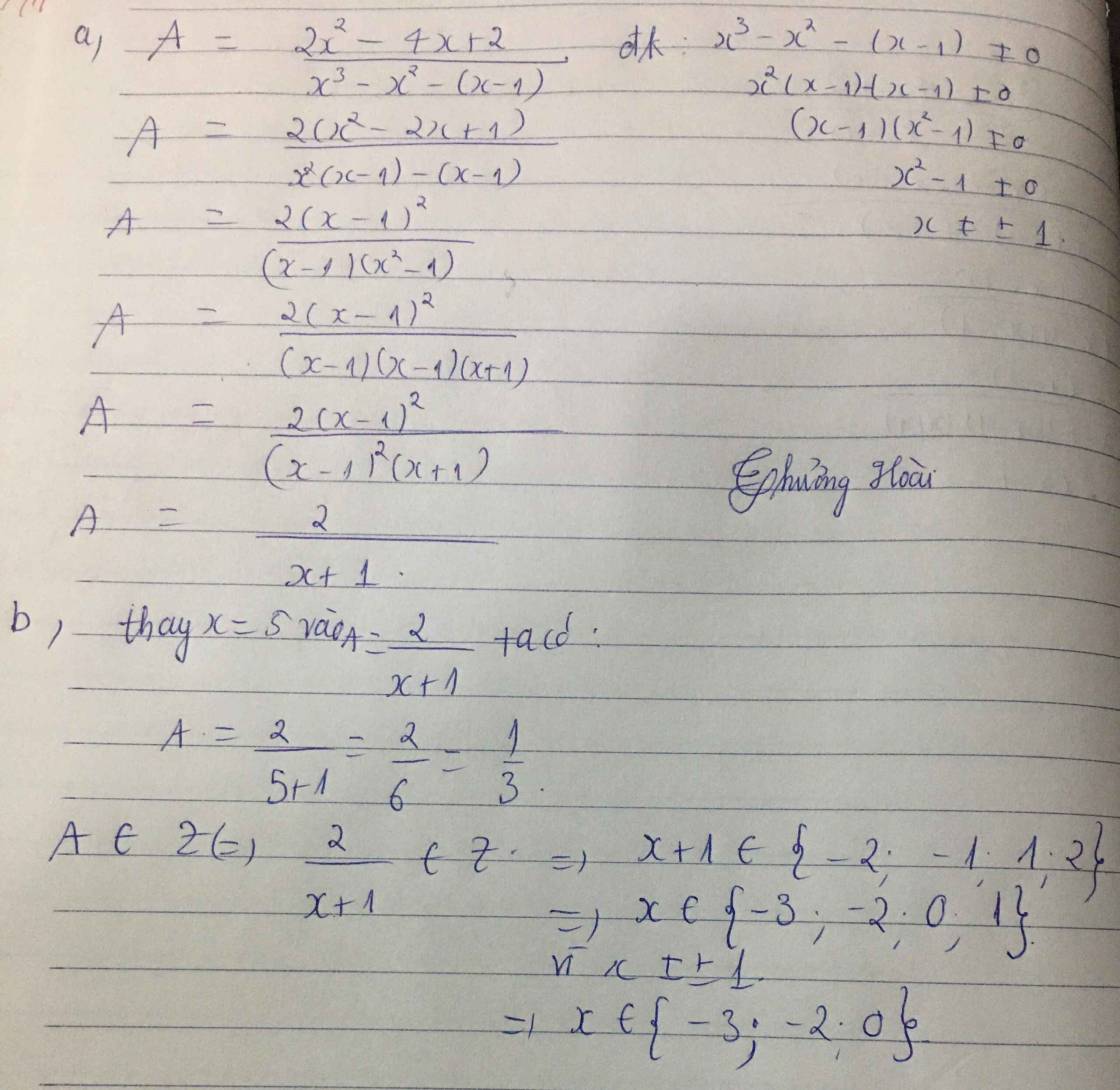
a ) \(\text{A}=\left(\frac{3}{x+1}+\frac{1}{1-x}-\frac{8}{1-x^2}\right):\frac{1-2x}{x^2-1}\)
\(=\left(\frac{3.\left(1-x\right)+1.\left(1+x\right)}{\left(1+x\right).\left(1-x\right)}-\frac{8}{1-x^2}\right).\frac{x^2-1}{1-2x}\)
\(=\frac{3-3x+1+x-8}{1-x^2}.\frac{x^2-1}{1-2x}\)
\(=\frac{-2x-4}{1-x^2}.\frac{x^2-1}{1-2x}\)
\(=\frac{-2x^3+2x-4x^2+4}{1-2x-x^2+2x^3}\)
\(=\frac{-2x^3-4x^2+2x+4}{2x^3-x^2-2x+1}\) ( * )
b ) Ta có : | 3x + 5 | = 2
\(\Leftrightarrow\orbr{\begin{cases}3x+5=2\\3x+5=-2\end{cases}}\Leftrightarrow\orbr{\begin{cases}3x=-3\\3x=-7\end{cases}}\Leftrightarrow\orbr{\begin{cases}x=-1\\x=-\frac{7}{3}\end{cases}}\)
Ta có : \(A=\frac{-2x^3-4x^2+2x+4}{2x^3-x^2-2x+1}\)
Đkxđ : \(2x^3-x^2-2x+1\ne0\) ( vì mẫu phải khác 0 )
Thay x = -1 vào ( * ) ta được : \(\frac{-2.\left(-1\right)^3-4.\left(-1\right)^2+2.\left(-1\right)+4}{2.\left(-1\right)^3-\left(-1\right)^2-2.\left(-1\right)+1}=\frac{0}{0}\left(lo\text{ại}\right)\)
Thay x = -7/3 vào ( * ) ta được : \(\frac{-2.\left(-\frac{7}{3}\right)^3-4.\left(-\frac{7}{3}\right)^2+2.\left(-\frac{7}{3}\right)+4}{2.\left(-\frac{7}{3}\right)^3-\left(-\frac{7}{3}\right)^2-2.\left(-\frac{7}{3}\right)+1}=-\frac{2}{17}\left(nh\text{ận}\right)\)
A có giá trị dương <=> A \(\ge\) 0
\(\Leftrightarrow\frac{-2x^3-4x^2+2x+4}{2x^3-x^2-2x+1}\ge0\)
\(\Leftrightarrow-2x^3-4x^2+2x+4\le0\)
\(\Leftrightarrow\hept{\begin{cases}x\ge-2\\x< -1\end{cases}}\) ( cái này là bất phương trình , dùng máy tính bấm ra nha bạn )
sai rồi, theo mk câu a bạn chưa rút gọn hết, cái gt x=-1 k cần thay vì theo ĐKXĐ, x khác -1 mà