K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
S
1
15 tháng 6 2016
\(\frac{1}{51}+\frac{1}{52}+...+\frac{1}{100}< \frac{1}{100}+\frac{1}{100}+...+\frac{1}{100}=50.\frac{1}{100}=\frac{1}{2}< \frac{5}{6}\)
Vậy \(\frac{1}{51}+\frac{1}{52}+...+\frac{1}{100}< \frac{5}{6}\)
KH
2
NV
Nguyễn Việt Lâm
Giáo viên
3 tháng 3 2021
\(\Leftrightarrow8x^3-36x^2+51x-22+2x-3-\sqrt[3]{3x-5}=0\)
\(\Leftrightarrow8x^3-36x^2+51x-22+\dfrac{8x^3-36x^2+51x-22}{\left(2x-3\right)^2+\left(2x-3\right)\sqrt[3]{3x-5}+\sqrt[3]{\left(3x-5\right)^2}}=0\)
\(\Leftrightarrow\left(8x^3-36x^2+51x-22\right)\left(1+\dfrac{1}{\left(2x-3\right)^2+\left(2x-3\right)\sqrt[3]{3x-5}+\sqrt[3]{\left(3x-5\right)^2}}\right)=0\)
\(\Leftrightarrow8x^3-36x^2+51x-22=0\)
\(\Leftrightarrow\left(x-2\right)\left(8x^2-20x+11\right)=0\)
\(\Leftrightarrow...\)
VT
26 tháng 7 2017
a, 16.(27+15)+8.(53+25):2
= 4.[(27+15).4] + 4.(53+25)
= 4.168 + 4.312
= 4.(168+312)
= 4.480=1920.
...
DH
26 tháng 7 2017
Câu b tình bt thui!
b, 53.(51+4)+53.53.(49+96)+53
=53.55+53.53.145+53
=2915+407305+53
=410220+53=410273
NC
2
DH
24 tháng 6 2017
\(\dfrac{-1}{39}+\dfrac{-1}{52}=\dfrac{-7}{156}\)
\(\dfrac{-6}{9}+\dfrac{-12}{16}=\dfrac{-17}{12}\)
\(\dfrac{-2}{5}-\dfrac{-3}{11}=\dfrac{-7}{55}\)
\(\dfrac{-34}{37}.\dfrac{74}{-85}=\dfrac{4}{5}\)
\(\dfrac{-5}{9}:\dfrac{-7}{18}=\dfrac{10}{7}\)
Chúc bạn học tốt!!!
Q
24 tháng 6 2017
a) \(\left(-\dfrac{1}{39}\right)+\left(-\dfrac{1}{52}\right)=\dfrac{-4-3}{156}=-\dfrac{7}{156}\)
b) \(\left(-\dfrac{6}{9}\right)+\left(-\dfrac{12}{16}\right)=-\dfrac{6}{9}-\dfrac{12}{16}=-\dfrac{17}{12}\)
c) \(-\dfrac{2}{5}-\left(-\dfrac{3}{11}\right)=-\dfrac{2}{5}+\dfrac{3}{11}=-\dfrac{7}{55}\)
d) \(\left(-\dfrac{34}{37}\right)\cdot\left(-\dfrac{74}{85}\right)=2\cdot\dfrac{2}{5}=\dfrac{4}{5}\)
e) \(\left(-\dfrac{5}{9}\right):\left(-\dfrac{7}{18}\right)=\dfrac{5}{9}\cdot\dfrac{18}{7}=5\cdot\dfrac{2}{7}=\dfrac{10}{7}\)
19 tháng 5 2022
a: \(=\dfrac{54-34}{189-119}=\dfrac{20}{70}=\dfrac{2}{7}\)
b: \(=\dfrac{6+6\cdot4+6\cdot49}{15+15\cdot4+15\cdot49}=\dfrac{6}{15}=\dfrac{2}{5}\)
c: \(=\dfrac{13\left(3-18\right)}{40\left(15-2\right)}=\dfrac{-15}{40}=-\dfrac{3}{8}\)
PT
1
CM
31 tháng 1 2017
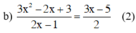
Điều kiện xác định: 2x – 1 ≠ 0 ⇔ x ≠ 1/2.
Quy đồng và bỏ mẫu chung ta được:
Phương trình (2) ⇔ 2(3x2 – 2x + 3) = (2x – 1)(3x – 5)
⇔ 6x2 – 4x + 6 = 6x2 – 10x – 3x + 5
⇔ 9x = –1
⇔ x = –1/9 (thỏa mãn đkxđ)
Vậy phương trình có nghiệm là x = –1/9.
Y
1
B=1+5+52+53+...+52008+52009 (1)
5B=5+52+53+54+...+52009+52010 (2)
Lấy (2) - (1) theo từng vế
5B - B = (5+52+53+54+....+52009+52010) - (1+5+52+53+...+52008+52009 )
<=> 5+52+53+54+...+52009+52010- 1-5-52-53-54-...-52008-52009
<=> (5-5)+(52-52)+(53-53)+...+(52009-52009)+(52010-1)
<=> 0+0+0+0+...+0)+0+52010-1
4B=52010-1
<=>B=(52010-1) : 4