DP
0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
18 tháng 9 2023
a) \(A=\sqrt[]{x^2-2x+5}\)
\(\Leftrightarrow A=\sqrt[]{x^2-2x+1+4}\)
\(\Leftrightarrow A=\sqrt[]{\left(x+1\right)^2+4}\)
mà \(\left(x+1\right)^2\ge0,\forall x\in R\)
\(A=\sqrt[]{\left(x+1\right)^2+4}\ge\sqrt[]{4}=2\)
Dấu "=" xảy ra khi và chỉ khi \(x+1=0\Leftrightarrow x=-1\)
Vậy \(GTNN\left(A\right)=2\left(khi.x=-1\right)\)
b) \(B=5-\sqrt[]{x^2-6x+14}\)
\(\Leftrightarrow B=5-\sqrt[]{x^2-6x+9+5}\)
\(\Leftrightarrow B=5-\sqrt[]{\left(x-3\right)^2+5}\left(1\right)\)
Ta có : \(\left(x-3\right)^2\ge0,\forall x\in R\)
\(\Leftrightarrow\left(x-3\right)^2+5\ge5,\forall x\in R\)
\(\Leftrightarrow\sqrt[]{\left(x-3\right)^2+5}\ge\sqrt[]{5},\forall x\in R\)
\(\Leftrightarrow-\sqrt[]{\left(x-3\right)^2+5}\le-\sqrt[]{5},\forall x\in R\)
\(\Leftrightarrow B=5-\sqrt[]{\left(x-3\right)^2+5}\le5-\sqrt[]{5},\forall x\in R\)
Dấu "=" xả ra khi và chỉ khi \(x-3=0\Leftrightarrow x=3\)
Vậy \(GTLN\left(B\right)=5-\sqrt[]{5}\left(khi.x=3\right)\)
27 tháng 4 2023
Với \(ab+bc+ca=1\) và a,b,c>0 ta có:
\(\left\{{}\begin{matrix}\sqrt{a^2+1}=\sqrt{\left(a+b\right)\left(c+a\right)}\\\sqrt{b^2+1}=\sqrt{\left(b+c\right)\left(a+b\right)}\\\sqrt{c^2+1}=\sqrt{\left(c+a\right)\left(b+c\right)}\end{matrix}\right.\). Do đó:
\(\dfrac{\sqrt{a^2+1}.\sqrt{b^2+1}}{\sqrt{c^2+1}}=a+b\)
Tương tự: \(\dfrac{\sqrt{b^2+1}.\sqrt{c^2+1}}{\sqrt{a^2+1}}=b+c\) ; \(\dfrac{\sqrt{c^2+1}.\sqrt{a^2+1}}{\sqrt{b^2+1}}=c+a\)
\(\Rightarrow P=2\left(a+b+c\right)\)
\(\Rightarrow P^2=4\left(a+b+c\right)^2\ge4.3\left(ab+bc+ca\right)=4.3.1=12\)
\(\Rightarrow P\ge2\sqrt{3}\)
Dấu "=" xảy ra khi \(a=b=c=\dfrac{\sqrt{3}}{3}\)
Vậy \(MinP=2\sqrt{3}\)
D
1
31 tháng 3 2022
$\large A=\frac{2\sqrt{x}-1}{\sqrt{x}+1}=2-\frac{3}{\sqrt{x}+1}$
Ta có: $\large \sqrt{x}+1\ge1\Leftrightarrow -\frac{3}{\sqrt{x}+1}\ge-3$
Do đó: $\large A \ge 2-3=-1$
Vậy $A_{min}=-1$
Dấu $"="$ xảy ra khi $x=0$
14 tháng 10 2021
\(a,ĐK:\left\{{}\begin{matrix}x\ge5\\x\le3\end{matrix}\right.\Leftrightarrow x\in\varnothing\)
Vậy pt vô nghiệm
\(b,ĐK:x\le\dfrac{2}{5}\\ PT\Leftrightarrow4-5x=2-5x\\ \Leftrightarrow0x=2\Leftrightarrow x\in\varnothing\)
\(c,ĐK:x\ge-\dfrac{3}{2}\\ PT\Leftrightarrow x^2+4x+5-2\sqrt{2x+3}=0\\ \Leftrightarrow\left(2x+3-2\sqrt{2x+3}+1\right)+\left(x^2+2x+1\right)=0\\ \Leftrightarrow\left(\sqrt{2x+3}-1\right)^2+\left(x+1\right)^2=0\\ \Leftrightarrow\left\{{}\begin{matrix}2x+3=1\\x+1=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-1\\x=-1\end{matrix}\right.\Leftrightarrow x=-1\left(tm\right)\\ d,PT\Leftrightarrow\left|x-1\right|=\left|2x-1\right|\Leftrightarrow\left[{}\begin{matrix}x-1=2x-1\\x-1=1-2x\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=\dfrac{2}{3}\end{matrix}\right.\)
TD
1
NV
Nguyễn Việt Lâm
Giáo viên
28 tháng 1 2022
\(\left\{{}\begin{matrix}a;b;c\ge0\\a+b+c=1\end{matrix}\right.\) \(\Rightarrow0\le a;b;c\le1\)
\(\Rightarrow a\left(a-1\right)\le0\Rightarrow a^2\le a\)
\(\Rightarrow\sqrt{2a^2+3a+4}=\sqrt{a^2+a^2+3a+4}\le\sqrt{a^2+a+3a+4}=a+2\)
Tương tự và cộng lại:
\(\Rightarrow M\le a+2+b+2+c+2=7\)
\(M_{max}=7\) khi \(\left(a;b;c\right)=\left(0;0;1\right)\) và các hoán vị
26 tháng 7 2023
1:
a: \(A=\dfrac{\sqrt{x}+1-2}{\sqrt{x}+1}=1-\dfrac{2}{\sqrt{x}+1}\)
căn x+1>=1
=>2/căn x+1<=2
=>-2/căn x+1>=-2
=>A>=-2+1=-1
Dấu = xảy ra khi x=0
b: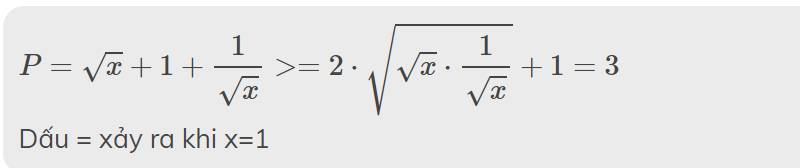 
DP
1
30 tháng 6 2017
\(A=x^2-5\ge-5,\forall x\)Vậy minA = -5 khi x = 0
\(B=\sqrt{x^2+4}\ge\sqrt{4}=2,\forall x\)Vậy minB = 2 khi x = 0
\(C=\sqrt{2x^2+3}\ge\sqrt{3},\forall x\)Vậy minC = \(\sqrt{3}\)khi x = 0
22 tháng 1 2021
Do \(a,b,c\geq 0\) và \(a+b+c=1\) nên \(a,b,c\le1\).
Xét hiệu \(5a+4-\left(a+2\right)^2=a\left(1-a\right)\ge0\)
\(\Rightarrow5a+4\ge\left(a+2\right)^2\)
\(\Rightarrow\sqrt{5a+4}\ge a+2\).
Tương tự, \(\sqrt{5b+4}\ge b+2;\sqrt{5c+4}\ge c+2\).
Cộng vế với vế ta có \(T\ge a+b+c+6=7\).
Đẳng thức xảy ra khi a = 1; b = c = 0 và các hoán vị.
Vậy Min T = 7 khi a = 1; b = c = 0.
T
22 tháng 1 2021
Một ý tưởng để có được bất đẳng thức phụ \(\sqrt{5a+4}\ge a+2\forall0\le a\le1.\)
Do $0\leq a \leq 1$ nên $a\ge a^2.$
Ta có: \(\sqrt{5a+4}=\sqrt{a+4a+4+\ 4}\ge\sqrt{a^2+4a+4+4}=a+2\)
Ngoài ra còn một cách là giả sử \(\sqrt{5a+4}\ge ma+n\)
rồi đi chọn $m,n$ theo điểm rơi.
Không biết còn cách nào khác không nhỉ?