Tìm GTLN -5x² -4x +2
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Bài 2:
a: Ta có: \(x^2+4x+7\)
\(=x^2+4x+4+3\)
\(=\left(x+2\right)^2+3\ge3\forall x\)
Dấu '=' xảy ra khi x=-2

\(A=\frac{5x^2+4x-1}{x^2}=\frac{9x^2-\left(4x^2-4x+1\right)}{x^2}=9-\frac{\left(2x-1\right)^2}{x^2}\le9\)
Dấu \(=\)khi \(2x-1=0\Leftrightarrow x=\frac{1}{2}\).
\(B=\frac{x^2}{x^2+x+1}=\frac{3x^2}{3x^2+3x+3}=\frac{4x^2+4x+4-\left(x^2+4x+4\right)}{3x^2+3x+3}=\frac{4}{3}-\frac{\left(x+2\right)^2}{3\left(x^2+x+1\right)}\le\frac{4}{3}\)
Dấu \(=\)khi \(x+2=0\Leftrightarrow x=-2\).

Ta có:
\(B=-5x^2-4x+1\)
\(=\left(4x^2-4x+1\right)-9x^2\)
\(=\left(2x-1\right)^2-\left(3x\right)^2\)
\(=\left(2x-1+3x\right)\left(2x-1-3x\right)\)
\(=-\left(x+1\right)\left(5x-1\right)\)
\(B=-5x^2-4x+1\)
\(B=-5\left(x^2+\frac{4}{5}x-\frac{1}{5}\right)\)
\(B=-5\left[x^2+2.x.\frac{2}{5}+\left(\frac{2}{5}\right)^2-\frac{9}{25}\right]\)
\(B=-5\left(x+\frac{2}{5}\right)^2+5.\frac{9}{25}\)
\(B=-5\left(x+\frac{2}{5}\right)^2+\frac{9}{5}\)
Ta có: \(\left(x+\frac{2}{5}\right)^2\ge0\forall x\)
\(\Rightarrow-5.\left(x+\frac{2}{5}\right)^2\le0\forall x\)
\(\Rightarrow-5.\left(x+\frac{2}{5}\right)^2+\frac{9}{5}\le\frac{9}{5}\forall x\)
\(B=\frac{9}{5}\Leftrightarrow-5.\left(x+\frac{2}{5}\right)^2=0\Leftrightarrow x+\frac{2}{5}=0\Leftrightarrow x=-\frac{2}{5}\)
Vậy \(B_{max}=\frac{9}{5}\Leftrightarrow x=-\frac{2}{5}\)
Tham khảo nhé~

Ta có: A = 2x2 - 5x - 8 = 2(x2 - 5/2x + 25/16) - 89/8 = 2(x - 5/4)2 - 89/8
Ta luôn có: 2(x - 5/4)2 \(\ge\)0 \(\forall\)x
=> 2(x - 5/4)2 - 89/8 \(\ge\)-89/8 \(\forall\)x
Dấu "=" xảy ra <=> x - 5/4 = 0 <=> x = 5/4
Vậy Min của A = -89/8 tại x = 5/4
Ta có: B = -x2 - 4x + 3 = -(x2 + 4x + 4) + 7 = -(x + 2)2 + 7
Ta luôn có: -(x + 2)2 \(\le\)0 \(\forall\)x
=> -(x + 2)2 + 7 \(\le\)7 \(\forall\)x
Dấu "=" xảy ra <=> x + 2 = 0 <=> x = -2
Vậy Max của B = 7 tại x = -2

\(giải:\)
\(-4x^2+5x+1\)
\(=-4x^2+5x-\frac{25}{16}+\frac{41}{16}\)
\(=\left(-4x^2+5x-\frac{25}{16}\right)+\frac{41}{16}\)
\(=-\left(4x^2-5x+\frac{25}{16}\right)+\frac{41}{16}\)
\(=-\left[\left(2x\right)^2-2.2x.\frac{5}{4}+\left(\frac{5}{4}\right)^2\right]+\frac{41}{16}\)
\(=-\left(2x-\frac{5}{4}\right)^2+\frac{41}{16}\le\frac{41}{16}\)
\(GTLN\) \(của\)\(-4x^2+5x+1=\frac{41}{16}\)\(đạt\)\(khi\)\(-\left(2x-\frac{5}{4}\right)^2=0\)
\(\Leftrightarrow2x-\frac{5}{4}=0\)
\(\Leftrightarrow2x=\frac{5}{4}\Leftrightarrow x=\frac{5}{8}\)
vậy gtln của -4x^2+5x+1 bằng 41/16 tại x=5/8


A = - 4\(x\)2 + 5\(x\) - 3
A = -( 4\(x^2\) - 5\(x\) + \(\dfrac{25}{16}\)) - \(\dfrac{23}{16}\)
A = -( 2\(x\) - \(\dfrac{5}{4}\))2 - \(\dfrac{23}{16}\)
Vì ( 2\(x\) - \(\dfrac{5}{4}\))2 ≥ 0; ⇒ - ( 2\(x\) - \(\dfrac{5}{4}\))2 ≤ 0 ⇒ -( 2 \(x\) - \(\dfrac{5}{4}\))2 - \(\dfrac{23}{16}\) ≤ - \(\dfrac{23}{16}\)
A(max) = - \(\dfrac{23}{16}\) ⇔ 2\(x\) - \(\dfrac{5}{4}\) = 0 ⇔ \(x\) = \(\dfrac{5}{4}\): 2 = \(\dfrac{5}{8}\)
Kết luận giá trị lớn nhất của biểu thức là - \(\dfrac{23}{16}\) xáy ra khi \(x\) = \(\dfrac{5}{8}\)

Ta có:
\(I=y^2+4x+5x^2-2xy\)
\(I=4x^2+4x+1+x^2-2xy+y^2-1\)
\(I=\left(2x+1\right)^2+\left(x-y\right)^2-1\)
Mà: \(\hept{\begin{cases}\left(2x+1\right)^2\ge0\\\left(x-y\right)^2\ge0\end{cases}\Rightarrow\left(2x+1\right)^2+\left(x-y\right)^2\ge0\left(\forall x,y\in R\right)}\)
\(\Rightarrow I=\left(2x+1\right)^2+\left(x-y\right)^2-1\ge-1\)
Dấu "=" xảy ra khi \(\hept{\begin{cases}2x+1=0\\x-y=0\end{cases}\Leftrightarrow\hept{\begin{cases}x=-\frac{1}{2}\\x=y\end{cases}\Leftrightarrow}x=y=-\frac{1}{2}}\)
Vậy Min I = -1 khi x = y = -1/2
Bài này không suy ra được GTLN nha bạn
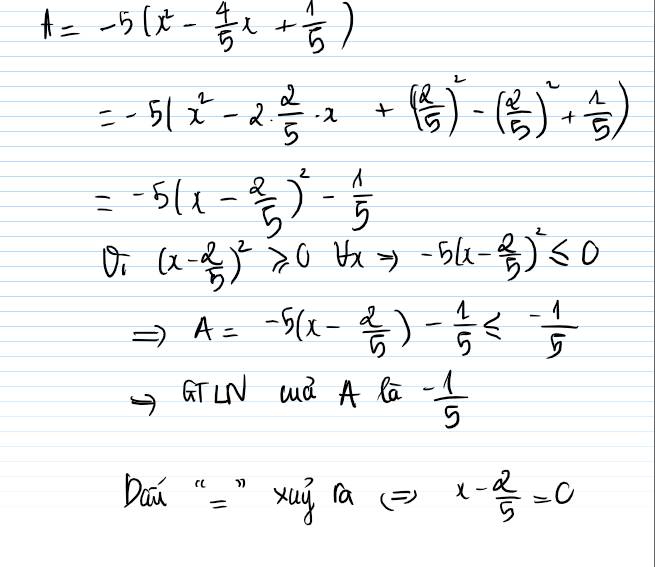
Con xin lỗi con ghi sai đề ạ . Có thể giải lại giúp con không ạ