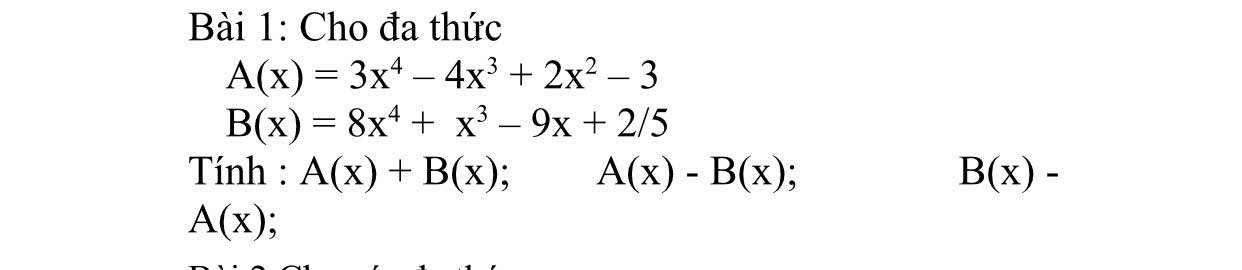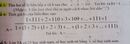
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


1 A
2 C
3 C
4 C
5 B
5 C
5 B
6 A
C
I
1 - C
2 - D
3 - B
4 - A
II
1 People of ethnic minorities
2 In March of every year
3 Money and other things
4 No, they aren't
5 Around all the villages
D
1 play volleyball
2 are 50 teachers and 800 students in my school
3 terrible weather
4 works fewer hours than ,e
II
1 performance
2 cultural
3 seasonal
4 carelessly


II Grammar and....
choose the correct answer
3.D
4.A
5.B
6.C
7.C
8.C
C.Reading
read the...
1.B
2.C
3.D
4.B

\(A\left(x\right)+B\left(x\right)=3x^4-4x^3+2x^2-3+8x^4+x^3-9x+\dfrac{2}{5}\)
\(=11x^4-3x^3+2x^2-9x-\dfrac{13}{5}\)
\(A\left(x\right)-B\left(x\right)=3x^4-4x^3+2x^2-3-8x^4-x^3+9x-\dfrac{2}{5}\)
\(=-5x^4-5x^3+2x^2+9x-\dfrac{17}{5}\)
\(B\left(x\right)-A\left(x\right)=8x^4+x^3-9x+\dfrac{2}{5}-3x^4+4x^3-2x^2+3\)
\(=5x^4+5x^3-2x^2-9x+\dfrac{17}{5}\)

a) Ta có: \(P\left(x\right)=3x^5-2x^4-2x^5-2x^2-x+3x^2+2\)
\(=x^5-2x^4+x^2+2\)
Ta có: \(Q\left(x\right)=x^5+8-5x+3x^3+3x+x^4-4x^5\)
\(=-3x^5+x^4+3x^3-2x+8\)
b) \(P\left(x\right)+Q\left(x\right)=x^5-2x^4+x^2+2-3x^5+x^4+3x^3-2x+8\)
\(=-2x^5-x^4+3x^3+x^2-2x+10\)
\(P\left(x\right)-Q\left(x\right)=x^5-2x^4+x^2+2+3x^5-x^4-3x^3+2x-8\)
\(=4x^5-3x^4-3x^3+x^2+2x-6\)


Vì Om, On đối Oy, Ox nên \(\widehat{xOy}=\widehat{mOn}=45^0\left(đối.đỉnh\right)\)
Ta có \(\widehat{xOy}+\widehat{xOm}=180^0\left(kề.bù\right)\Rightarrow\widehat{xOm}=180^0-45^0=135^0\)
Vì Om, On đối Oy, Ox nên \(\widehat{xOm}=\widehat{yOn}=135^0\left(đối.đỉnh\right)\)
ta có : mOn=xOy=45o
Do xOy và xOm kề bù nên:
xOy+xOm=180o
suy ra:xOm=180o - xOy=135o
Mà yOn và xOm đối đỉnh nên
yOn=xOm=135o

mk đi chơi nô en cũng thấy chán,ko bằng tết của bọn mk đâu

a.
Do chóp tứ giác đều \(\Rightarrow\Delta SAC\) cân tại A
Mà O là tâm đáy \(\Rightarrow O\) là trung điểm AC
\(\Rightarrow SO\perp AC\) (trung tuyến đồng thời là đường cao trong tam giác cân)
Hoàn toàn tương tự, ta có \(SO\perp BD\)
\(\Rightarrow SO\perp\left(ABCD\right)\)
b.
Ta có: \(AC\perp BD\) (hai đường chéo hình vuông)
Theo cmt, \(SO\perp AC\)
\(\left\{{}\begin{matrix}SO\in\left(SBD\right)\\BD\in\left(SBD\right)\end{matrix}\right.\) \(\Rightarrow AC\perp\left(SBD\right)\)
Tương tự: \(\left\{{}\begin{matrix}BD\perp AC\\BD\perp SO\left(cmt\right)\end{matrix}\right.\) \(\Rightarrow BD\perp\left(SAC\right)\)