Giải phương trình:
4cosx-2cos2x-cos4x=0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
HG
Hân Gia Bảo
11 tháng 10 2017
Đúng(0)
Những câu hỏi liên quan
14 tháng 8 2017
\(4cosx-2cos2x-cos4x=1\)
\(\Leftrightarrow4cosx-2cos2x-\left(2cos^22x-1\right)=1\)
\(\Leftrightarrow4cosx-2cos2x-2cos^22x=0\)
\(\Leftrightarrow4cosx-2cos2x\cdot\left(1+cos2x\right)=0\)
\(\Leftrightarrow4cosx-2cos2x\cdot2cos^2x=0\)
\(\Leftrightarrow2cosx\cdot\left(2-2cos2x\cdot cosx\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}cosx=0\rightarrow x=\dfrac{\pi}{2}+k\pi\left(k\in Z\right)\\2-2cos2x\cdot cosx=0\end{matrix}\right.\)
\(\Leftrightarrow2cos2x\cdot cosx=2\)
\(\Leftrightarrow cos2x\cdot cosx=1\)
\(\Leftrightarrow\left(2cos^2x-1\right)\cdot cosx-1=0\)
\(\Leftrightarrow2cos^3x-cosx-1=0\)
\(\Leftrightarrow cosx=1\)
\(\Leftrightarrow x=k2\pi\) \(\left(k\in Z\right)\)
16 tháng 8 2021
a) <=> 4sinxcosx -(2cos2x-1)=7sinx+2cosx-4
<=> 2cos2x+(2-4sinx)cosx+7sinx-5=0
- sinx=1 => 2cos2x-2cosx+2=0
pt trên vn
16 tháng 8 2021
b) <=> 2sinxcosx-1+2sin2x+3sinx-cosx-1=0
<=> cos(2sinx-1)+2sin2x+3sinx-2=0
<=> cosx(2sinx-1)+(2sinx-1)(sinx+2)=0
<=> (2sinx-1)(cosx+sinx+2)=0
<=> sinx=1/2 hoặc cosx+sinx=-2(vn)
<=> x= \(\frac{\pi}{6}+k2\pi\) hoặc \(x=\frac{5\pi}{6}+k2\pi\left(k\in Z\right)\)
CM
6 tháng 11 2019
Phương pháp:
Biến đổi về phương trình bậc 2 đối với cos2x. Sử dụng công thức nhân đôi: cos 2 x = cos 2 x − sin 2 x
Cách giải:
Ta có:
2 cos 2 x + 5 sin 4 x − cos 4 x + 3 = 0 ⇔ 2 cos 2 x + 5 sin 2 x − cos 2 x sin 2 x + cos 2 x + 3 = 0
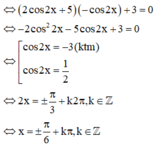
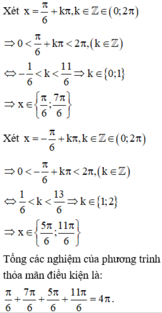
Chọn: A
CM
29 tháng 8 2019
Đáp án B.
PT ⇔ 2 cos 2 x + 5 sin 2 x - cos 2 x sin 2 x + cos 2 x + 3 = - 2 cos 2 x + 5 cos 2 x + 3 = 0
⇔ 2 cos 2 2 x + 5 cos 2 x - 3 = 0 ⇔ [ cos 2 x = - 3 ( ! ) cos 2 x = 1 2 ⇔ 2 x = ± π 3 + k 2 π
⇔ x = ± π 3 + k π ∈ 0 ; 2 π ⇔ x ∈ π 6 ; 5 π 6 ; 7 π 6 ; 11 π 6 ⇒ S = 4 π .
