tìm x nguyên để A=3x+1/x+1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
27 tháng 1 2018
a) \(ĐKXĐ:\hept{\begin{cases}3x\ne0\\x+1\ne0\\2-4x\ne0\end{cases}}\Leftrightarrow\hept{\begin{cases}x\ne0\\x\ne-1\\x\ne\frac{1}{2}\end{cases}}\)
\(A=\left(\frac{x+2}{3x}+\frac{2}{x+1}-3\right):\frac{2-4x}{x+1}-\frac{3x+1-x^2}{3x}\)
\(=\left[\frac{\left(x+1\right)\left(x+2\right)}{3x\left(x+1\right)}+\frac{6x}{3x\left(x+1\right)}-\frac{9x\left(x+1\right)}{3x\left(x+1\right)}\right]:\frac{2\left(1-2x\right)}{x+1}-\frac{3x+1-x^2}{3x}\)
\(=\frac{\left(x+1\right)\left(x+2\right)+6x-9x\left(x+1\right)}{3x\left(x+1\right)}.\frac{x+1}{2\left(1-2x\right)}-\frac{3x+1-x^2}{3x}\)
\(=\frac{2-8x^2}{3x\left(x+1\right)}.\frac{x+1}{2\left(1-2x\right)}-\frac{3x+1-x^2}{3x}\)
\(=\frac{1+2x-3x-1+x^2}{3x}\)
\(=\frac{x\left(x-1\right)}{3x}=\frac{x-1}{3}\)
27 tháng 1 2018
b)\(\text{Với }x\ne0,x\ne-1,x\ne\frac{1}{2}\text{ ta có:}\)
\(\text{Để A< 0\Leftrightarrow}\frac{x-1}{3}< 0\Rightarrow x-1< 0\Leftrightarrow x< 1\)
CM
20 tháng 6 2018
b) Tìm x nguyên để A nguyên
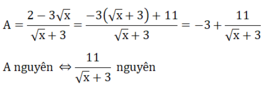
⇔ x + 3 ∈ Ư(11) ⇔ x + 3 ∈ {-11; -1; 1; 11}
Do x + 3 ≥ 3 nên x + 3 = 11 ⇔ x = 8 ⇔ x = 64
Vậy với x = 64 thì A nguyên
14 tháng 1 2021
a)
ĐKXĐ: \(x\notin\left\{0;3;-3\right\}\)
Ta có: \(A=\left(\dfrac{1}{3}+\dfrac{3}{x^2-3x}\right):\left(\dfrac{x^2}{27-3x^2}+\dfrac{1}{x+3}\right)\)
\(=\left(\dfrac{1}{3}+\dfrac{3}{x\left(x-3\right)}\right):\left(\dfrac{-x^2}{3\left(x-3\right)\left(x+3\right)}+\dfrac{1}{x+3}\right)\)
\(=\left(\dfrac{x\left(x-3\right)}{3x\left(x-3\right)}+\dfrac{9}{3x\left(x-3\right)}\right):\left(\dfrac{-x^2}{3\left(x-3\right)\left(x+3\right)}+\dfrac{3\left(x-3\right)}{3\left(x-3\right)\left(x+3\right)}\right)\)
\(=\dfrac{x^2-3x+9}{3x\left(x-3\right)}:\dfrac{-x^2+3x-9}{3\left(x-3\right)\left(x+3\right)}\)
\(=\dfrac{x^2-3x+9}{3x\left(x-3\right)}\cdot\dfrac{3\left(x-3\right)\left(x+3\right)}{-\left(x^2-3x+9\right)}\)
\(=\dfrac{-x-3}{x}\)
b) Để A nguyên thì \(-x-3⋮x\)
mà \(-x⋮x\)
nên \(-3⋮x\)
\(\Leftrightarrow x\inƯ\left(-3\right)\)
\(\Leftrightarrow x\in\left\{1;-1;3;-3\right\}\)
Kết hợp ĐKXĐ, ta được: \(x\in\left\{1;-1\right\}\)
Vậy: Để A nguyên thì \(x\in\left\{1;-1\right\}\)
2 tháng 1 2023
a: ĐKXĐ: x<>1; x<>-1
b: \(A=\dfrac{\left(x-4\right)\left(x+1\right)}{\left(x-1\right)\left(x+1\right)}=\dfrac{x-4}{x-1}\)
c: Để A là số nguyên thì x-1-3 chia hết cho x-1
=>\(x-1\in\left\{1;-1;3;-3\right\}\)
=>\(x\in\left\{2;0;4;-2\right\}\)
ML
1
ML
16 tháng 8 2023
\(A=\dfrac{3x+5}{x-1}=\dfrac{3x-3+8}{x-1}=\dfrac{3\left(x-1\right)+8}{x-1}=\dfrac{3\left(x-1\right)}{x-1}+\dfrac{8}{x-1}=3+\dfrac{8}{x-1}\)
A là số nguyên => x - 1 \(\in\) Ư(8) = {-8,-4,-2,-1,1,2,4,8}
Ta có bảng:
| x-1 | -8 | -4 | -2 | -1 | 1 | 2 | 4 | 8 |
| x | -7 | -3 | -1 | 0 | 2 | 3 | 5 | 9 |
Vậy với giá trị x = 9 sẽ là giá trị x lớn nhất để A là số nguyên.
Ta có:
\(A=\frac{3x+1}{x+1}=\frac{3x+3-2}{x+1}=3-\frac{2}{x+1}\)
Để A nguyên thì \(x+1\inƯ\left(2\right)\in\left\{1,2,-1,-2\right\}\)
x + 1 = 1 => x = 0
x + 1 = 2 => x = 1
x + 1 = -1 => x = -2
x + 1 = -2 => x = -3
Vậy \(x\in\left\{0;1;-2;-3\right\}\)thì A nguyên