HT
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
PT
1
JK
0
HP
17 tháng 9 2021
a, Hàm số xác định khi: \(\left\{{}\begin{matrix}cos\dfrac{x}{2}\ne3\\tanx\ne\sqrt{3}\\cosx\ne0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\ne\dfrac{\pi}{3}+k\pi\\x\ne\dfrac{\pi}{2}+k\pi\end{matrix}\right.\)
HP
26 tháng 9 2021
Hàm số xác định khi: \(\left\{{}\begin{matrix}tanx\ne\pm1;cosx\ne0\\cosx\ne-1\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\ne\pm\dfrac{\pi}{4}+k\pi\\x\ne\dfrac{\pi}{2}+k\pi\\x\ne\pi+k2\pi\end{matrix}\right.\)
AH
Akai Haruma
Giáo viên
29 tháng 7 2020
Bạn chú ý gõ đề bằng công thức toán (hộp biểu tượng $\sum$) trên thanh công cụ. Nhìn đề rối mắt thế này thật tình không ai muốn đọc chứ đừng nói đến giúp =)))
HQ
Hà Quang Minh
Giáo viên
25 tháng 8 2023
a, ĐK: \(cos\left(x\right)\ne0\Leftrightarrow x\ne\dfrac{\pi}{2}+k\pi\)
Vậy tập xác định của hàm số là: \(D=R\backslash\left\{\dfrac{\pi}{2}+k\pi,k\in Z\right\}\)
b, ĐK: \(cos\left(x+\dfrac{\pi}{4}\right)\ne0\Leftrightarrow x+\dfrac{\pi}{4}\ne\dfrac{\pi}{2}+k\pi\Leftrightarrow x\ne\dfrac{\pi}{4}+k\pi\)
Vậy tập xác định của hàm số là \(D=R\backslash\left\{\dfrac{\pi}{4}+k\pi,k\in Z\right\}\)
c, ĐK: \(2-sin^2\left(x\right)\ne0\Leftrightarrow sin^2\left(x\right)\ne2\)
Vì \(0\le sin^2\left(x\right)\le1\Rightarrow sin^2\left(x\right)\ne2\forall x\)
Vậy tập xác định của hàm số là \(D=R\)
PT
1
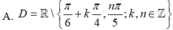
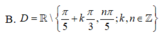
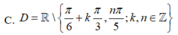
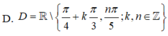
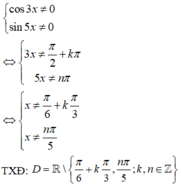
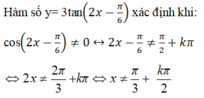
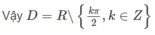
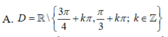
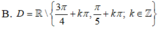
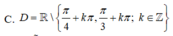
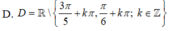
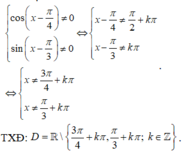
\(\hept{\begin{cases}\cos x\ne0\\tan2x+1\ne0,cos2x\ne0\end{cases}\Leftrightarrow\hept{\begin{cases}x\ne\frac{\pi}{2}+k\pi\\x\ne-\frac{\pi}{8}+\frac{k\pi}{2}\\x\ne\frac{\pi}{4}+\frac{k\pi}{2}\end{cases}}}\)