K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
15 tháng 8
A = - 3\(x\).(\(x-5\)) + 3(\(x^2\) - 4\(x\)) - 3\(x\) - 10
A = - 3\(x^2\) + 15\(x\) + 3\(x^2\) - 12\(x\) - 3\(x\) - 10
A = (- 3\(x^2\) + 3\(x^2\)) + (15\(x\) - 12\(x\) - 3\(x\)) - 10
A = 0 + (3\(x-3x\)) - 10
A = 0 - 10
A = - 10
YT
1
16 tháng 10 2023
`#3107.101107`
`1/2x + 4/5 = 2x - 8/5`
`=> 1/2x - 2x = -4/5 - 8/5`
`=> -3/2x = -12/5`
`=> x = -12/5 \div (-3/2)`
`=> x = 8/5`
Vậy, `x = 8/5`
_____
`\sqrt{x} = 5`
`=> x = 5^2`
`=> x = 25`
Vậy, `x = 25`
___
`x^2 = 3`
`=> x^2 = (+-\sqrt{3})^2`
`=> x = +- \sqrt{3}`
Vậy, `x \in {-\sqrt{3}; \sqrt{3}}.`
24 tháng 10 2023
Dễ
Thế
Mà
Cũnhoir
Dc
Ạ
Chịu
Chắc
Phải
Ngu
Lamqs
Mới
Hỏi
Câu
Này
26 tháng 7 2023
a: K(x)=0
=>x=0 hoặc x+5=0
=>x=0 hoặc x=-5
b: K(x)=0
=>x(2x-5)(x+3)=0
=>x=0 hoặc 2x-5=0 hoặc x+3=0
=>x=0;x=5/2;x=-3
c: K(x)=0
=>x(x^2+4)(2x+1)=0
=>x(2x+1)=0
=>x=0 hoặc x=-1/2
d: G(x)=0
=>(x-3)(x+3)=0
=>x=3 hoặc x=-3
e: G(x)=0
=>x(x^2-25)=0
=>x(x-5)(x+5)=0
=>x=0;x=5;x=-5
8 tháng 11 2021
c:
Trường hợp 1: x<-3
\(\Leftrightarrow-x-3-x-1=3x\)
\(\Leftrightarrow-5x=4\)
hay \(x=-\dfrac{4}{5}\left(loại\right)\)
Trường hợp 2: -3<=x<-1
\(\Leftrightarrow x+3-x-1=3x\)
hay \(x=\dfrac{2}{3}\left(loại\right)\)
Trường hợp 3: x>=-1
\(\Leftrightarrow2x+4=3x\)
hay x=4(nhận)
H9
HT.Phong (9A5)
CTVHS
27 tháng 6 2023
Bài 1:
a) \(3x^2\left(2x^3-x+5\right)-6x^5-3x^3+10x^2\)
\(=6x^5-3x^3+10x^2-6x^5-3x^3+10x^2\)
\(=10x^2+10x^2\)
\(=20x^2\)
b) \(-2x\left(x^3-3x^2-x+11\right)-2x^4+3x^3+2x^2-22x\)
\(=-2x^4+6x^3+2x^2-22x-2x^4+3x^3+2x^2-22x\)
\(=-4x^4+9x^3+4x^2-44x\)
24 tháng 4 2022
a) A(x) = 2x3 + 5 + x2 - 3x - 5x3 - 4
= 2x3 - 5x3 + x2 - 3x + 5 - 4
= -3x3 + x2 - 3x + 1
B(x) = -3x4 - x3 + 2x2 + 2x + x4 - 4 - x2
= -3x4 + x4 - x3 + 2x2 - x2 + 2x - 4
= -2x4 - x3 + x2 + 2x - 4
b)
H(x) = A(x) - B(x)
H(x) = (-3x3 + x2 - 3x + 1) - (-2x4 - x3 + x2 + 2x - 4)
= -3x3 + x2 - 3x + 1 + 2x4 + x3 - x2 - 2x + 4
= 2x4 - 3x3 + x3 + x2 - x2 - 3x - 2x + 1 + 4
= 2x4 - 2x3 -5x + 5
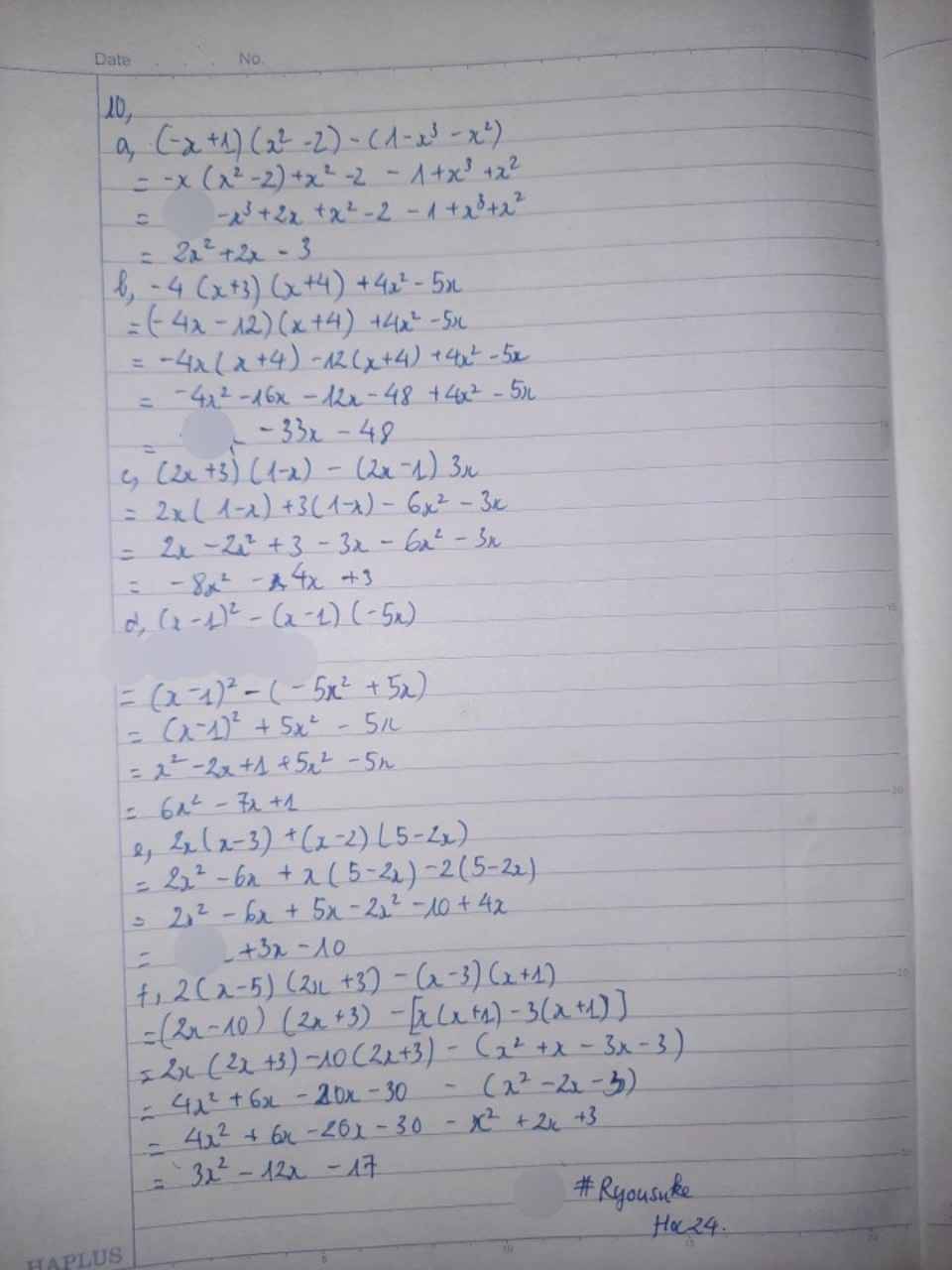
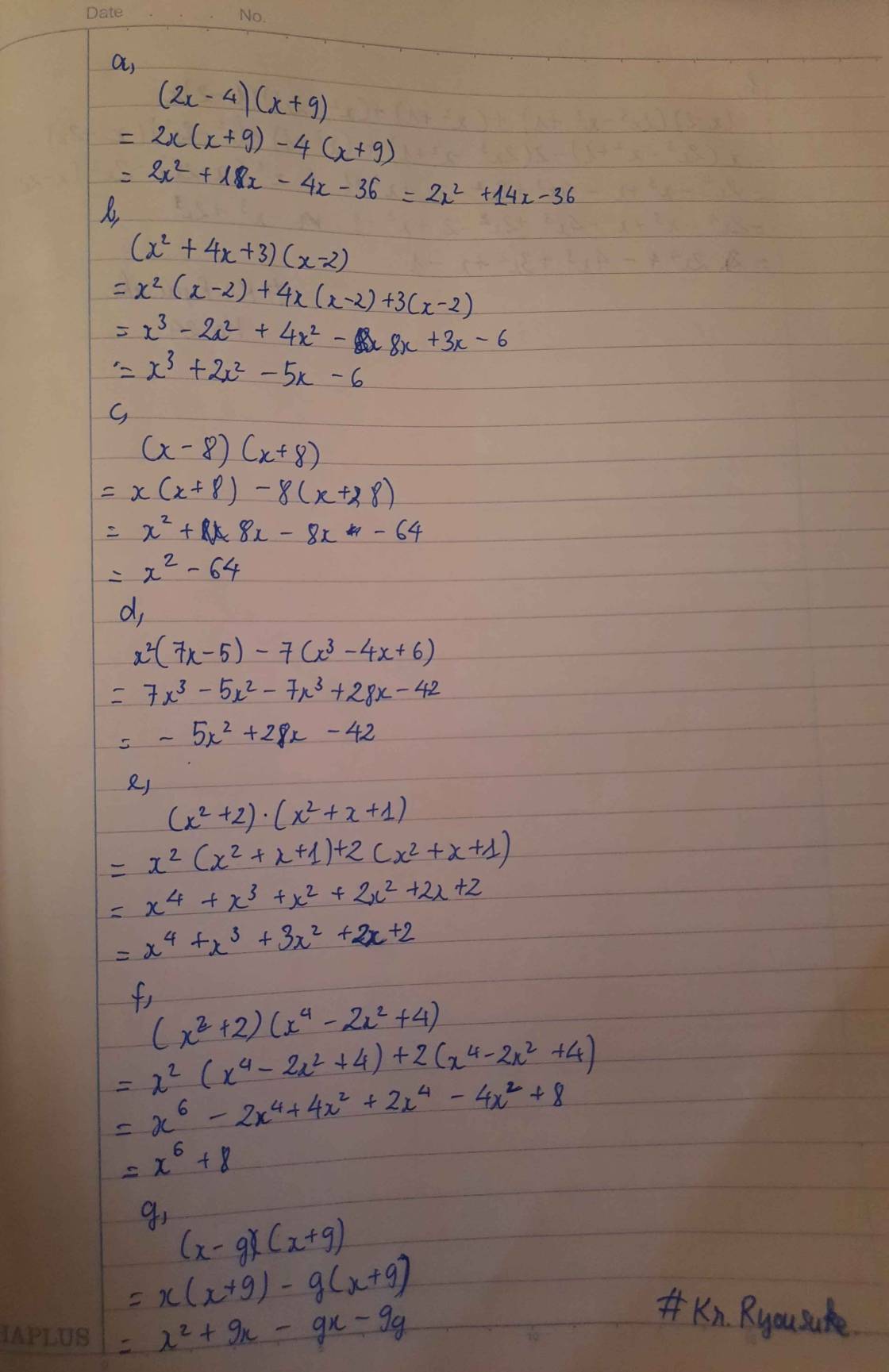
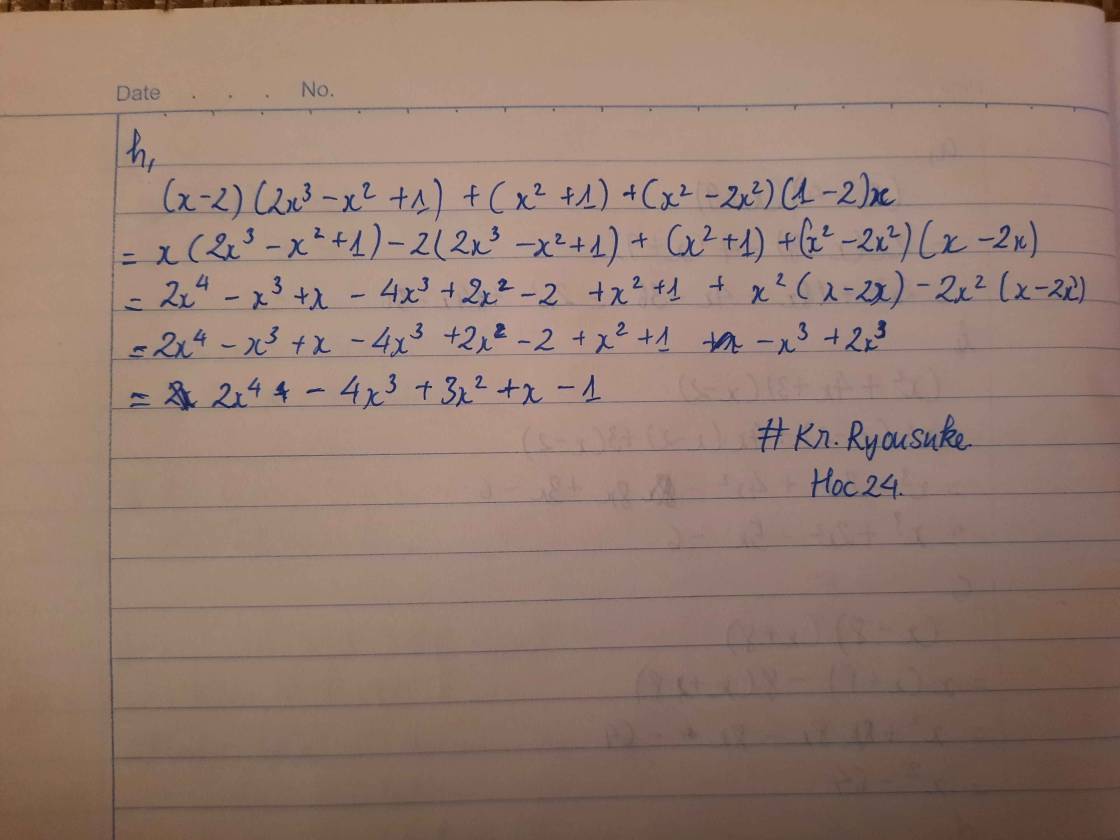
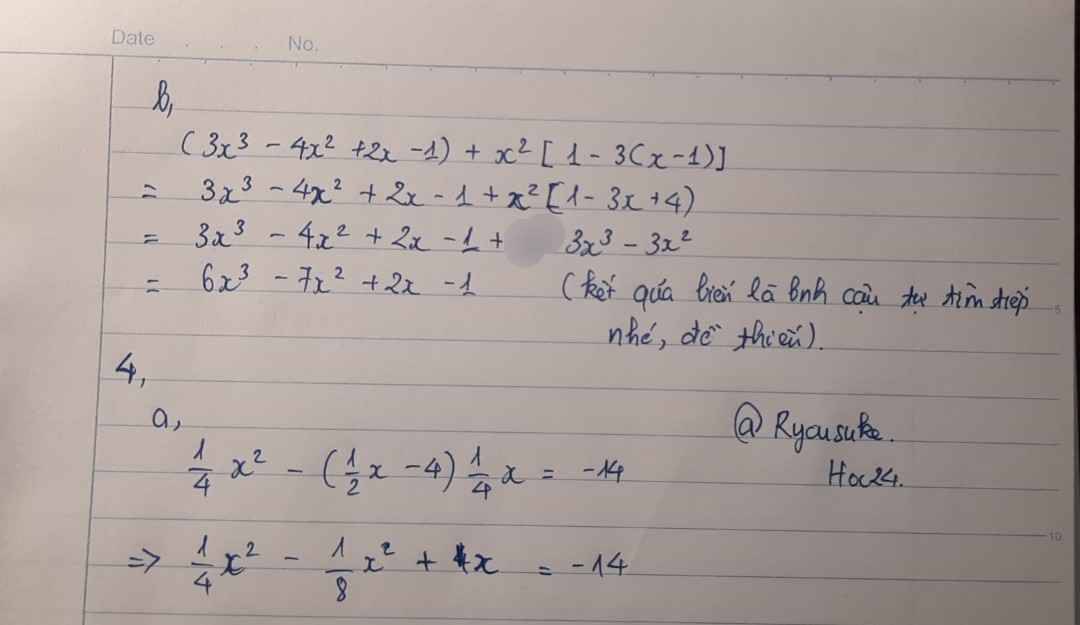
\(x\left(x-5\right)\left(x+5\right)-\left(x+2\right)\left(x^2-2x+4\right)=3\)
\(\Rightarrow x\left(x^2+5x-5x-25\right)-\left(x^3-2x^2+4x+2x^2-4x+8\right)=3\)
\(\Rightarrow x^3+5x^2-5x^2-25x-x^3+2x^2-4x-2x^2+4x-8=3\)
\(\Rightarrow\left(x^3-x^3\right)+\left(5x^2-5x^2+2x^2-2x^2\right)+\left(-25x-4x+4x\right)-8=3\)
\(\Rightarrow-25x=3+8=11\)
\(\Rightarrow x=\frac{11}{-25}=\frac{-11}{25}\)
Vay x = \(\frac{-11}{25}\)
\(x\left(x-5\right)\left(x+5\right)-\left(x+2\right)\left(x^2-2x+4\right)=3\)
\(\Leftrightarrow x^3-25x-\left(x^3-2x^2+4x+2x^2-4x+8\right)=3\)
\(\Leftrightarrow x^3-25x-x^3+2x^2-4x-2x^2+4x-8-3=0\)
\(\Leftrightarrow-25x-11=0\)
\(\Leftrightarrow-25x=11\)
\(\Leftrightarrow x=-\frac{11}{25}\)