Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

x2-8x-15=x2-8x+16-31=(x-4)2-31=\(\left(x-4-\sqrt{31}\right).\left(x-4+\sqrt{31}\right)\)

x^3-2x-4
=x^3-2x-8+4 (Ta thấy - 8 + 4 là bằng -4 nên ta thêm vào thì cũng giống nhau)
=(x^3-8)-(2x-4) (Nhóm hạng tử)
=(x-2)(x^2+2x+4)-2(x-2) \([\)(Hằng đẳng thức 6) và ta thấy -2 là nhân tử chung\(]\)
=(x-2)(x^2+2x+4-x+2) (Rút gọn)
=(x-2)(x^2+x+6)

a) ( x-2 )( x - 4 )( x - 6 )( x -8 ) + 15
= ( x- 2 )( x - 8 )( x - 4)( x- 6 ) + 15
= ( x^2 - 10x + 16 )( x^2 - 10x + 24 ) + 15
Đắt x^2 + x + 16 = y
= y ( y + 8 ) + 15
= y^2 + 8y + 15
= y^2 + 3y + 5y + 15
=y ( y + 3 ) + 5 ( y + 3 )
= ( y+ 5)( y + 3)
Thay vào

Áp dụng \(\left(a+b\right)^3=a^3+b^3+3ab\left(a+b\right)\)
\(\left(x+y+z\right)^3-x^3-y^3-z^3\)
\(=\left[\left(x+y\right)+z\right]^3-x^3-y^3-z^3\)
\(=\left(x+y\right)^3+z^3+3z\left(x+y\right)\left(x+y+z\right)-x^3-y^3-z^3\)
\(=x^3+y^3+3xy\left(x+y\right)+3z\left(x+y\right)\left(x+y+z\right)-x^3-y^3\)
\(=3\left(x+y\right)\left(xy+xz+yz+z^2\right)\)
\(=3\left(x+y\right)\left[x\left(y+z\right)+z\left(y+z\right)\right]\)
\(=3\left(x+y\right)\left(y+z\right)\left(z+x\right)\)

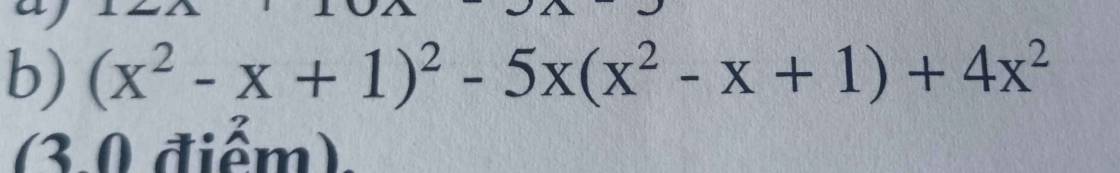
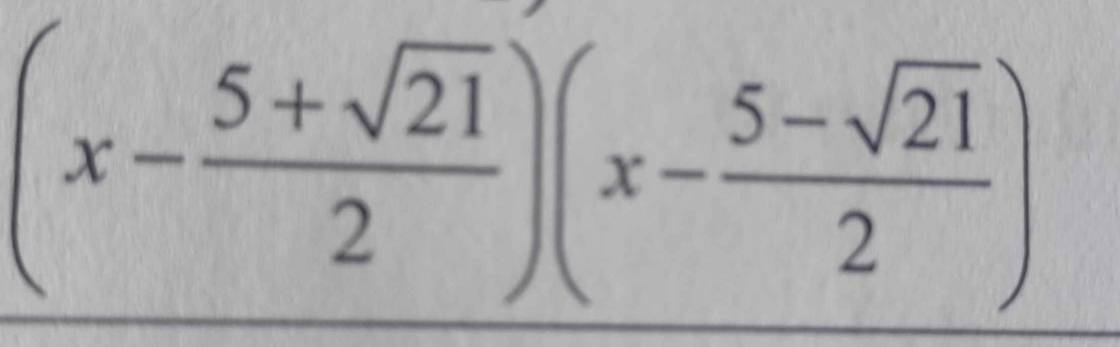
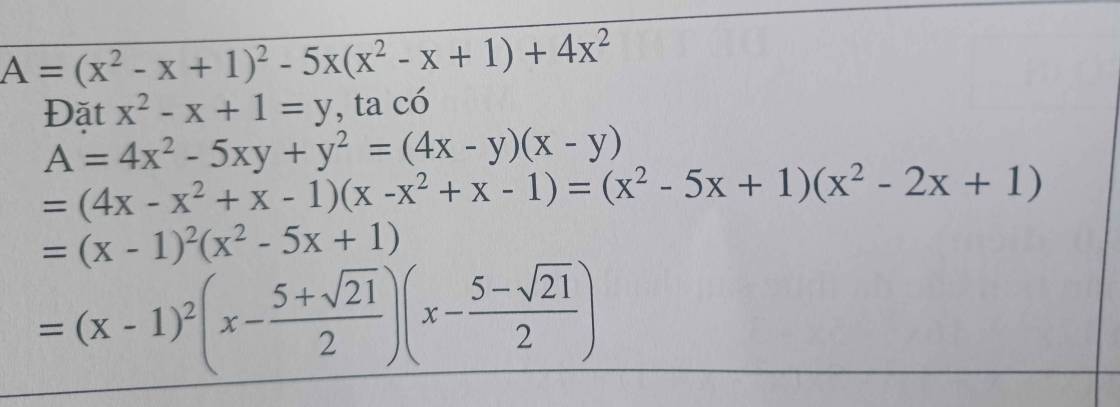
\(x^2-x-30\)
\(=x^2+5x-6x-30\)
\(=x\left(x+5\right)-6\left(x+5\right)\)
\(=\left(x+5\right)\left(x-6\right)\)
\(x^2+5x-6x-30\)
\(=x\left(x+5\right)-6\left(x+5\right)\)
\(=\left(x-6\right)\left(x+5\right)\)