
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.



a, Đặt \(x^2-4x+8=a\left(a>0\right)\)
\(\Rightarrow a-2=\frac{21}{a+2}\)
\(\Leftrightarrow a^2-4=21\Rightarrow a^2=25\Rightarrow a=5\)
Thay vào là ra
b) ĐK: \(y\ne1\)
bpt <=> \(\frac{4\left(1-y\right)}{1-y^3}+\frac{1+y+y^2}{1-y^3}+\frac{2y^2-5}{1-y^3}\le0\)
<=> \(\frac{3y^2-3y}{1-y^3}\le0\)
\(\Leftrightarrow\frac{y\left(y-1\right)}{\left(y-1\right)\left(y^2+y+1\right)}\ge0\)
\(\Leftrightarrow\frac{y}{y^2+y+1}\ge0\)
vì \(y^2+y+1=\left(y+\frac{1}{2}\right)^2+\frac{3}{4}>0\)
nên bpt <=> \(y\ge0\)


1) \(\frac{x-1}{x+3}-\frac{x}{x-3}=\frac{4x+15}{9-x^2}\)
ĐKXĐ : \(x\ne\pm3\)
\(\Leftrightarrow\frac{x-1}{x+3}-\frac{x}{x-3}=\frac{-4x-15}{x^2-9}\)
\(\Leftrightarrow\frac{\left(x-1\right)\left(x-3\right)}{\left(x-3\right)\left(x+3\right)}-\frac{x\left(x+3\right)}{\left(x-3\right)\left(x+3\right)}=\frac{-4x-15}{\left(x-3\right)\left(x+3\right)}\)
\(\Leftrightarrow\frac{x^2-4x+3}{\left(x-3\right)\left(x+3\right)}-\frac{x^2+3x}{\left(x-3\right)\left(x+3\right)}=\frac{-4x-15}{\left(x-3\right)\left(x+3\right)}\)
\(\Leftrightarrow\frac{x^2-4x+3-x^2-3x}{\left(x-3\right)\left(x+3\right)}=\frac{-4x-15}{\left(x-3\right)\left(x+3\right)}\)
\(\Leftrightarrow-7x+3=-4x-15\)
\(\Leftrightarrow-7x+4x=-15-3\)
\(\Leftrightarrow-3x=-18\)
\(\Leftrightarrow x=6\)( tmđk )
Vậy x = 6 là nghiệm của phương trình
2) 2x + 3 < 6 - ( 3 - 4x )
<=> 2x + 3 < 6 - 3 + 4x
<=> 2x - 4x < 6 - 3 - 3
<=> -2x < 0
<=> x > 0
Vậy nghiệm của bất phương trình là x > 0


1/ y(y+4)=21 -> y^2 +4y -21=0 -> (y-3)(y+7)=0
VẬY y=3, -7.
2/???
3/(y-4)(y-1)=0 -> y=4, 1
THOI, MAY CAI CO BAN SGK CUNG HOI.DẸP, TỰ LÀM NỐT ĐI, DỄ MÀ.
XONG BẤM ĐÚNG CHO MÌNH
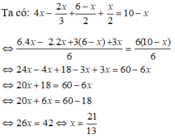
PT <=> (x2-4x+6)(x2-4x+10)=21
<=> x4-4x3+10x2-4x3+16x2-40x+6x2-24x+60-21=0
<=> x4-8x3+32x2-64x+39=0
<=> x4-x3-7x3+7x2+25x2-25x-39x+39=0
<=> x3(x-1)-7x2(x-1)+25x(x-1)-39(x-1)=0
<=> (x-1)(x3-7x2+25x-39)=0
<=> (x-1)(x3-3x2-4x2+12x+13x-39)=0
<=> (x-1)[x2(x-3)-4x(x-3)+13(x-3)]=0
<=> (x-1)(x-3)(x2-4x+13)=0
Nhận thấy: x2-4x+13 > 0 với mọi x
=> Phương trình có nghiệm là: \(\orbr{\begin{cases}x-1=0\\x-3=0\end{cases}}\)
<=> \(\orbr{\begin{cases}x_1=1\\x_2=3\end{cases}}\)
x²-4x+6=√(2x²-5x+3) - √(-3x²+9x-5).
Ta sẽ dùng đánh giá hai vế như sau :
VT = x²-4x+6 = x²-4x+4 + 2 = (x-2)² + 2 ≥ 2.
Dấu = xảy ra khi x = 2.
VP = √(2x²-5x+3) - √(-3x²+9x-5)
Áp dụng bất đẳng thức Bunhia Copxki ta có:
VP = √(2x²-5x+3) - √(-3x²+9x-5) ≤ √[(1² + 1²).(2x²-5x+3 - 3x²+9x-5)] = √[2.(-x²+4x-2)]
Mà: -x²+4x-2 = - ( x² - 4x+4) + 2 = -(x-2)² + 2 ≤ 2.
Do đó: VP ≤ √( 2.2) = √4 = 2.
Dấu = xảy ra khi x = 2.
Ta có: VT ≥ 2 ; VP ≤ 2 => VT = VP = 2 khi x = 2.
Vậy x = 2 là nghiệm của phương trình.