NT
1
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
19 tháng 8 2018
Vì |x-y| \(\ge\)0 với mọi x,y;|x+1|\(\ge\)0 vs mọi x
=>A\(\ge\)2016 vs mọi x,y
=> A đạt giá trị nhỏ nhất khi:\(\hept{\begin{cases}\left|x-y\right|=0\\\left|x+1\right|=0\end{cases}}\Leftrightarrow\hept{\begin{cases}x-y=0\\x+1=0\end{cases}}\Leftrightarrow\hept{\begin{cases}x=y\\x=-1\end{cases}}\)
vậy với x=y=-1 thì A đạt giá trị nhỏ nhất là 2016
k mik nha
bài này mik từng làm rồi
-----Chúc hok tốt---------
V
Với giá trị nào của x,y thì biểu thức : A = \(|x-y|+|x+1|+2016\)đạt giá trị nhỏ nhất. Tìm giá trị đó
3
27 tháng 3 2018
Ta có : \(\left|x+1\right|\ge0\forall x\)
Nên : |x + 1| nhỏ nhất bằng 0
<=> x + 1 = 0
=> x = -1
Lại có : \(\left|x-y\right|\ge0\forall x\)
Nên : |x - y| nhỏ nhất bằng 0
=> x - y = 0
mà x = -1
=> -1 - y = 0
=> y = -1
Vậy A = |x - y| + |x + 1| + 2016 nhwor nhất bằng 0 + 0 + 2016
=> A nhở nhất bằng 2016 khi x = y = -1
14 tháng 4 2020
Ta có: |x-y| >=0 với mọi x,y
|x+1| >=0 với mọi x,y
=> |x-y|+|x+1|+2016 >=2016 với mọi x,y
Dấu "=" xảy ra <=> \(\hept{\begin{cases}\left|x-y\right|=0\\\left|x+1\right|=0\end{cases}\Leftrightarrow\hept{\begin{cases}x-y=0\\x+1=0\end{cases}\Leftrightarrow}\hept{\begin{cases}x=y\\x=-1\end{cases}\Leftrightarrow}\hept{\begin{cases}y=-1\\x=-1\end{cases}}}\)
15 tháng 3 2017
Vì | x -3 | > hoặc = 0
Suy ra : |x-3|+50 >hoặc =50
Vì A nhỏ nhất suy ra | x-3 | +50 =50
Suy ra x-3 =0
Suy ra x=3
Vậy GTNN của A = 50 khi x=3
DD
Với giá trị nào của x; y thì biểu thức: A=lx- yl+l x+ 1l+ 2016 đạt giá trị nhỏ nhất. Tìm giá trị đó!
1
15 tháng 4 2018
Vì |x-y|\(\ge\)0 với mọi x,y
|x+1|\(\ge\)0 Với mọi x
\(\Rightarrow\)|x-y|+|x+1|\(\ge\)0 Với mọi x,y
\(\Rightarrow\)|x-y|+|x+1|+2016\(\ge\)2016 với mọi x,y
\(\Rightarrow\)A\(\ge\)2016 với mọi x,y
Dấu '=' xảy ra\(\Leftrightarrow\)\(\hept{\begin{cases}x-y=0\\x+1=0\end{cases}}\)\(\Leftrightarrow\)\(\hept{\begin{cases}x-y=0\\x=0-1=-1\end{cases}}\)\(\Leftrightarrow\)\(\hept{\begin{cases}-1-y=0\\x=-1\end{cases}}\)
\(\Leftrightarrow\)\(\hept{\begin{cases}y=-1-0=-1\\x=-1\end{cases}}\)
Vậy Min A=2016\(\Leftrightarrow\)x=-1,y=-1
NM
1 tháng 6 2016
Vì |x-2015| |\(\ge\) 0 và |y+2016| \(\ge\) 0
=>|x-2015| + |y+2016| - 20 \(\ge\) -20 (Vậy có nghĩa giá trị nhỏ nhất có thể có sẽ là -20)
Dấu "=" xảy ra khi:
|x-2015|=0 và |y+2016|=0
x-2015 =0 y+2016 =0
x =2015 y =-2016
Vậy GTNN của H=-20 khi x=2015 và y=-2016
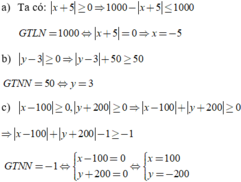
Vì \(\hept{\begin{cases}\left|x-y\right|\ge0\\\left|x-1\right|\ge0\end{cases}}\)\(\Rightarrow\left|x-y\right|+\left|x-1\right|\ge0\)
\(\Rightarrow\left|x-y\right|+\left|x-1\right|+2016\ge2016\).Nên GTNN của A là 2016 đạt được khi \(\hept{\begin{cases}\left|x-y\right|=0\\\left|x-1\right|=0\end{cases}}\)\(\Rightarrow\hept{\begin{cases}x-y=0\\x-1=0\end{cases}}\)\(\Rightarrow\hept{\begin{cases}x=y\\x=1\end{cases}}\)\(\Rightarrow x=y=1\)
Vậy với x=y=1 thì A có GTNN